The ratio of boys and girls in a college is 5:3. If 50 boys leave the college and 50 girls join the college, the ratio becomes 9:7. The number of boys in the college is:
300
400
500
600
The correct Answer is:C
To solve the problem step by step, we will follow the instructions given in the video transcript.
Step 1: Define the Initial Ratio
The initial ratio of boys to girls is given as 5:3. Let's denote:
- Number of boys = 5x
- Number of girls = 3x
Step 2: Apply the Changes
According to the problem, 50 boys leave the college, and 50 girls join. After these changes, the new number of boys and girls will be:
- New number of boys = 5x - 50
- New number of girls = 3x + 50
Step 3: Set Up the New Ratio
The new ratio of boys to girls is given as 9:7. We can set up the equation based on this ratio:
5x−503x+50=97
Step 4: Cross-Multiply to Solve for x
Cross-multiplying gives us:
7(5x−50)=9(3x+50)
Expanding both sides:
35x−350=27x+450
Step 5: Rearrange the Equation
Now, we will rearrange the equation to isolate x:
35x−27x=450+350
8x=800
Step 6: Solve for x
Now, divide both sides by 8:
x=100
Step 7: Calculate the Number of Boys
Now that we have the value of x, we can find the number of boys:
Number of boys=5x=5×100=500
Final Answer
The number of boys in the college is 500.
---
The initial ratio of boys to girls is given as 5:3. Let's denote:
- Number of boys = 5x
- Number of girls = 3x
According to the problem, 50 boys leave the college, and 50 girls join. After these changes, the new number of boys and girls will be:
- New number of boys = 5x - 50
- New number of girls = 3x + 50
The new ratio of boys to girls is given as 9:7. We can set up the equation based on this ratio:
5x−503x+50=97
Cross-multiplying gives us:
7(5x−50)=9(3x+50)
35x−350=27x+450
Now, we will rearrange the equation to isolate x:
35x−27x=450+350
8x=800
Now, divide both sides by 8:
x=100
Now that we have the value of x, we can find the number of boys:
Number of boys=5x=5×100=500
The number of boys in the college is 500.
Topper's Solved these Questions
- RATIO AND PROPORTION BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TYPE-IV
30 Videos
- RATIO AND PROPORTION BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TYPE-V
64 Videos
- RATIO AND PROPORTION BOOK - KIRAN PUBLICATIONCHAPTER - RATIO AND PROPORTION EXERCISE - TYPE-II
49 Videos
- PROFIT AND LOSS BOOK - KIRAN PUBLICATIONCHAPTER - PROFIT AND LOSS EXERCISE - TEST YOURSELF
23 Videos
- SEQUENCE AND SERIES BOOK - KIRAN PUBLICATIONCHAPTER - SEQUENCE AND SERIES EXERCISE - TEST YOURSELF
20 Videos
Similar Questions
Knowledge Check
The ratio of boys and girls in a college is 5:3. If 50 boys leave the college and 50 girls join the college, the ratio becomes 9:7. The number of boys in the college is:
A300B400C500D600What is the ratio of boys and girls in the colleges A and B taken together
A5:6B6:7C1:1D7:8The table below shows the percentage of students and the ratio of boys and girls in different colleges. Total students = 1800
नीचे दी गयी तालिका छात्रों का प्रतिशत तथा अलग-अलग कॉलेजों में लड़कों एवं लड़कियों का अनुपात दर्शाती है | कुल छात्र - 1800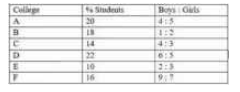 What is the ratio of boys and girls in the colleges A and B taken together?
What is the ratio of boys and girls in the colleges A and B taken together?
कॉलेज A और B को मिलाकर लड़कों तथा लड़कियों का अनुपात क्या है?A45:71B37:52C43:67D67:104
The ratio of boys and girls in a group is 7:6. If 4 more boys join the group and 3 girls leave the group, then the ratio of boys to girls becomes 4:3. What is the total number of boys and girls initially in the group?
एक समूह में लड़कों तथा लड़कियों का अनुपात 7 : 6 है | यदि 4 और लड़के समूह में आ जाते हैं तथा 3 लड़कियाँ समूह से चली जाती हैं, तो लड़कों तथा लड़कियों का अनुपात 4: 3 हो जाता है | आरंभ में इस समूह में लड़कों तथा लड़कियों की कुल संख्या कितनी थी ?
View SolutionIn a school , the number of boys and girls are in Ratio 5:3 Some new boys and girls took admission in the school in the ratio 5:7 Now , the total number of students in the school are 1200 and the ratio of total number of boys and girls become 7:5 Find the number of students initially .
View SolutionThe ratio of the number of boys and girls in a college in 3:2. If 20% of boys and 25% of girls are adults, the percentage of those students who are not adults, is
View SolutionThe ratio of number of boys to that of girls in a group becomes 2 : 1 when 15 girls leave. But, afterward5 when 45 boys also leave, the ratio becomes I Originally the number of girls in the group was 20 b. 30 c. 40 d. 50
View SolutionThe given pie chart shows the percentage of students enerolled into the colleges A,B,C,D,E and F in a city , and the table shows the ratio of boys to girls in the college .
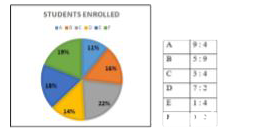
Based on this information , if the total number of students is 9800, then the number of girls in the college B is :
View Solution
