A light rigid rod of length l is constrained to move in a vertical plane, so that its ends are along the x and y axes respectively. Find the instantaneous axis of rotation of the rod when it makes an angle θ with horizontal.
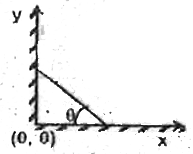
When the rod inclines at an angle θ with vertical, let the contact forces at A & B are NA&NB respectively as shown in the figure.
⇒ Equation of motion of c.m. of the rod can be given by,
Fx=max ⇒ NA=max ...(i)
& Fy=may NB−mg=may ...(ii)
where ax&ay are the horizontal & vertical acceleration of the c.m. of the rod respectively. The moment of NA&NB anticlockwise about G is given by [G is COM of rod]
τ(G)=−NA(l/2)cosθ+NB(l/2)sinθ
⇒ τG=IGα,where IG = M.I. of the rod about G.
IG=ml2/12andα = angular accleration of the rod =d2θdt2
⇒ (−NAcosθ+NBsinθ)l/2=ml2α/12 ...(iii)

Now we have three equations and four unknowns NA,NB,ax&ay. Therefore we need another two equations by using kinematics, x = (l/2) sin θ & y = (l/2) cos θ, by differentiating x and y, we obtain
ax=d2xdt2=l2(αsinθ−ω2cosθ) ...(iv)
ay=d2ydt2=−l2(αsinθ+ω2cosθ) ...(v)
Using (i) & (iv), find NA & using (ii) & (v) find NB, then put NA&NB in equation (iii) to obtain α=(3g/2l)sinθ
Putting α=ωdωdθ we obtain,
ωdω=3g2lsinθdθ⇒∫ω0ωdω=3g2l∫θ0sinθ ⇒ω=√3g(1−cosθ)l
Alter
I=ml212+ml24 (∵y=l/2)
I=ml23
By law of energy conservation,
12Iω2=mgl2(1−cosθ)⇒ω=√3gl(1−cosθ)
α=dωdt=3g2lsinθ

Similar Questions
A rod of length l is given two velocities v1 and v2 in opposite directions at its two ends at right angles to the length. The distance of the instantaneous axis of rotation from v1 is
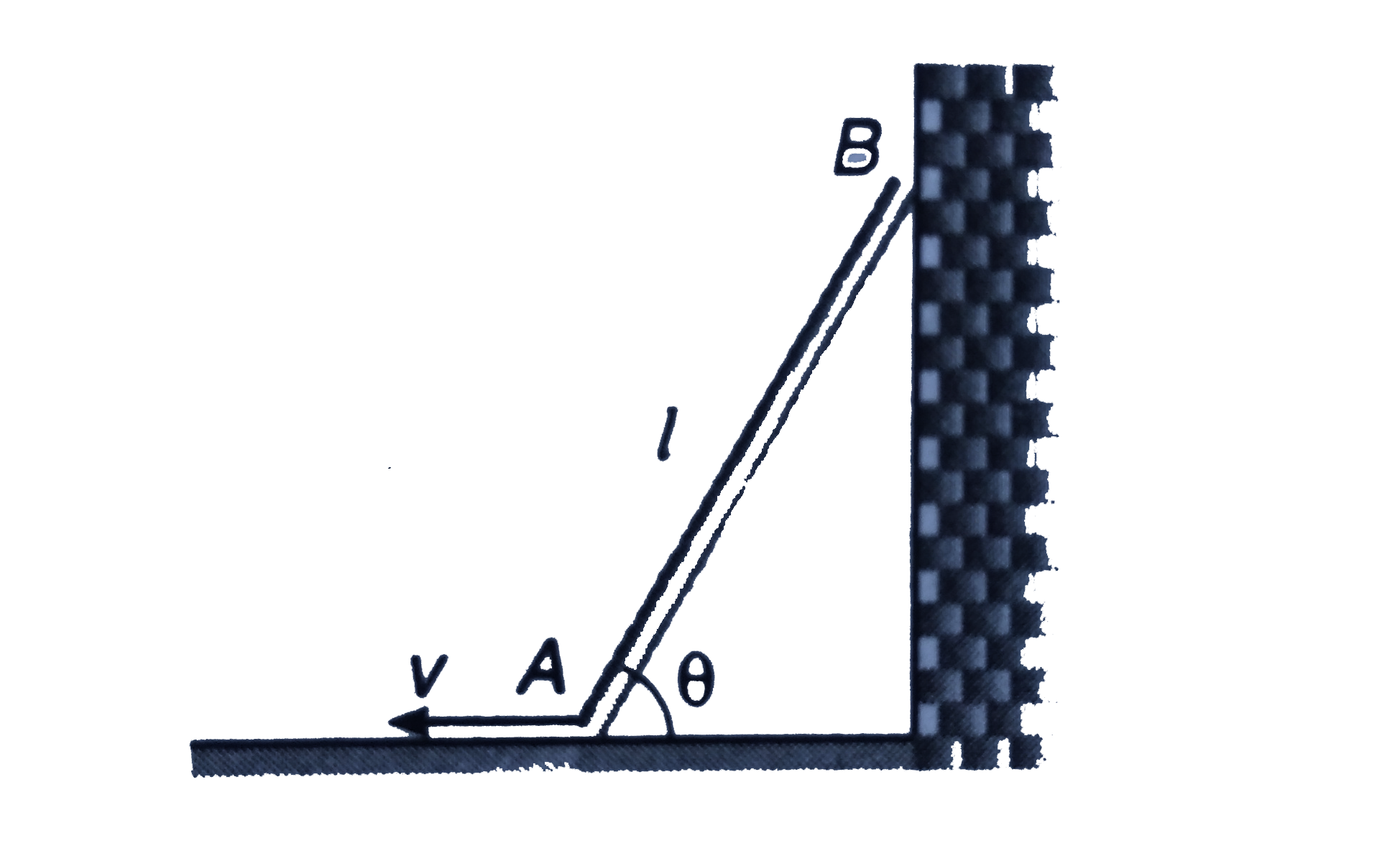
View SolutionFigure shows a rod of length l resting on a wall and the floor. Its lower end A is pulled towards left with a constant velocity v. Find the velocity of the other end B downward when the rod makes an angle θ with the horizontal.
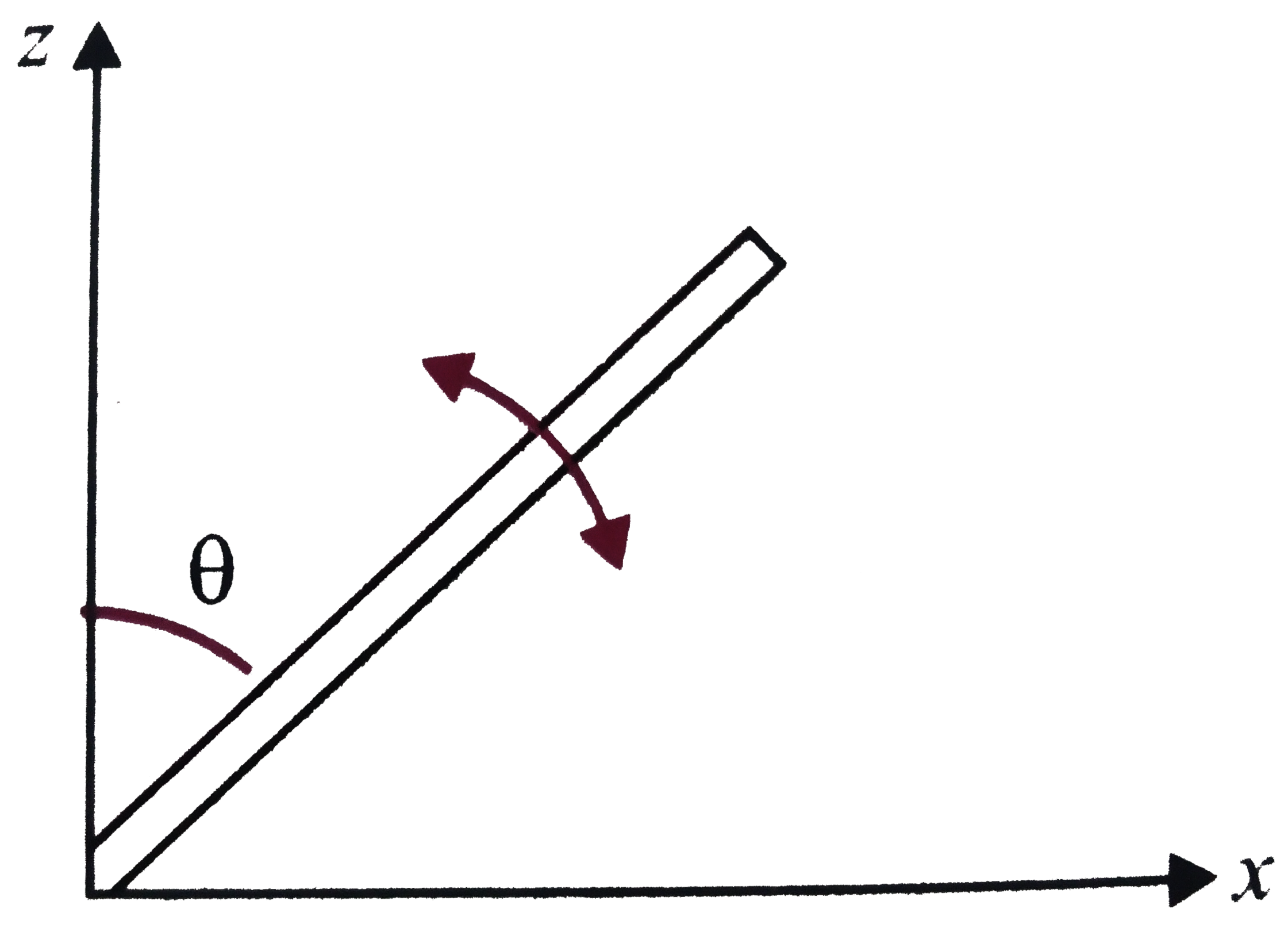
View SolutionA rod of length l is moving in a vertical plane (x−y) when the lowest point A of the rod is moved with a velocity v. find the a angular velocity of the rod and b velocity of the end B.

View SolutionA slender uniform rod of mass M and length l is pivoted at one end so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
View SolutionA uniform thin rod of mass m and length l is standing on a smooth horizontal suface. A slight disturbance causes the lower end to slip on the smooth surface and the rod starts falling. Find the velocity of centre of mass of the rod at the instant when it makes an angle θ with horizontal.
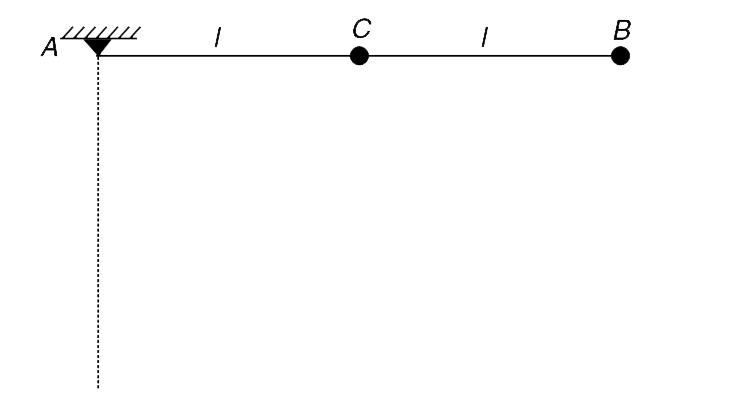
View SolutionAB is a mass less rigid rod of length 2l. It is free to rotate in vertical plane about a horizontal axis passing through its end A. Equal point masses (m each) are stuck at the centre C and end Bof the rod. The rod is released from horizontal position. Write the tension in the rod when it becomes vertical.
View Solution
A uniform rod of length L is free to rotate in a vertical plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given as
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle α with the horizontal will be proportional to : (see figure)
View SolutionA uniform rod of mass m and length l0 is rotating with a constant angular speed ω about a vertical axis passing through its point of suspension. Find the moment of inertia of the rod about the axis of rotation if it make an angle θ to the vertical (axis of rotation).
View SolutionA rigid rod of mass m & length l is pivoted at one of its ends. If it is released from its horizontal position, find the speed of the centre of mass of the rod when it becomes vertical.
View Solution
