Question

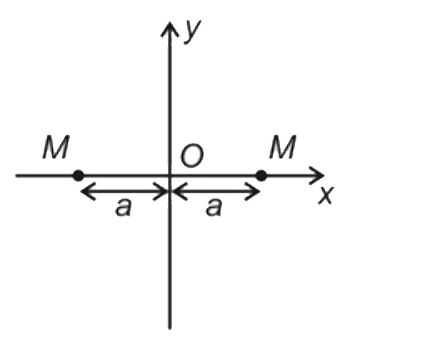
There are two fixed heavy masses of magnitude $M$ of high density at a distance $2d$ apart. On the axis, a small mass $m$ moves in a circle of radius $R$ in the $y-z$ plane between the heavy masses. Find the velocity of the small particle.

Explanation

To find the velocity of the small mass $$m$$ moving in a circular path between two fixed heavy masses $$M$$ at a distance $$2d$$ apart, we can follow these steps:

Step 1: Understanding the Setup
We have two heavy masses \( M \) located at points \( (-d, 0, 0) \) and \( (d, 0, 0) \). The small mass \( m \) is moving in a circular path of radius \( R \) in the \( y-z \) plane. The gravitational force between the small mass \( m \) and each of the heavy masses \( M \) will provide the necessary centripetal force for circular motion.

Step 2: Calculate the Gravitational Force
The gravitational force \( F \) between each mass \( M \) and the small mass \( m \) can be given by Newton's law of gravitation:
\( F = \frac{G M m}{r^2} \)
where \( r \) is the distance from the mass \( m \) to the mass \( M \). For the small mass \( m \), the distance to each mass \( M \) can be calculated using the Pythagorean theorem:
\( r = \sqrt{d^2 + R^2} \)
Thus, the gravitational force exerted by one mass \( M \) on \( m \) becomes:
\( F = \frac{G M m}{d^2 + R^2} \)

Step 3: Net Gravitational Force on the Small Mass
Since there are two masses \( M \) exerting gravitational force on \( m \), the net force acting on \( m \) in the downward direction (towards the center of the circular path) is:
\( F_{\text{net}} = 2F \cos(\theta) \)
where \( \theta \) is the angle between the line connecting \( m \) to \( M \) and the vertical axis. By symmetry, \( \cos(\theta) \) can be expressed as:
\( \cos(\theta) = \frac{R}{\sqrt{d^2 + R^2}} \)
Thus, the net gravitational force becomes:
\( F_{\text{net}} = 2 \left(\frac{G M m}{d^2 + R^2}\right) \frac{R}{\sqrt{d^2 + R^2}} = \frac{2 G M m R}{(d^2 + R^2)^{3/2}} \)

Step 4: Centripetal Force Requirement
For circular motion, the centripetal force required is given by:
\( F_{\text{centripetal}} = \frac{m v^2}{R} \)
where \( v \) is the velocity of the small mass \( m \).

Step 5: Setting Forces Equal
Since the net gravitational force provides the centripetal force, we can set them equal:
\( \frac{m v^2}{R} = \frac{2 G M m R}{(d^2 + R^2)^{3/2}} \)
Cancelling \( m \) from both sides (assuming \( m \neq 0 \)):
\( \frac{v^2}{R} = \frac{2 G M R}{(d^2 + R^2)^{3/2}} \)

Step 6: Solve for Velocity
Multiplying both sides by \( R \):
\( v^2 = \frac{2 G M R^2}{(d^2 + R^2)^{3/2}} \)
Taking the square root gives:
\( v = \sqrt{\frac{2 G M R^2}{(d^2 + R^2)^{3/2}}} \)

Final Expression
Thus, the velocity of the small mass \( m \) is:
\( v = R \sqrt{\frac{2 G M}{(d^2 + R^2)^{3/2}}} \)