A water tank is 20 m deep . If the water barometer reads 10 m at the surface , then what is the pressure at the bottom of the tank in atmosphere ?
To find the pressure at the bottom of the tank, we can follow these steps:
Step 1: Understand the given information
We know that the water tank is 20 meters deep. The barometer reads 10 meters at the surface of the water. This indicates the atmospheric pressure acting on the surface of the water.
Step 2: Identify the pressures
Let:
- P0 be the atmospheric pressure at the surface (which corresponds to the 10 m barometer reading).
- PB be the pressure at the bottom of the tank (point B).
- PA be the pressure at the surface of the water (point A).
From the barometer reading, we know:
P0=ρg×10 m
where:
- ρ is the density of water (approximately 1000kg/m3),
- g is the acceleration due to gravity (approximately 10m/s2).
Step 3: Calculate the atmospheric pressure
Using the values:
P0=1000kg/m3×10m/s2×10m=100000Pa=105Pa
Step 4: Calculate the pressure at the bottom of the tank
The pressure at the bottom of the tank can be calculated using the hydrostatic pressure formula:
PB=P0+ρgh
where h=20m.
Substituting the values:
PB=P0+ρg×20
PB=105Pa+(1000kg/m3)(10m/s2)(20m)
PB=105Pa+200000Pa
PB=300000Pa
Step 5: Convert pressure to atmosphere
To convert the pressure from Pascals to atmospheres, we use the conversion factor:
1atm=1.013×105Pa
Thus,
PB=300000Pa1.013×105Pa/atm≈2.96atm
Final Answer
The pressure at the bottom of the tank is approximately 2.96 atm.
---
We know that the water tank is 20 meters deep. The barometer reads 10 meters at the surface of the water. This indicates the atmospheric pressure acting on the surface of the water.
Let:
- P0 be the atmospheric pressure at the surface (which corresponds to the 10 m barometer reading).
- PB be the pressure at the bottom of the tank (point B).
- PA be the pressure at the surface of the water (point A).
P0=ρg×10 m
where:
- ρ is the density of water (approximately 1000kg/m3),
- g is the acceleration due to gravity (approximately 10m/s2).
Using the values:
P0=1000kg/m3×10m/s2×10m=100000Pa=105Pa
The pressure at the bottom of the tank can be calculated using the hydrostatic pressure formula:
PB=P0+ρgh
where h=20m.
PB=P0+ρg×20
PB=105Pa+(1000kg/m3)(10m/s2)(20m)
PB=105Pa+200000Pa
PB=300000Pa
To convert the pressure from Pascals to atmospheres, we use the conversion factor:
1atm=1.013×105Pa
PB=300000Pa1.013×105Pa/atm≈2.96atm
The pressure at the bottom of the tank is approximately 2.96 atm.
Similar Questions
The pressure at the bottom of a tank of liquid is not proprtional to
View SolutionP is the atmospheric pressure and the pressure at the bottom of a tank of water is 3P. If the water is drawn out to lower the level of water by one fifth, then the pressure at the bottom of the tank will be
View SolutionThe pressure at the bottom of an open tank of water is 3p where p is the atmospheric pressure. If the water is drawn out till the level of water remains one fifth, the pressure at the bottom of the tank will now be
View SolutionA hole is made at the bottom of the tank filled with water (density =1000kgm−3). If the total pressure at the bottom of the tank is three atmospheres (1 atmosphere =105Nm−2), then the velocity of efflux is nearest to
View SolutionThe pressure at the bottom of a tank of water is 2P, where P is the atmospheric pressure.If the water is drawn out till the level of water is lowered by one half of initial,the pressure at the bottom of the tank will now be
View SolutionThe pressure at the bottom of a water tank is 4 P, where P is .atmospheric pressure. If water is drawn out till the water level decreases by —35th, then pressure at the bottom of the tank is
View SolutionA tank 5 m high is half filled with water and then is filled to top with oil of density 0.85g/cm3 The pressure at the bottom of the tank, due to these liquids is
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
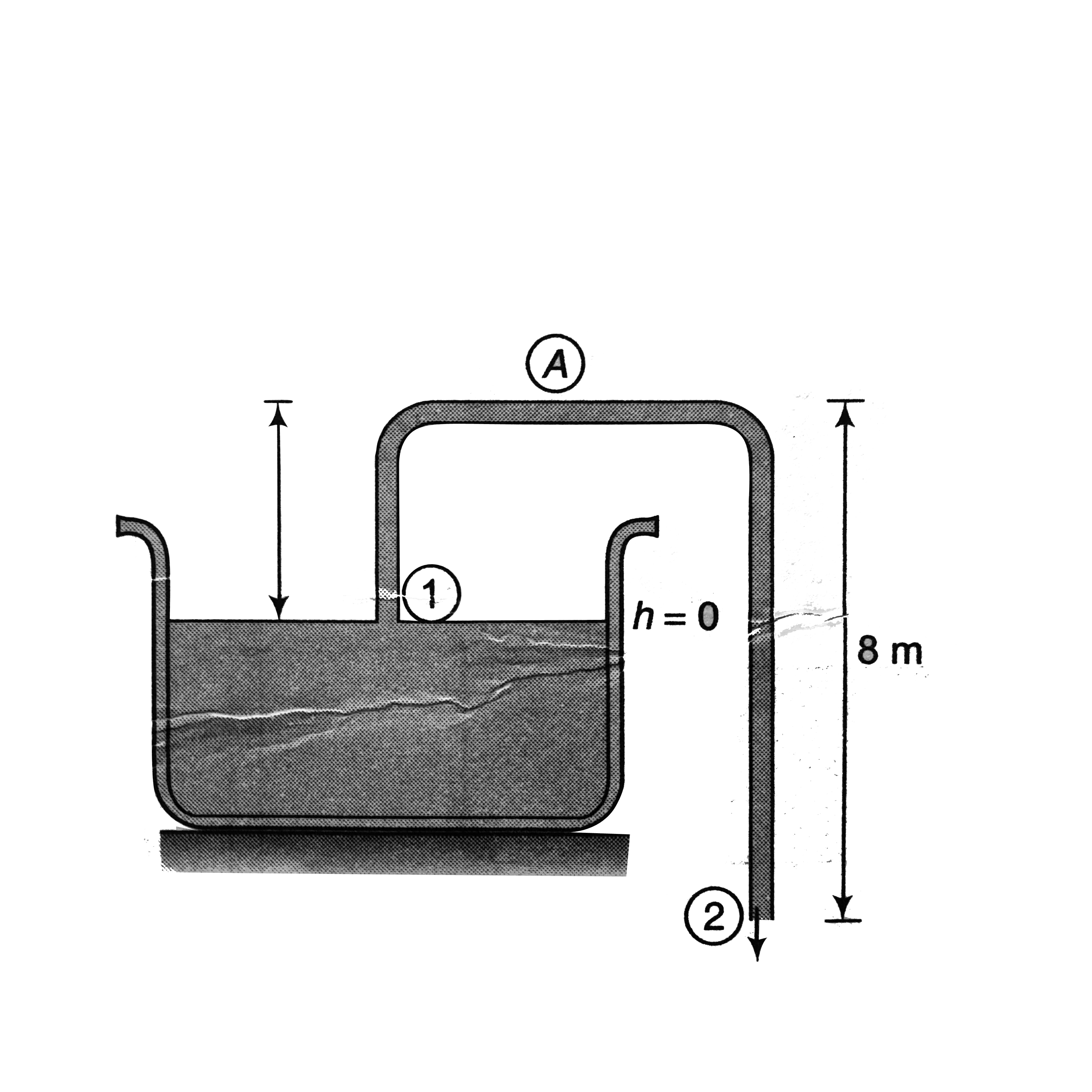 .
.
View SolutionAn air bubble rises from the bottom of a lake of depth 10.34m to its surface. Compare the pressure on bubble at the bottom to that on surface. (Atmospheric pressure =0.76mofHg, density of Hg=13.6×103Kgm−3 and density of water =103kgm−3).
View SolutionIf the gauge pressure, at the bottom of a water tank is 2.7 kPa, what is the height of the water [Take g = 10 m s−2]
Hint : Gauge Pressure = absolute pressure - atmospheric pressure = pgh
View Solution
