A tank 5 m high is half filled with water and then is filled to top with oil of density 0.85g/cm3 The pressure at the bottom of the tank, due to these liquids is
1.85g(wt)cm2
89.25g(wt)cm2
462.5g(wt)cm2
500g(wt)cm2
The correct Answer is:C
Step by step video & image solution for A tank 5 m high is half filled with water and then is filled to top with oil of density 0.85 g//cm^3 The pressure at the bottom of the tank, due to these liquids is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Surface Tension)26videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Thermal Physics) (Temperature scales & thermal expansion)13videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Fluid Dynamics + Viscosity)18videos
RACE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
Similar Questions
A uniformly tapering vessel is filled with a liquid of density 900km/m3. The force that acts on the base of the vessel due to the liquid is (g=10ms−2)
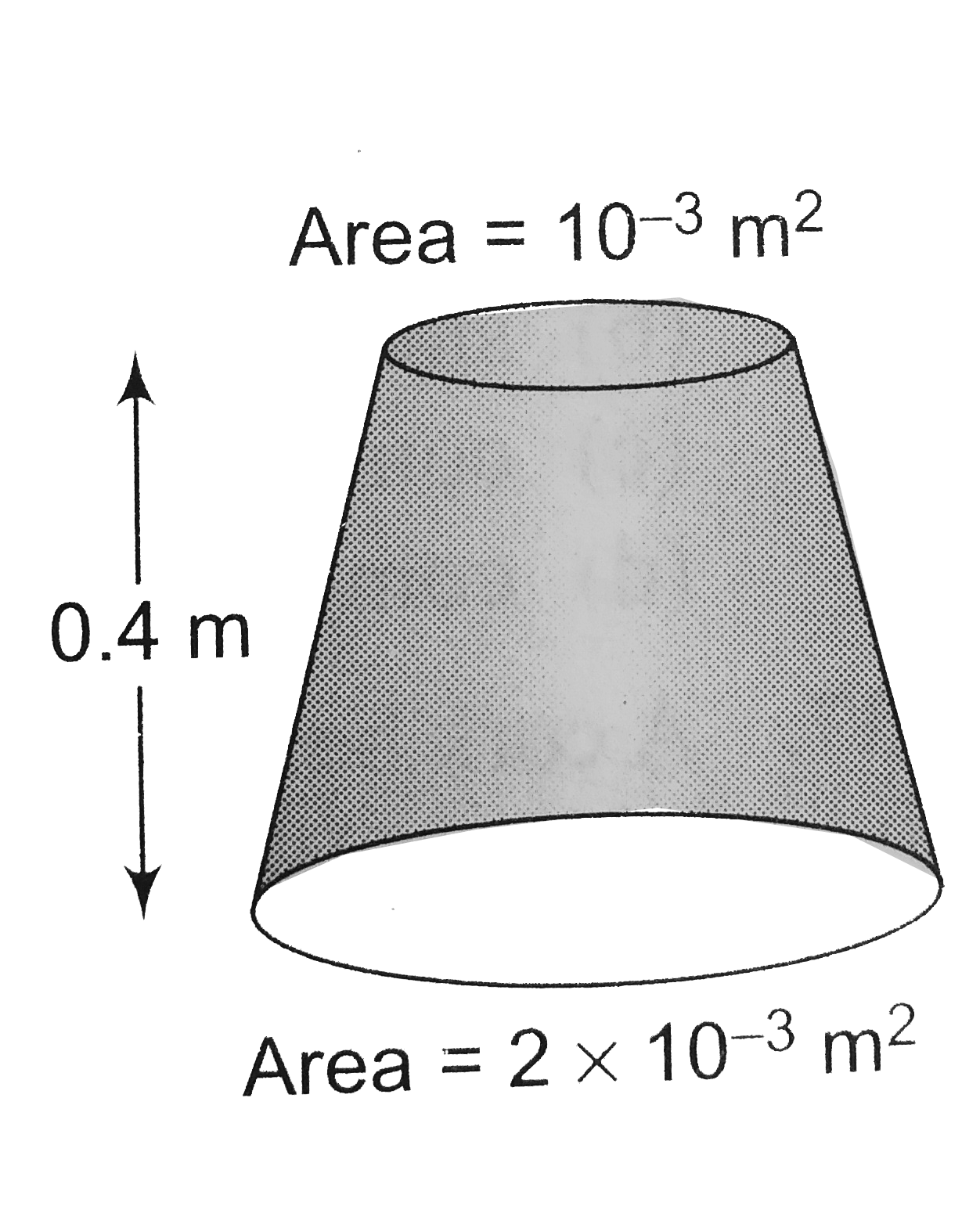 View Solution
View SolutionA hole is made at the bottom of the tank filled with water (density =1000kgm−3). If the total pressure at the bottom of the tank is three atmospheres (1 atmosphere =105Nm−2), then the velocity of efflux is nearest to
View SolutionA water tank is 20 m deep . If the water barometer reads 10 m at the surface , then what is the pressure at the bottom of the tank in atmosphere ?
View SolutionP is the atmospheric pressure and the pressure at the bottom of a tank of water is 3P. If the water is drawn out to lower the level of water by one fifth, then the pressure at the bottom of the tank will be
View SolutionA tank of height H and base area A is half filled with water and there is a small orifice at the bottom and there is a heavy solid cylinder having base area A3 and height of the cylinder is same as that of the tak. The water is flowing out of the orifice. Here cylinder is put into the tank to increase the speed of water flowing out.
After long time, when the height of water inside the tank again becomes equal to H2, the solid cylinder is taken out. then the velocity of liquid flowing out of the orifice (just after removing the cylinder) will beView SolutionA tank is filled with water of density 103kg/m3 and oil of density 0.9×103kg/m3.The height of water layer is 1m and that of the oil layer is 4M. The velocity of efflux front an opening in the bottom of the tank is
View SolutionThe pressure at the bottom of a water tank is 4 P, where P is .atmospheric pressure. If water is drawn out till the water level decreases by —35th, then pressure at the bottom of the tank is
View SolutionA tank of large base area is filled with water up to a height of 5m. A hole of 2cm2 cross section in the bottom allows the water to drain out in continuous streams. For this situation, mark out the correct statement(s) (take ρwater=1000kg/m3,g=10ms−12)
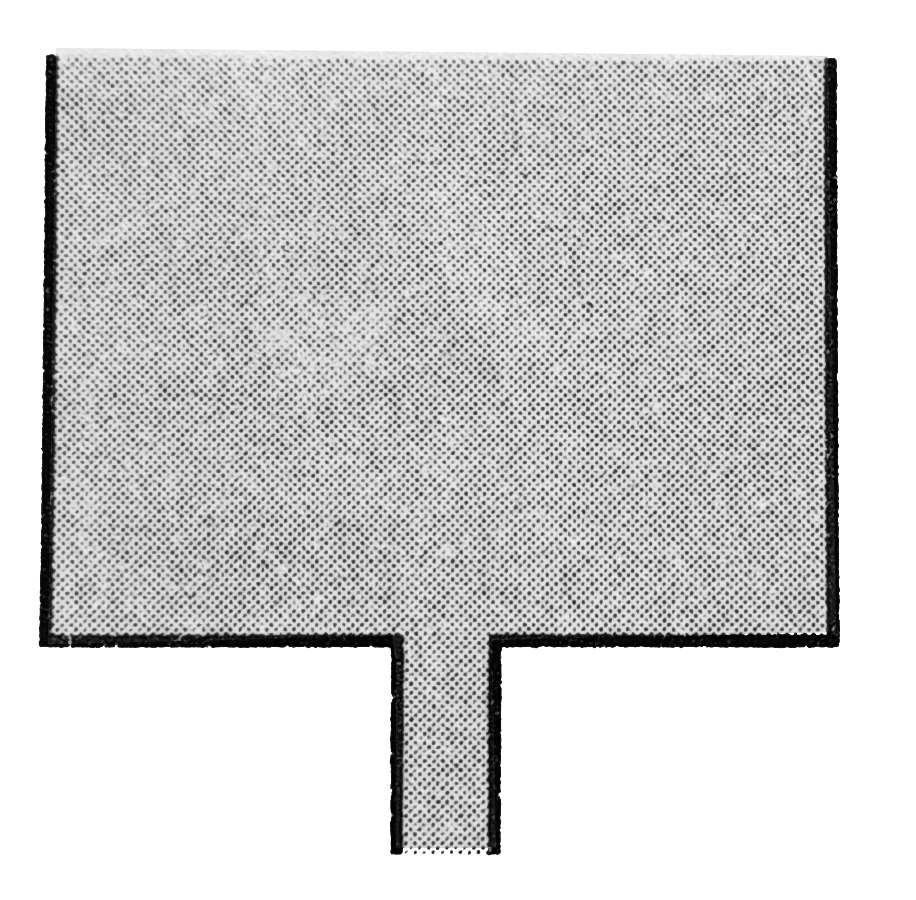 View Solution
View SolutionThe flat bottom of cylinder tank is silvered and water (μ=43) is filled in the tank upto a height h. A small bird is hovering at a height 3h from the bottom of the tank. When a small hole is opened near the bottom of the tank, the water level falls at the rate of 1 cm/s. The bird will perceive that his velocity of image is 1/x cm/sec (in downward directions) where x is
View SolutionIf liquid of density ρ is filled in an open cylindrical container to height h, then pressure at bottom
View Solution


