Taking X - axis along horizontal and Y - axis along vertical, with what minimum speed must a particle be projected from the origin so that it is able to pass through a given point (30m, 40 m)? Take g=10ms−2
26 m/s
30 m/s
20 m/s
10 m/s
The correct Answer is:b
40=30 tan α−(g(30))22u2(1+tan2α)
For real value of α
(6u2)2≥3600(900+8u2)
⇒u4−800u2≥900,00
(u2−400)2≥25,000
⇒u2−400≥5,00
u2≥900
⇒u≥30m/s
Similar Questions
With what minimum speed must a particle be projected from origin so that it is able to pass through a given point (30m,40m) ? Take g=10m/s2
View SolutionWith what minimum speed must a particle be projected from origin so that it is able to pass through a given point (30m,40m) ? Take g=10m/s2
View SolutionA body is projected from the ground with some speed at some angle with the horizontal. Taking the horizontal and vertical direction to be x and y axis respectively and the point of projection as origin, calculate the minimum speed (in ms−1) of projection so that it can pass through a point whose x and y coordinates are 30 m and 40 m respectively? Take g=10ms−2
View SolutionThe equation of the parabola whose vertex is origin axis along x-axis and which passes through the point (-2,4) is
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View SolutionA cart is moving horizontally along a straight line with constant speed 30ms−1. A particle is to be fired vertically upwards from the moving cart in such a way that it returns to the cart at the same point from where it was projected after the cart has moved 80m. At what speed (relative to the cart) must the projectile be fired? (Take g=10ms−2)
View SolutionA cart is moving horizontally along a straight line with constant speed 30 m/s. A projectile is to be fired from the moving cart in such a way that it will return to the cart after the cart has moved 120m. At what speed (relative to the cart, in m/s) must the projectile be fired ? (Take g=10m/s2)
View SolutionTrack OABCD (as shown in figure ) is smooth. What minimum speed has to be given to a particle lying at point A, so that it can reach point C? (take g=10m/s2)
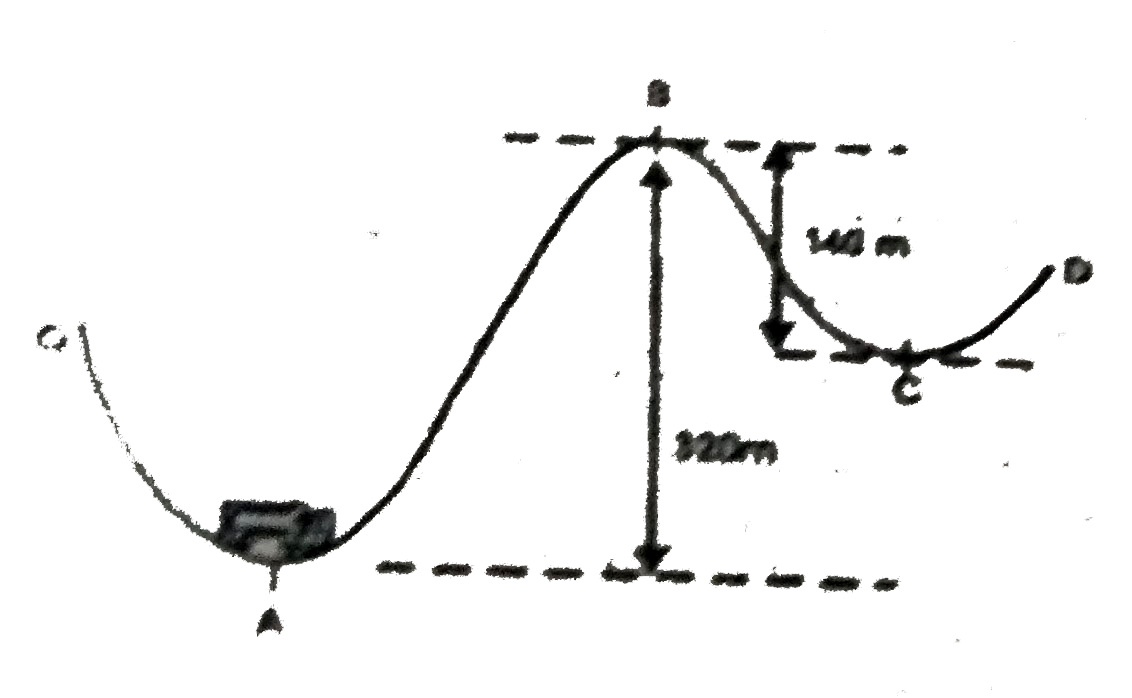
View SolutionTaking horizontal ground as xz- plane and y-axis vertically upwards. A particle is projected from the horizontal ground from a point whose position vector is ˆi+ˆk meter with initial velocity 2i +3j+k from the origin when it hits ground is given by 15[11ˆi+nˆk], find n. (Take g=10m/s2 in negative y - direction)
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View Solution

