A certain ideal spring stretches 20cm when m1=40gm mass is hung from it. If a mass of m2=20532gm gm is hung instead of 40gm at its end pulled out 20cm from equilibirum and released at t=0 sec. Choose the correct option (s) (g=10m/s2)
a) The magnitude of acceleration of the m2 mass when it is 2.5cmfrom the equilibrium position is 6445m/s2
b) The magnitude of acceleration of the m2 mass when it si 2.5cm from the equilibrium position is 3245m/s2
c) At t=π8 sec m2 mass is 10cm from equilibrium position
d) At t=π2 sec m2 is 10cm from equilibrium position
The correct Answer is:B, C, D
To solve the problem step by step, we will follow these steps:
Step 1: Calculate the Spring Constant (k)
Given that the spring stretches 20 cm (0.2 m) when a mass m1=40g=0.04kg is hung from it, we can use Hooke's Law:
F=kx
Where:
- F=mg=0.04kg×10m/s2=0.4N
- x=0.2m
Rearranging gives:
k=Fx=0.4N0.2m=2N/m
Step 2: Calculate the Angular Frequency (ω)
Now we need to find the angular frequency for the new mass m2=20532g=20532000kg.
Convert m2 to kg:
m2=20532g=20532×1000kg=20532000kg
Now, we can find ω:
ω=√km=
⎷220532000
Calculating ω:
ω=√2×32000205=√64000205≈16rad/s
Step 3: Write the Equation of Motion
The equation of motion for simple harmonic motion (SHM) is given by:
x(t)=x0cos(ωt)
Where x0=0.2m and ω≈16rad/s:
x(t)=0.2cos(16t)
Step 4: Calculate the Acceleration
The acceleration a(t) can be found using:
a(t)=−ω2x0cos(ωt)
Substituting the values:
a(t)=−162×0.2cos(16t)=−256×0.2cos(16t)=−51.2cos(16t)m/s2
Step 5: Find Specific Values
To find the acceleration when x=2.5cm=0.025m:
Set x(t)=0.025:
0.025=0.2cos(16t)
Solving for cos(16t):
cos(16t)=0.0250.2=0.125
Now, we can find 16t:
16t=cos−1(0.125)
Step 6: Final Calculation of Acceleration
Substituting back into the acceleration equation:
a(t)=−51.2cos(16t)
Using cos(16t)=0.125:
a(t)=−51.2×0.125=−6.4m/s2
Summary of Results
- Spring constant k=2N/m
- Angular frequency ω≈16rad/s
- Acceleration when x=2.5cm is approximately −6.4m/s2
- F=mg=0.04kg×10m/s2=0.4N
- x=0.2m
⎷220532000
- Angular frequency ω≈16rad/s
- Acceleration when x=2.5cm is approximately −6.4m/s2
Similar Questions
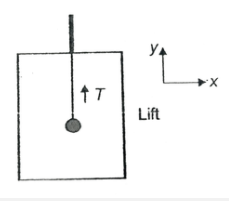
A pendulum bob of mass 50 gm is hanging from the ceiling of a lift by a light , inextensible string. Choose the correct options ( g = 9.8 m/s2)
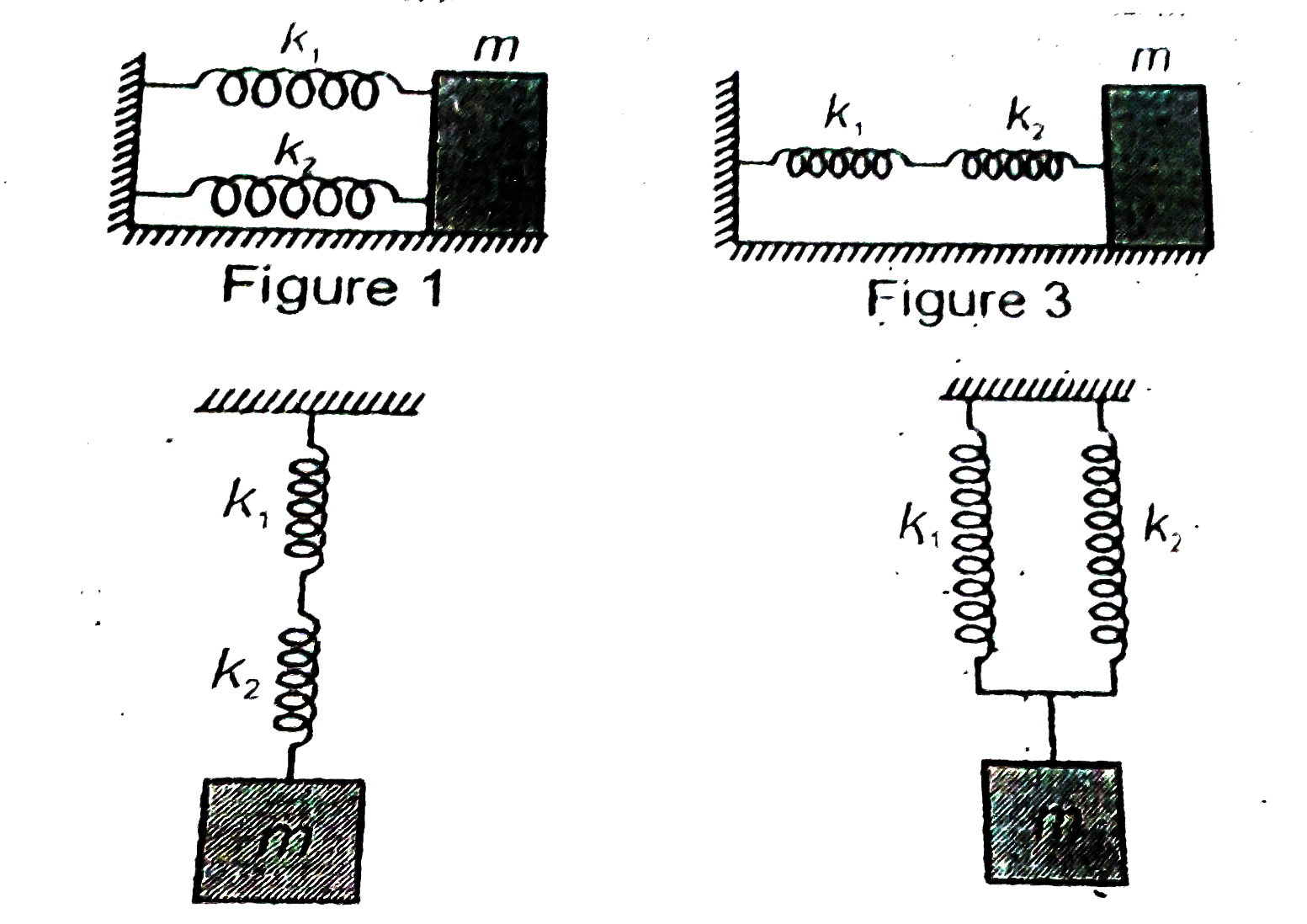
View SolutionFigure-1 to Figure -4 shows four different spring arrangements . Mass m in each arrangement is displacement from its equilibrium position and released Neglec mass of the springs. Choose the correct statement (s)
View SolutionA metal rod of length 50cm having mass 2kg is supported on two edges placed 10cm from each end. A 3kg load is suspended at 20cm from one end. Find the reactions at the edges (take g=10m/s2)
View SolutionA metal rod of length 50cm having mass 2kg is supported on two edges placed 10cm from each end. A 3kg load is suspended at 20cm from one end. Find the reactions at the edges (take g=10m/s2)
View SolutionA mass of 10 g moving horizontally with a velocity of 100cm−1 strikes a pendulum bob of mass 10g. Length of string is 50 cm. The two masses stick together. The maximum height reached by the stystm now is (Take, g=10ms−2
View SolutionA mass of 10 gm moving with a velocity of 100 cm / s strikes a pendulum bob of mass 10 gm . The two masses stick together. The maximum height reached by the system now is (g=10m/s2)
View SolutionThe reading of a spring balance when a mass is weighed on it in air is 40 gm. When the mass is immersed in water, its reading is 20 gm. The specific gravity of the mass is
View SolutionAn ideal spring with spring constant k is hung from the ceiling and a block of mass M is attached to its lower end. The mass is released with the spring initially unstretched. Then the maximum extension in the spring is
View SolutionAn ideal spring with spring constant k is hung from the ceiling and a block of mass M is attached to its lower end. The mass is released with the spring initially unstretched. Then the maximum extension in the spring is
View SolutionA ball of mas 2gm is thrown vertically upwards with a speed of 30m/s from a tower of height 35m. (Given g=10m/s2)
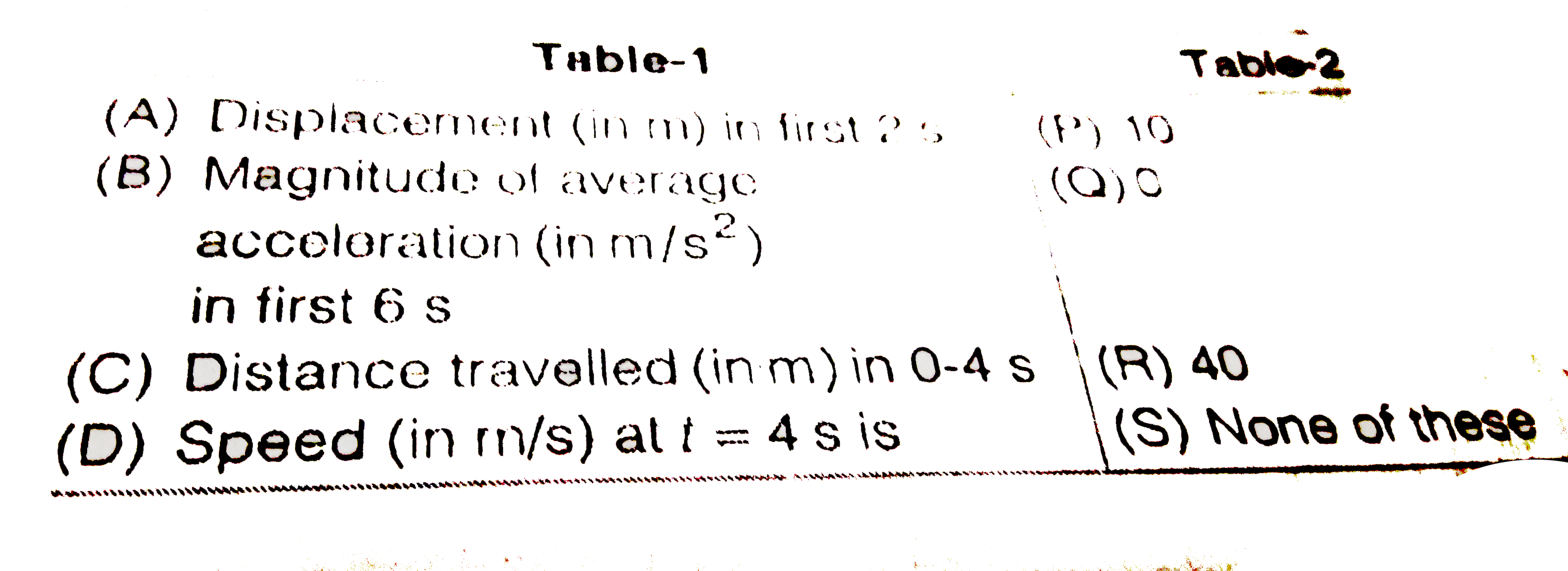
View Solution
