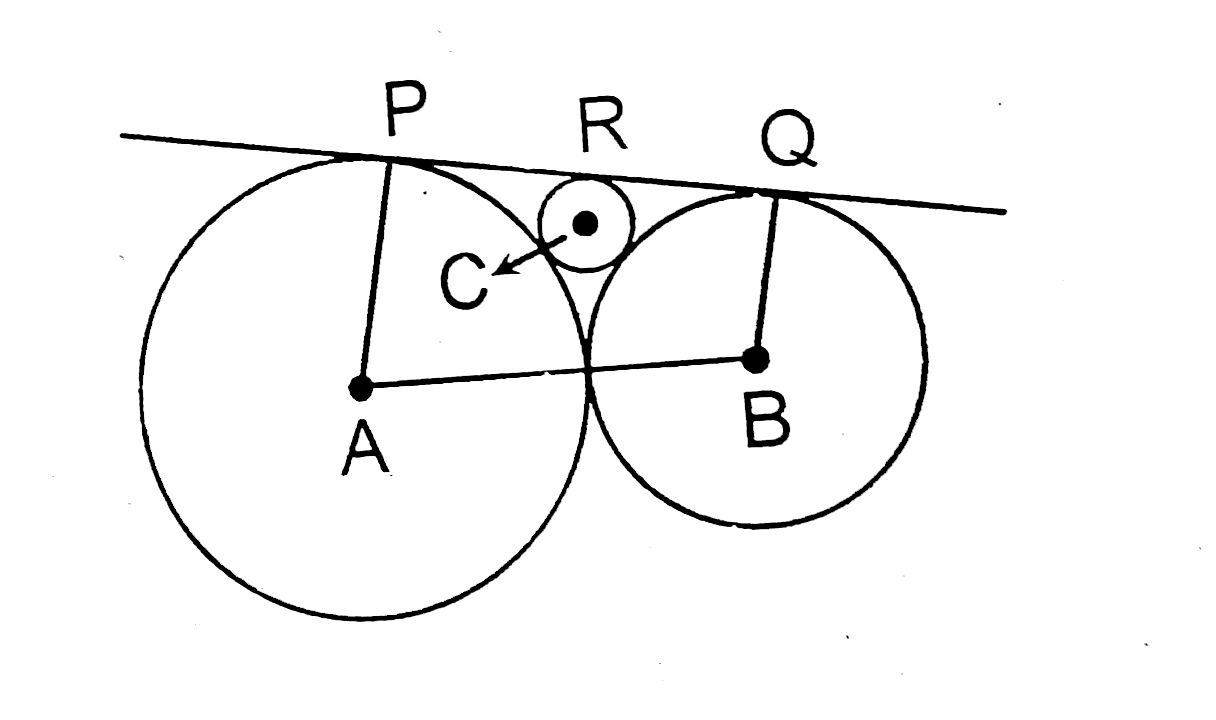
Two circles with radii a and b(agtb) touch each other externally . Let c be the radii of a circle both touches these two circles as well as a direct common tangent to these two circles. Then

A

$\frac{1}{\sqrt{r_{3}}} = \frac{1}{\sqrt{r_{1}}} + \frac{1}{\sqrt{r_{2}}}$

B

$\frac{1}{\sqrt{r_{3}}} = ∣ ∣ ∣ \frac{1}{r_{1}} - \frac{1}{{\sqrt{r}}_{2}} ∣ ∣ ∣$

C

$\sqrt{r_{3}} - \sqrt{r_{1}} + \sqrt{r_{2}}$

D

$\sqrt{r_{3}} = ∣ ∣ \sqrt{r_{1}} - \sqrt{r_{2}} ∣ ∣$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Using length of direct common tangent
$\begin{matrix} P Q^{2} & = & 4 r_{1} r_{2} Q R^{2} & = & 4 r_{1} r_{3} \end{matrix} ∣ = P Q = P R + Q R$
$\Rightarrow \frac{1}{\sqrt{r_{3}}} = \frac{1}{\sqrt{r_{1}}} + \frac{1}{\sqrt{r_{2}}}$

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Two circles with radii 25 cm and 9 cm touch each other externally. Find the length of the direct common tangent.
A32 cm
B50 cm
C40 cm
D30 cm

Recommended Questions

Home
Ask Doubt
Profile