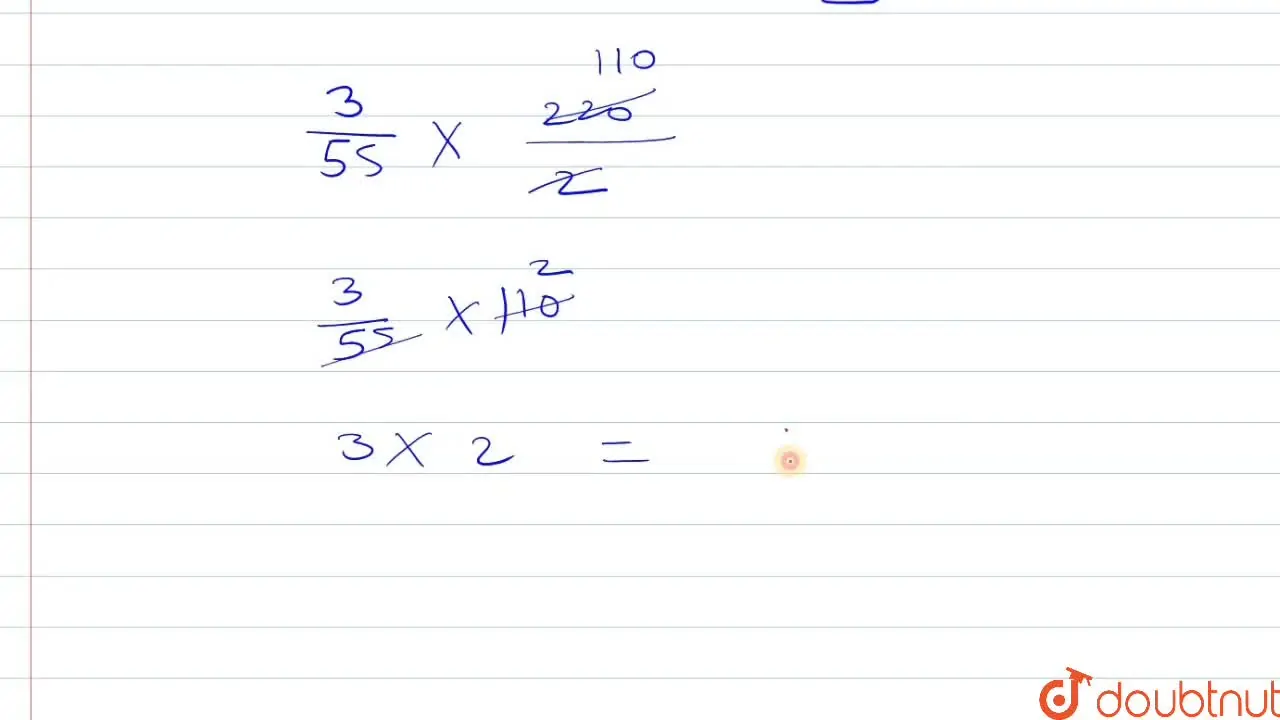Let f(x)=cos2x.cos4x.cos6x.cos8x.cos10x then limx→01−(f(x))355sin2x equals
Video Solution
Text Solution
The correct Answer is:6
6
limx→0Incos2xx2=−222,limx→0Incos4xx2=−422
|
Updated on:21/07/2023
Similar Questions
limx−01−cos4xx2 is equal to
View SolutionIf f(x)=cosxcos2xcos4xcos8xcos16x then find f'(π4)
View Solutionlimx→0(1−cos2x)sin5xx2sin3x
View SolutionIf f(x)=cosxcos2xcos4xcos(8x).cos16x then find f'(π4)
View SolutionIf f(x)=cosxcos2xcos4xcos(8x).cos16x then find f'(π4)
View SolutionIf f(x)=limn→∞tan−1(4n2(1−cos(xn)))and g(x) = limn→∞n22lncos(2xn) then limx→0e−2g(x)−ef(x)x6 equals
View Solutionlimx→0(sinx+cos3x)2/x =
View Solutionlimx→0cos2x−1cosx−1
View SolutionLet f(x)=limn→∞tan−1(4n2(1−cosπn))andg(x)=limn→∞n22lncos(2xn) then limx→0e−2g(x)−ef(x)x6 equals.
View SolutionLet f(x)=cos10x+cos8x+3cos4x+3cos2x and g(x)=8cosxcos3(3x) then for all x we have
View Solution