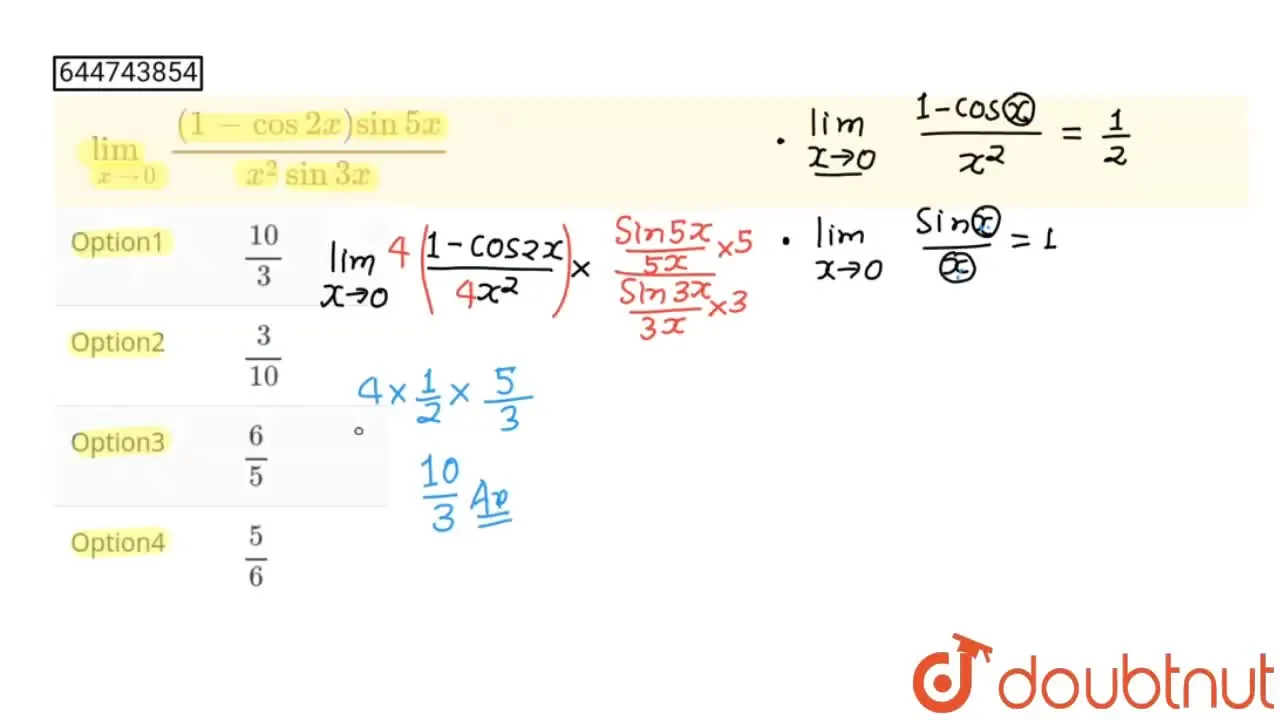
limx→0(1−cos2x)sin5xx2sin3x
A
103
B
310
C
65
D
56
Video Solution
Text Solution
The correct Answer is:A
We have,
limx→0(1−cos2x)sin5xx2sin3x
limx→02(sinxx)2×(sin5x5x)×5x3x×(3xsin3x)=103
|
Updated on:21/07/2023
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - LIMITSEXERCISE - Section I - Solved Mcqs101videos
LIMITS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - LIMITSEXERCISE - Section II - Assertion Reason Type5videos
LIMITS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Chapter Test30videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Exercise30videos
MATHEMATICAL INDUCTION
Similar Questions
Ltx→0(1−cos2x)sin5xx2sin3x is equal to
(i) 65
(ii) 56
(iii) 103
(iv) 310View Solutionlimx→0xcosx−sinxx2sinx
View SolutionEvaluate the following limit: (lim)x→02sinx−sin2xx3
View SolutionEvaluate the following limit: (lim)x→02sinx−sin2xx3
View Solutionlimx→0(1−ex)sinxx2+x3 is equal to
View Solutionthe value of limx→0{(cosx)1sin2x+sin2x+2tan−13x+3x2ln(1+3x+sin2x)+xex}
View Solutionlimx→01−cosxcos2xcos3xsin22x is equal to a) 3/4 b) 7/4 c) 7/2 d) -3/4
View Solutionlimx→02+2x+sin2x(2x+sin2x)esinx is
View Solutionlimx→0sin5xtan3x
View SolutionEvaluate the following limit: (lim)x→0sin2x(cos3x−3cosx)x3
View Solution