A rod of negligible heat capacity has a length of 50 cm, area of cross section 5cm2 and thermal conductivity 500 W/m∘C. The temperature of one end is maintained at 0∘C and that fo the other end is slowly and linearly varied from 0∘C to 60∘C in 20 min. Assuming no heat loss through the lateral side, the total heat transmitted throught he rod in 20 minutes is
18 kJ
8 kJ
27 kJ
4.5 kJ
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Understand the given data
- Length of the rod, L=50cm=0.5m
- Area of cross-section, A=5cm2=5×10−4m2
- Thermal conductivity, k=500W/m°C
- Temperature at one end, T1=0°C
- Temperature at the other end, T2 varies from 0°C to 60°C over 20min=1200s
Step 2: Determine the average temperature difference
Since the temperature at the other end varies linearly, we can find the average temperature difference over the time interval. The temperature at the other end increases linearly from 0°C to 60°C.
The average temperature difference ΔT can be calculated as:
ΔT=T1+T22=0+602=30°C
Step 3: Use the heat conduction formula
The rate of heat transfer dQdt through the rod is given by Fourier's law of heat conduction:
dQdt=k⋅A⋅(T2−T1)L
Substituting the average temperature difference:
dQdt=k⋅A⋅ΔTL
Step 4: Substitute the values
Now substituting the known values:
dQdt=500W/m°C⋅5×10−4m2⋅30°C0.5m
Calculating this:
dQdt=500⋅5×10−4⋅300.5
=500⋅5⋅30×10−40.5
=7500×10−40.5=15000×10−4=1.5W
Step 5: Calculate total heat transmitted in 20 minutes
To find the total heat transmitted Q over 1200s:
Q=dQdt⋅t=1.5W⋅1200s
Q=1800J
Step 6: Convert to kilojoules
Q=18001000=1.8kJ
Final Answer
The total heat transmitted through the rod in 20 minutes is 1.8 kJ.
---
- Length of the rod, L=50cm=0.5m
- Area of cross-section, A=5cm2=5×10−4m2
- Thermal conductivity, k=500W/m°C
- Temperature at one end, T1=0°C
- Temperature at the other end, T2 varies from 0°C to 60°C over 20min=1200s
Since the temperature at the other end varies linearly, we can find the average temperature difference over the time interval. The temperature at the other end increases linearly from 0°C to 60°C.
ΔT=T1+T22=0+602=30°C
The rate of heat transfer dQdt through the rod is given by Fourier's law of heat conduction:
dQdt=k⋅A⋅(T2−T1)L
dQdt=k⋅A⋅ΔTL
Now substituting the known values:
dQdt=500W/m°C⋅5×10−4m2⋅30°C0.5m
dQdt=500⋅5×10−4⋅300.5
=500⋅5⋅30×10−40.5
=7500×10−40.5=15000×10−4=1.5W
To find the total heat transmitted Q over 1200s:
Q=dQdt⋅t=1.5W⋅1200s
Q=1800J
Q=18001000=1.8kJ
The total heat transmitted through the rod in 20 minutes is 1.8 kJ.
Similar Questions
A rod of negligible heat capacity has length 20cm, area of cross section 1.0cm2 and thermal conductivity 200Wm−1C−1 . The temperature of one end is maintained at 0∘C and that of the other end is slowly and linearly varied from 0∘C to 60∘C in 10 minutes. Assuming no loss of heat through the sides, find the total heat transmitted through the rod in these 10 minutes.
View SolutionOne end of conducting rod is maintained at temperature 50∘C and at the other end ice is melting at 0∘C. The rate of melting of ice is doubled if:
View SolutionA rod AB is 1m long. The temperature of its one end A is maintained at 100∘C and other end B at 10∘C, the temperature at a distance of 60 cm from point B is
View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross section 100cm2. The thermal conductivity of copper is 400W/m/∘C The two ends of this rod must be kept at a temperature difference of
View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross section 100cm2. The thermal conductivity of copper is 400W/m/∘C The two ends of this rod must be kept at a temperature difference of
View SolutionThree rods of equal length, area of cross section and thermal conductivity are connected as shown in the figure.
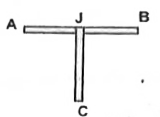
There is no heat loss through lateral surface of the rods and temperature of ends are TA=100∘C,TB=70∘C and TC=50∘C. Find the temperature of junction.
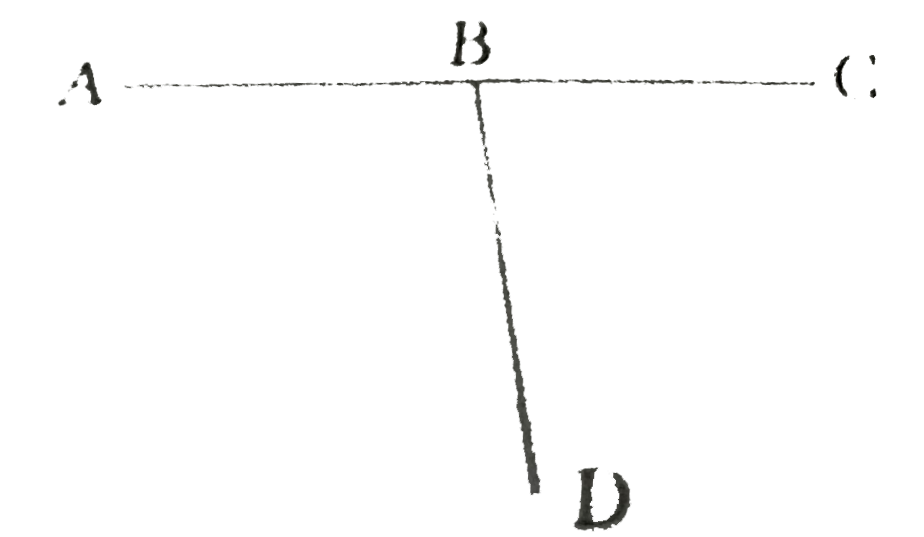
View SolutionThree conducting rods of same material and cross-section are shown in figure. Temperatures of A,D and C are maintained at 20∘C,90∘Cand0∘C. The ratio of lengths BD and BC if there is no heat flow in AB is
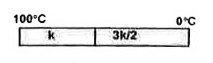
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View SolutionThe length of the two rods made up of the same metal and having the same area of cross-section are 0.6 m and 0.8 m respectively. The temperature between the ends of first rod is 90∘ C and 60∘ C and that for the other rod is 150 and 110∘ C. For which rod the rate of conduction will be greater
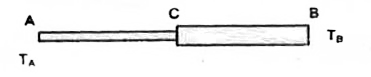
View SolutionTwo rods of same length and same material having area of cross section A and 4A respectively are joined as shown in the figure. If TA and TB are temperatures of free ends in steady state. The temperature of junction point C is (Assume no heat loss wil take place through lateral surface)
View Solution
