An infinitely long cylinderical wire of radius R is carrying a current with current density j=αr3 (where α is constant and r is the distance from the axis of the wire). If the magnetic fixed at r=R2 is B1 and at r=2R is B2 then the ratio B2B1 is
2
4
6
8
The correct Answer is:D
To solve the problem, we need to find the ratio of the magnetic fields B2 and B1 at two different distances from the axis of an infinitely long cylindrical wire carrying a current with a given current density.
1. Understanding Current Density:
The current density j is given by:
j=αr3
where α is a constant and r is the distance from the axis of the wire.
2. Finding Total Current Enclosed:
To find the magnetic field inside the wire at r=R2, we will first calculate the total current I enclosed within a radius r:
I=∫r0j⋅dA=∫r0j⋅2πr′dr′
Substituting j:
I=∫r0α(r′)3⋅2πr′dr′=2πα∫r0(r′)4dr′
Evaluating the integral:
I=2πα((r′)55)r0=2παr55
3. Applying Ampere's Law:
According to Ampere's Law, the magnetic field B at a distance r from the center is given by:
B⋅2πr=μ0I
Therefore,
B=μ0I2πr
Substituting for I:
B=μ02πr⋅2παr55=μ0αr45
4. Finding B1 at r=R2:
Now, substituting r=R2:
B1=μ0α(R2)45=μ0αR45⋅16=μ0αR480
5. Finding B2 at r=2R:
For r=2R, we consider the total current I flowing through the entire wire:
I=∫R0j⋅2πr′dr′=2πα∫R0(r′)4dr′=2πα((r′)55)R0=2παR55
Now applying Ampere's Law for r=2R:
B2⋅2π(2R)=μ0I
Thus,
B2=μ0I4πR=μ04πR⋅2παR55=μ0αR410
6. Finding the Ratio B2B1:
Now we can find the ratio:
B2B1=μ0αR410μ0αR480=8010=8
Final Answer:
The ratio B2B1 is 8.
The current density j is given by:
j=αr3
where α is a constant and r is the distance from the axis of the wire.
To find the magnetic field inside the wire at r=R2, we will first calculate the total current I enclosed within a radius r:
I=∫r0j⋅dA=∫r0j⋅2πr′dr′
Substituting j:
I=∫r0α(r′)3⋅2πr′dr′=2πα∫r0(r′)4dr′
Evaluating the integral:
I=2πα((r′)55)r0=2παr55
According to Ampere's Law, the magnetic field B at a distance r from the center is given by:
B⋅2πr=μ0I
Therefore,
B=μ0I2πr
Substituting for I:
B=μ02πr⋅2παr55=μ0αr45
Now, substituting r=R2:
B1=μ0α(R2)45=μ0αR45⋅16=μ0αR480
For r=2R, we consider the total current I flowing through the entire wire:
I=∫R0j⋅2πr′dr′=2πα∫R0(r′)4dr′=2πα((r′)55)R0=2παR55
Now applying Ampere's Law for r=2R:
B2⋅2π(2R)=μ0I
Thus,
B2=μ0I4πR=μ04πR⋅2παR55=μ0αR410
Now we can find the ratio:
B2B1=μ0αR410μ0αR480=8010=8
The ratio B2B1 is 8.
Similar Questions
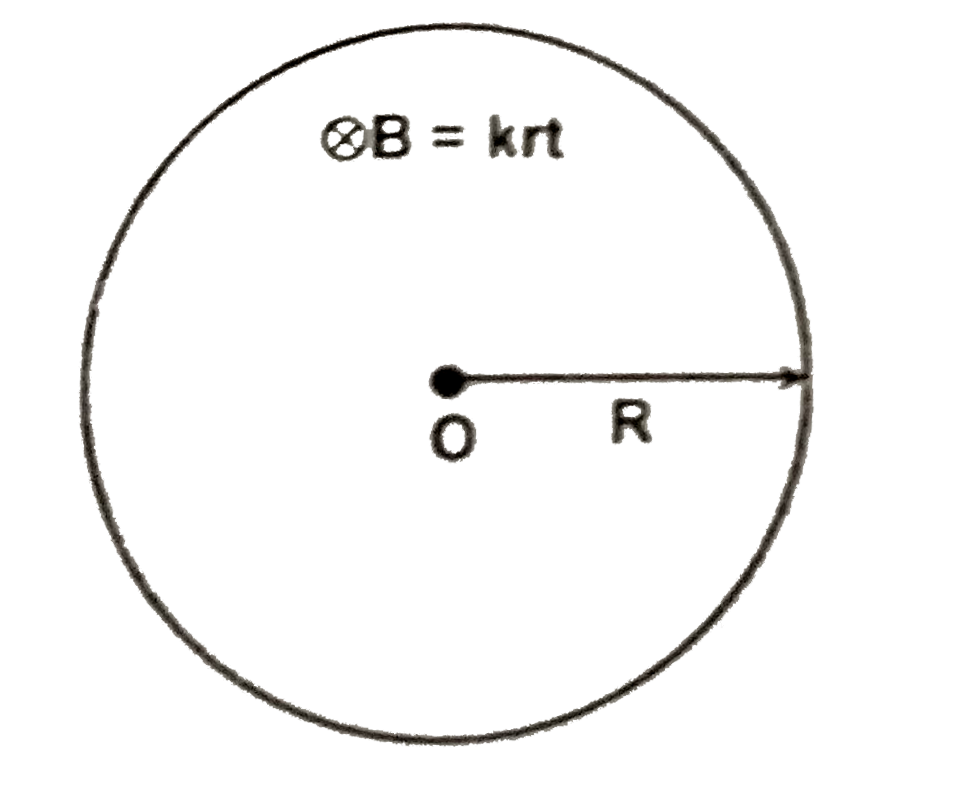
A time varying magnetic field B=krt (where k is a constant r is the radial distance from centre O) is existing in a circular region of radius R as shown. If induced electric field at r=R2 is E1 and at r=2R is E2 then the ratio E2E1 is
View SolutionSuppose that the current density in a wire of radius a varius with r according to Kr2 where K is a constant and r is the distance from the axis of the wire. Find the magnetic field at a point at distance r form the axis when (a) r<a and (b) r>a.
View SolutionA circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
View SolutionA long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis

View SolutionAn infinite cylindrical wire of radius R and having current density varying with its radius r as, J=J0[1−(r/R)]. Then answer the following questions.
Graph between the magnetic field and radius is
View SolutionFor an infinitely long solid cylinder of radius R having a uniform density , the gravitational field at a distance r1 is E1 and at a distance r2 is E2 , then
View SolutionA circular coil of radius 2R is carrying current 'i' . The ratio of magnetic fields at the centre of the coil and at a point at a distance 6R from the centre of the coil on the axis of the coil is
View SolutionA cylinderical conductor of radius R is carrying constant current. The plot of the magnitude of the magnetic field, B with the distance, d from the centre of the conductor , is correctly represented by the figure:
View SolutionA thin but long, hollow, cylindrical tube of radius r carries a current i along its length. Find the magnitude of the magnetic field at a distance r/2 from the surface (a) inside the tube (b) outside the tube.
View SolutionA long cylidrical conductor of radius R carries a current i as shown in figure. The current desity J is a function of radius according to J=br, where b is a constant. Find an expression for the magnetic field B
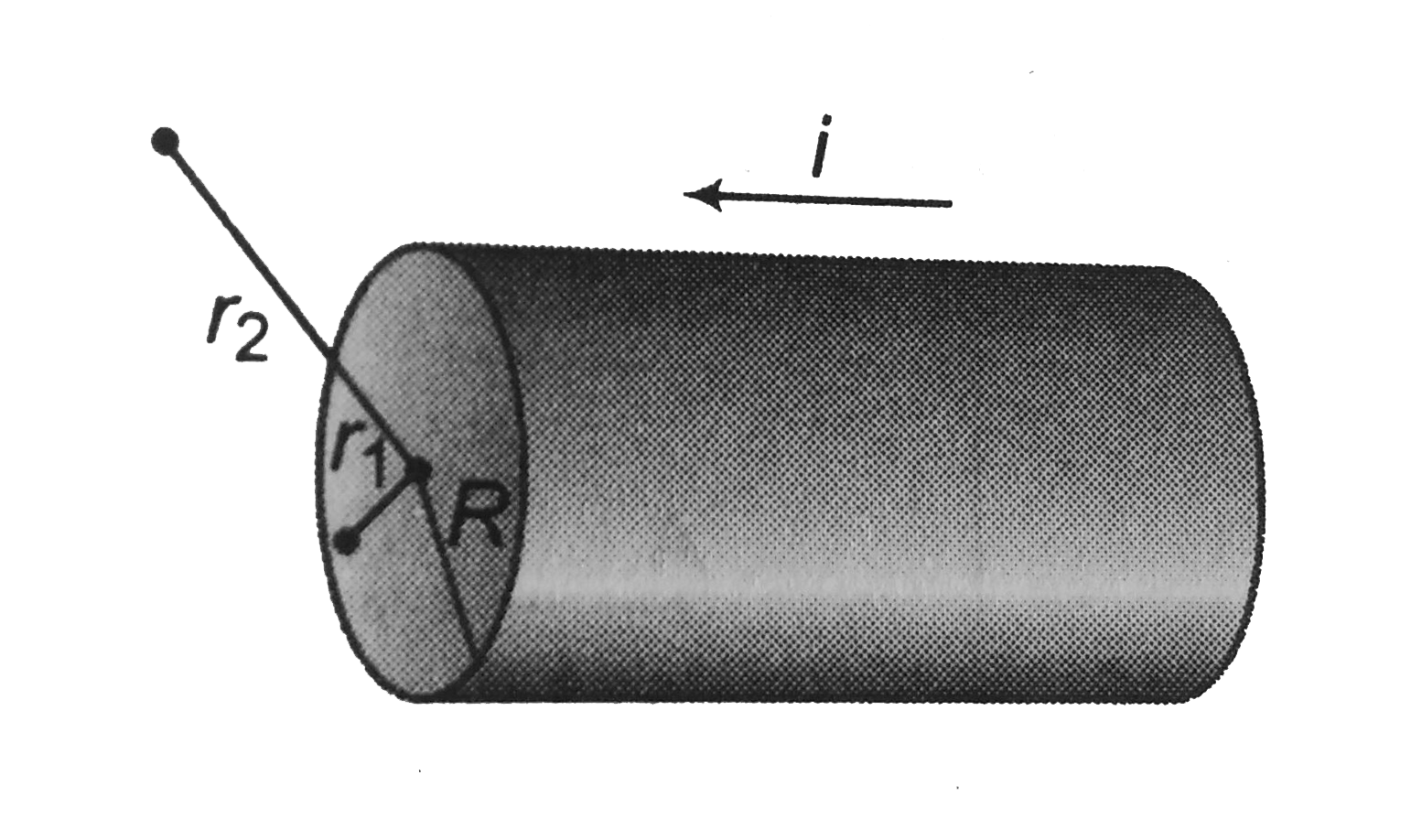
a. at a distasnce r1<R and
b.at a distance r2>R, measured from the axis.
View Solution