A point mass performs straight line motion along positive x-axis At t=0 point mass is at point A(x1,0) it moves such that its velocity is given by v=ax, where a is positive constant and x is the x-coordinate of position vector mass at a certain time t. Find the time required to move from A to B (x2,0)
x22−x212a
x22−x21a
2x22−x212a
2x22−x21a
The correct Answer is:A
To solve the problem step by step, we will analyze the motion of the point mass and derive the time required to move from point A to point B.
Step 1: Understand the given information
We know that:
- The point mass starts at position A(x1,0) at time t=0.
- The velocity of the mass is given by the equation v=ax, where a is a positive constant and x is the position of the mass.
Step 2: Relate velocity to position and time
The velocity v can also be expressed as the derivative of position with respect to time:
v=dxdt
Setting the two expressions for velocity equal gives us:
dxdt=ax
Step 3: Rearranging the equation
We can rearrange this equation to separate variables:
xdx=adt
Step 4: Integrate both sides
Now we will integrate both sides. The left side will be integrated with respect to x from x1 to x2, and the right side will be integrated with respect to t from 0 to t:
∫x2x1xdx=∫t0adt
Step 5: Perform the integration
The left side integrates to:
x22∣∣∣x2x1=x222−x212
The right side integrates to:
at∣∣∣t0=at−0=at
Step 6: Set the integrals equal to each other
Now we can set the results of the integrals equal to each other:
x222−x212=at
Step 7: Solve for time t
Rearranging the equation gives:
x22−x212=at
Thus, solving for t:
t=x22−x212a
Final Result
The time required to move from point A to point B is:
t=x22−x212a
---
We know that:
- The point mass starts at position A(x1,0) at time t=0.
- The velocity of the mass is given by the equation v=ax, where a is a positive constant and x is the position of the mass.
The velocity v can also be expressed as the derivative of position with respect to time:
v=dxdt
Setting the two expressions for velocity equal gives us:
dxdt=ax
We can rearrange this equation to separate variables:
xdx=adt
Now we will integrate both sides. The left side will be integrated with respect to x from x1 to x2, and the right side will be integrated with respect to t from 0 to t:
∫x2x1xdx=∫t0adt
The left side integrates to:
x22∣∣∣x2x1=x222−x212
The right side integrates to:
at∣∣∣t0=at−0=at
Now we can set the results of the integrals equal to each other:
x222−x212=at
Rearranging the equation gives:
x22−x212=at
Thus, solving for t:
t=x22−x212a
The time required to move from point A to point B is:
t=x22−x212a
Similar Questions
A particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
View SolutionA particle moves in a plane such that its coordinates changes with time as x=at and y=bt, where a and b are constants. Find the position vector of the particle and its direction at any time t.
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA particle A of mass 10/7kg is moving in the positive direction of x-axis. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)

View SolutionA disc of radius R start at time t=0 moving along the positive X-axis with linear speed v and angular speed ω. Find the x and y-coordinates of the bottommost point at any time t.
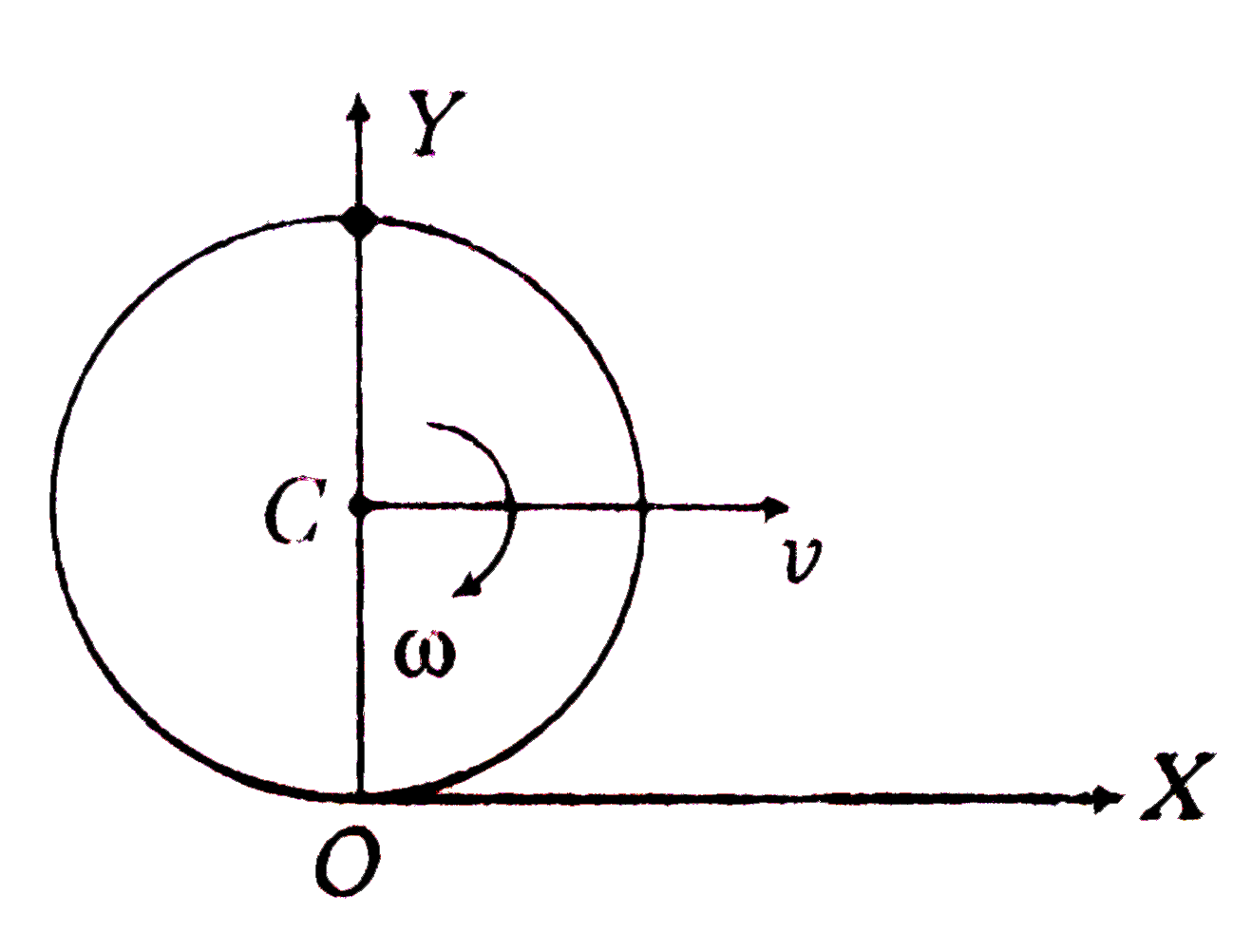
View SolutionTwo point masses m1 and m2 are connected by a spring of natural length l0. The spring is compressed such that the two point masses touch each other and then they are fastened by a string. Then the system is moved with a velocity v0 along positive x-axis. When the system reached the origin, the string breaks (t=0). The position of the point mass m1 is given by x1=v0t−A(1−cosωt) where A and ω are constants. Find the position of the second block as a function of time. Also, find the relation between A and l0.
View SolutionA particle A of mass 10/7kg is moving in the positive direction of x−aξs. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)

View SolutionThe position (x) of body moving along x-axis at time (t) is given by x=3f2 where x is in matre and t is in second. If mass of body is 2 kg, then find the instantaneous power delivered to body by force acting on it at t=4 s.
View SolutionThe reation between the time t and position x for a particle moving on x-axis is given by t=px2+qx, where p and q are constants. The relation between velocity v and acceleration a is as
View SolutionThe velocity (v) of a particle of mass m moving along x-axis is given by v=α√x, where α is a constant. Find work done by force acting on particle during its motion from x=0 to x=2m :-
View Solution
