A particle A of mass 10/7kg is moving in the positive direction of x-axis. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)

(a) 4ms−1
(b) 2ms−1
(c) 3√2ms−1
(d) 1003ms−1
The correct Answer is:A
Area under P−x graph
=∫pdx=(mdvdt)vdx=v∫1mv2dV
=[mv33]v1=107×3(v3−1)
From the graph, area=12(2+4)×10=30
or 107×3(v3−1)=30
or v=4ms−1
Alternative method:
From graph
P=0.2x+2
mvdvdxv=0.2x+2
mv2dv=(0.2x+2)dx
Now integrating both sides, we get
v∫1mv2dv=10∫0(0.2x+2)dx
v=4ms−1
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension
55 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective
23 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A particle of mass 10−2kg is moving along the positive x axis under the influence of a force F(x)=−K/(2x2) where K=10−2Nm2. At time t=0 it is at x=1.0m and its velocity is v=0.
(a) Find its velocity when it reaches x=0.50m.
(b) Find the time at which it reaches x=0.25m.
View SolutionThe velocity of a particle moving in the positive direction of x-axis veries as v=10√x. Assuming that at t=0, particle was at x=0
View SolutionA particle of mass 1031kg is moving with a velocity equal to 105ms−1. The wavelength of the particle is equal to
View SolutionA particle of mass m1 moving with velocity v in a positive direction collides elastically with a mass m2 moving in opposite direction also at velocity v. If m2>>m1, then
View SolutionA particle moving in the positive x-direction has initial velocity v0. The particle undergoes retardation kv2 , where vis its instantaneous velocity. The velocity of the particle as a function of time is given by:
View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V-t graph.
View SolutionA particle is moving in a straight line according to graph given below : (a is acceleration, v is velocity, x is position, t is time) :
View SolutionThe acceleration of a particle which moves along the positive x-axis varies with its position as shown in figure. If the velocity of the particle is 0.8ms−1 at x = 0, then velocity of the particle at x=1.4m is (∈ms−1).
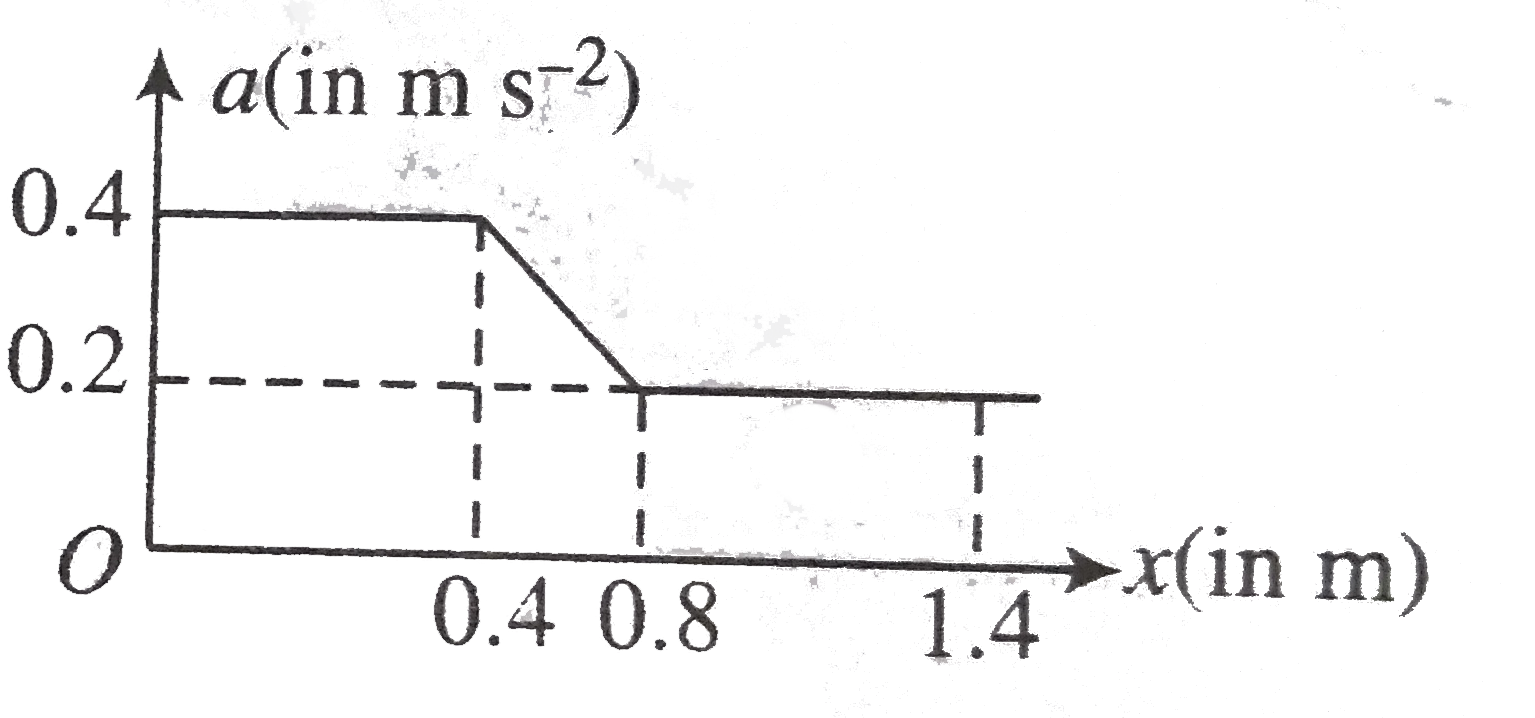
View SolutionA particle is moving along x-direction with a constant acceleration a. The particle starts from x=x0 position with initial velocity u. We can define the position of the particle with time by the relation
x=x0+ut+12at2
plot the position of the particle in relation with time is following situations
(i) If initial position of the particle is on negativ x-axis, initial velocity is positive and acceleration is negative.
(ii) If initial position is positive, initial velocity is negative and acceleration is positive.
View SolutionA ball of mass 200g is moving with a velocity of 10msec−1 . If the error in measurement of velocity is 0.1%, the uncertainty in its position is :
View Solution
