Assertion: If position vector is given by ˆr=sinhati+costˆj−7tˆk, then magnitude of acceleration |→a|=1
Reason: The angles which the vector →A=A1→i+A3ˆk makes with the co-ordinate
asex are given by cosα=A1A,cosβ=A2A&cosγ=A3A.
The correct Answer is:Angular momentum : [ML2T−1]; Latent heat : [M−1L−2T2Q2]; Torque : [ML2T−2];
Capacitance : [L2T−2]; Inductance : [ML3T−1Q−2]; Resistivity : [ML2Q−2]
Step by step video, text & image solution for Assertion: If position vector is given by hatr=sinhati+costhatj-7thatk, then magnitude of acceleration |veca|=1 Reason: The angles which the vector vecA=A_(1)veci+A_(3)hatk makes with the co-ordinate asex are given by cos alpha=(A_(1))/(A), cos beta=(A_(2))/(A)& cos gamma=(A_(3))/(A). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-01
24 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-02
53 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-5(A)
15 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
The direction cosines of a vector a are cosα=45√2,cosβ=1√2 and cosγ=35√2 then the vector →A is
View SolutionAssertion: The angle between the two vectors (ˆi+ˆj) and (ˆj+ˆk) is π3 radian.
Reason: Angle between two vectors →A and →B is given by θ=cos−1(→A.→BAB)
View Solutiona1,a2,a3∈R−{0}anda1+a2cos2x+a3sin2x=0 " for all " x in R then (a) vectors →a=a1ˆi+a2ˆj+a3ˆkand→b=4ˆi+2ˆj+ˆk are perpendicular to each other (b)vectors →a=a1ˆi+a2ˆj+a3ˆkand→b=ˆi+ˆj+2ˆk are parallel to each each other (c)if vector →a=a1ˆi+a2ˆj+a3ˆk is of length √6 units, then on of the ordered trippplet (a1,a2,a3)=(1,−1,−2) (d)if 2a1+3a2+6a3=26, then ∣∣→aˆi+a2ˆj+a3ˆk∣∣is2√6
View SolutionFind the relation between acceleration of blocks a1,a2 and a3.

View SolutionFor A2×2 matrix, whose elements are given by: aij=12|−3i+j|. Find a11+a22
View SolutionIf a1anda2 aare two non- collineaar unit vectors and if |a1+a2|=√3, ,then value of (a1−a2).(2a1−a2) is
View SolutionLet →a,→b,→c be vectors of equal magnitude such that the angle between →aand→b is α,→band→c is βand→cand→a is γ .then minimum value of cosα+cosβ+cosγ is
View SolutionIf →a1and→a2 are two non-collinear unit vectors and if ∣∣→a1+→a2∣∣=√3, then the value of (→a1−→a2).(2→a1+→a2) is :
View SolutionIf a1 and a2 are two values of a for which the unit vector a→i+b→j+12→k is linearly dependent with →i+2→j and →j−2→k, then 1a1+1a2 is equal to
View SolutionIn given system a1anda2 are the accelerations of blocks 1 and 2. Then constraint relation is
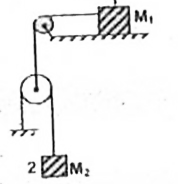
View Solution
