Frequency f of a simple pendulum depends on its length l and acceleration g due to gravity according to the following equation f=12π√gl
Graph between which of the following quantities is a straight line?
The correct Answer is:[ML5T−2]
[P]=[aV2]⇒[a]=[PV2]=[ML−1T−2][L6]=[ML5T−2]
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-01
24 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-02
53 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-5(A)
15 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
Knowledge Check
Which of the following is equivalent to f2g+f ?
Afg(f+g)Bf(fg+f)Cf2(1g−1f)Df2(1g+1)The time period of a simple pendulum is given by T=2π√L/g, where L is length and g acceleration due to gravity. Measured value of length is 10cm known to 1mm accuracy and time for 50 oscillations of the pendulum is 80 s using a wrist watch of 1 s resloution. What is the accuracy in the determination of g?
A0.02B0.03C0.04D0.05IF f(x)=√x and the composite function f(g(x))=√4x2−5, which of the following could be g(x) ?
A√4x4−5B√16x4−25C2x2−25D4x2−5
The time period of a simple pendulum of length L as measured in an elevator descending with acceleration g / 3 is
View SolutionThe velocity of water wave v may depend on their wavelength λ, the density of water ρ and the acceleration due to gravity g. The method of dimensions gives the relation between these quantities as
View SolutionCopper of fixed volume V is drawn into wire of length l. When this wire is subjected to a constant force F, the extension produced in the wire is △l. Which of the following graphs is a straight line?
View SolutionConsider the following function f whose graph is given below.
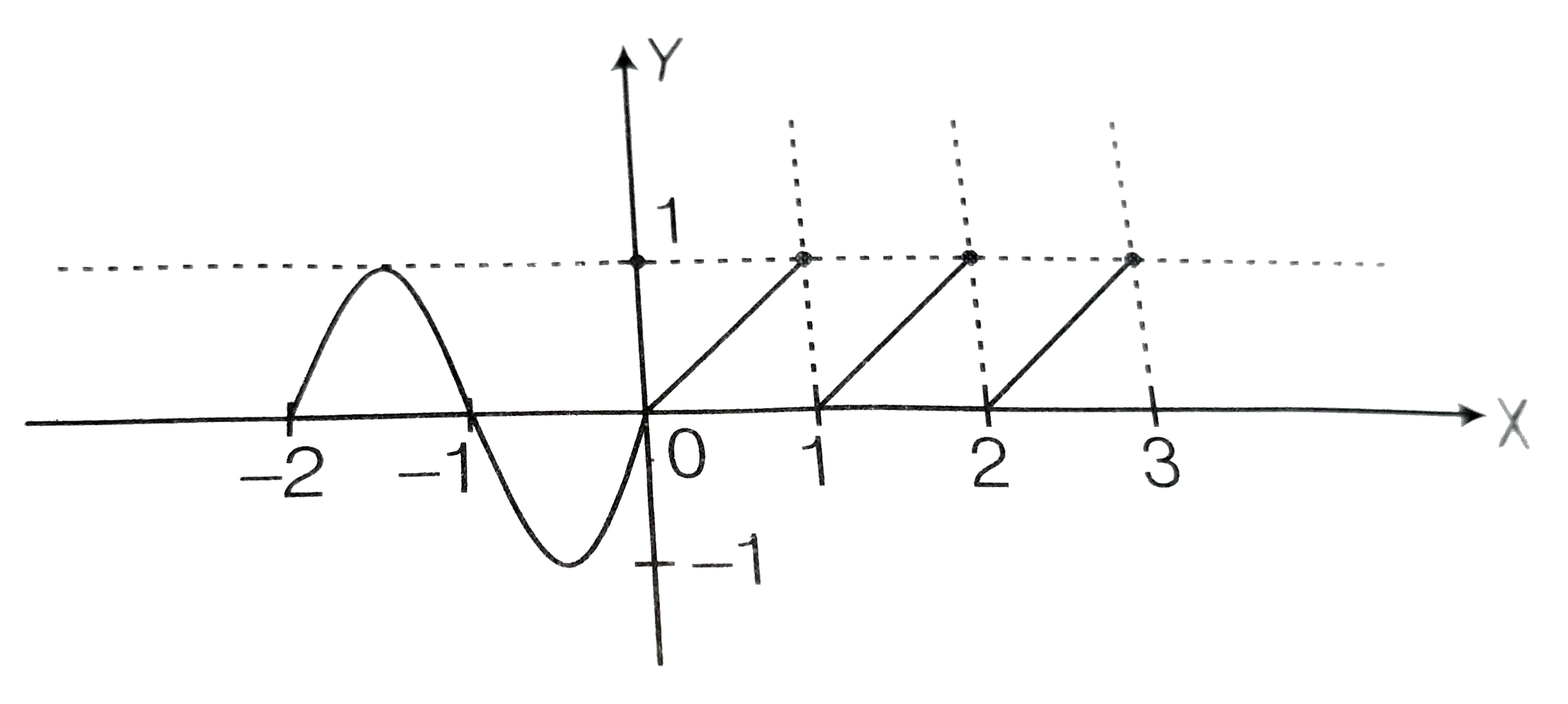
Draw the graph of following functions.
(g) 2f(x)
View SolutionGiven that T stands for time period nd 1 stands for the length of simple pendulum. If g is the acceleration due to gravity, then which of the following statements about the relation T2=lg is correct ?
(I) It is correct both dimensionally as well unmerically
(II) It is neither dimensionally correct nor numerically
(III) It is dimensionally correct but not numerically
(IV) It is numerically correct but not dimensionally
View Solution
