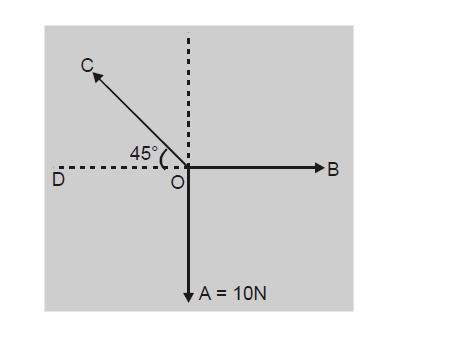
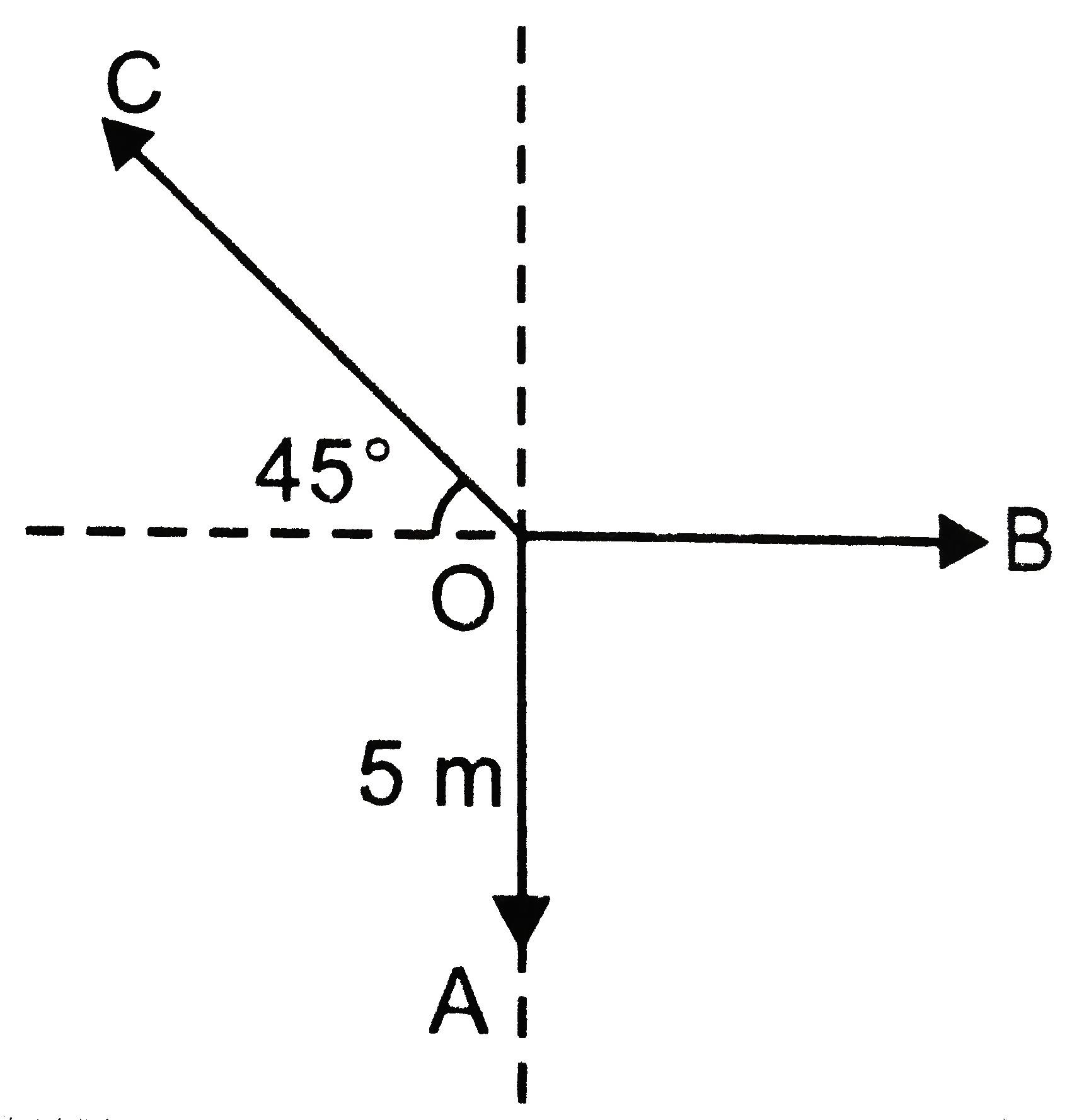
The sum of three vectors shown in Fig. 2 ( c) . 77. is zero .
 .
.
(a) What is the magnitude of the vector −−→OB?
(b) What is the magnitude of the vector −−→OC ?
Dot product of two mutually perpendicular vectors is zero →A.→B=0
∴(4ˆi+nˆj−2ˆk).(2ˆi+3ˆj+ˆk)=0⇒(4×2)+(n×3)+(−2×1)=0⇒3n=−6⇒n=−2
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exersice-03
7 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - ASSERTION-REASON
11 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
Knowledge Check
The magnitude of the x-component of vector →A is 3 and the magnitude of vector →A is 5. What is the magnitude of the y-component of vector →A ?
A3B4C5D8
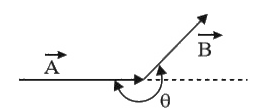
What is the angle between the vector →A and →B as shown in figure ?
View Solution→A is a vector of magnitude 2.7 units due east . What is the magnitude and direction of vector 4 →A ?
View Solutiona, b are the magnitudes of vectors →a & →b. If →a×→b=0 the value of →a.→b is
View SolutionA vector →B which has a magnitude 8.0 is added to a vector →A which lies along the x-axis. The sum of these two vector is a third vector which lies along the y-axis and has a magnitude that is twice the magnitude of →A. Find the magnitude of →A
View SolutionWhat are the properties fo two vectors →aand→b such that →a+→b=→a−→b ?
View SolutionThe resultant of two vectors →A and →B is perpendicular to the vector →A and its magnitudes is equal to half of the magnitudes of vector →B (figure). The angle between →A and →B is

View SolutionA vector →A of length 10 units makes an angle of 60∘ with a vector →B of length 6 units. Find the magnitude of the vector difference →A−→B & the angles with vector →A.
View Solution