An object of mass m is attached to a spring. The restroing force of the spring is F=−λx3, where x is the displacement. The oscillation period depends on the mass, lmabd and oscillation amplitude. Suppose the object is initially at rest. If the initial displacement is D then its period is τ. If the initial displacement is 2D, find the period.
8τ
2τ
τ
τ/2
The correct Answer is:D
To solve the problem, we need to find the relationship between the period of oscillation and the amplitude of the oscillation for the given restoring force F=−λx3.
1. Understanding the Force: The restoring force of the spring is given by F=−λx3. This indicates that the force is non-linear and depends on the cube of the displacement x.
2. Identifying the Period Dependence: The period T of oscillation for a system can generally be expressed as a function of mass m, spring constant λ, and amplitude A. We can express this as:
T=k⋅ma⋅λb⋅Ac
where k is a constant, and a,b,c are the powers to which each variable is raised.
3. Finding the Dimensions: We need to analyze the dimensions of each variable:
- Mass m has the dimension (M).
- The spring constant λ has dimensions derived from the force equation. Since F=m⋅a and a=d2xdt2, we can express λ as having dimensions (M)(L−2) when considering the force F=−λx3.
4. Setting Up the Equation: From the dimensional analysis, we can write:
(T)=(Ma)(λb)(Ac)⟹(T)=(Ma)(Mb)(L−2b)(Lc)
This simplifies to:
(T)=(Ma+b)(Lc−2b)
5. Equating Dimensions: Since the dimension of time (T) is (L0)(M0)(T1), we can set up the following equations:
- For mass: a+b=0
- For length: c−2b=1
6. Solving the Equations:
- From a+b=0, we have a=−b.
- Substituting a=−b into c−2b=1:
c−2(−a)=1⟹c+2a=1
- We can express c in terms of a:
c=1−2a
7. Finding the Relationship: Now, we know that the period T depends on the amplitude A. If we assume A=D gives period τ, then:
τ∝Dc⟹τ=k⋅ma⋅λb⋅Dc
When A=2D:
T′=k⋅ma⋅λb⋅(2D)c=k⋅ma⋅λb⋅2c⋅Dc
Thus:
T′=2c⋅τ
8. Substituting for c: Since c=1−2a, we can substitute this into the equation:
T′=2(1−2a)⋅τ
9. Conclusion: The period when the initial displacement is 2D can be expressed as:
T′=2(1−2a)⋅τ
Final Result
The period when the initial displacement is 2D is T′=2(1−2a)⋅τ.
T=k⋅ma⋅λb⋅Ac
where k is a constant, and a,b,c are the powers to which each variable is raised.
- Mass m has the dimension (M).
- The spring constant λ has dimensions derived from the force equation. Since F=m⋅a and a=d2xdt2, we can express λ as having dimensions (M)(L−2) when considering the force F=−λx3.
(T)=(Ma)(λb)(Ac)⟹(T)=(Ma)(Mb)(L−2b)(Lc)
This simplifies to:
(T)=(Ma+b)(Lc−2b)
- For mass: a+b=0
- For length: c−2b=1
- From a+b=0, we have a=−b.
- Substituting a=−b into c−2b=1:
c−2(−a)=1⟹c+2a=1
- We can express c in terms of a:
c=1−2a
τ∝Dc⟹τ=k⋅ma⋅λb⋅Dc
When A=2D:
T′=k⋅ma⋅λb⋅(2D)c=k⋅ma⋅λb⋅2c⋅Dc
Thus:
T′=2c⋅τ
T′=2(1−2a)⋅τ
T′=2(1−2a)⋅τ
The period when the initial displacement is 2D is T′=2(1−2a)⋅τ.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-02
19 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A block of mass m hangs from three springs having same spring constant k. If the mass is slightly displaced downwards, the time period of oscillation will be

View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View Solution
Knowledge Check
A particle moves such that its accleration a is given by a=−bx, where x is the displacement from equilibrium position and b is a constant. The period of oscillation is
A√2π/bB2π/√bC2π/bD2√(π/b)
A block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

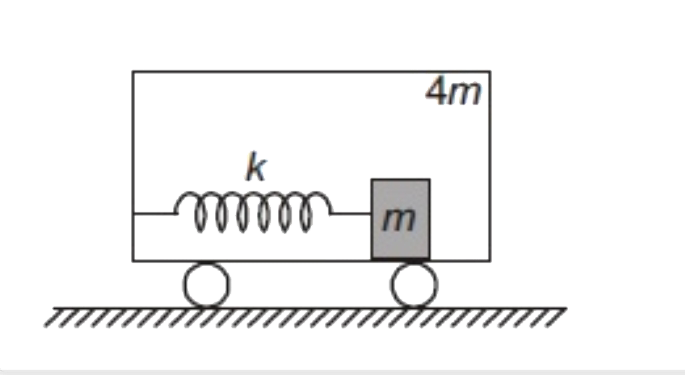
View SolutionA block of mass m is attached to a cart of mass 4m through spring of spring constant k as shown in the figure. Friction is absent everywhere. The time period of oscillations of the system, when spring is compressed and then released, is
View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View SolutionDerive an experession for the period of oscillation of a mass attached to a spring executing simple harmonic motion. Given that the period of oscillation depends on the mass m and the force constant k, where k is the force required to stretch the spring by unit length?
View SolutionA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is

View SolutionWhen a mass m attached to a spring it oscillates with period 4s. When an additional mass of 2 kg is attached to a spring, time period increases by 1s. The value of m is :-
View Solution
