A convex mirror of focal length 'f' is placed at the origin with its reflecting surface towards the negative x-axis . Choose the correct graps between 'v' and 'u' for u<0.
_E01_010_O01.png)
_E01_010_O02.png)
_E01_010_O03.png)
_E01_010_O04.png)
The correct Answer is:C
To solve the problem, we need to analyze the relationship between the object distance (u) and the image distance (v) for a convex mirror. The mirror formula we will use is:
1v+1u=1f
Where:
- v is the image distance,
- u is the object distance (which is negative for real objects in this case),
- f is the focal length of the convex mirror (which is positive).
1. Understanding the Sign Convention:
- For a convex mirror, the focal length f is positive.
- The object distance u is negative because the object is placed on the negative x-axis (to the left of the mirror).
- The image distance v will also be positive since the image formed by a convex mirror is virtual and located on the positive side of the axis.
2. Using the Mirror Formula:
- Rearranging the mirror formula:
1v=1f−1u
- Since u<0, we can express it as:
1v=1f+1|u|
Here, |u| is the magnitude of u.
3. Analyzing the Relationship:
- As |u| increases (meaning the object moves further away from the mirror), 1|u| decreases, which means 1v approaches 1f.
- Therefore, v approaches f as |u| approaches infinity.
4. Finding Specific Values:
- If u approaches 0 (which is not physically possible for a real object but helps in understanding the trend), then:
v→0
- If u approaches −∞, then v approaches f.
5. Graphing the Relationship:
- The graph of v versus u will show that as u becomes less negative (approaching 0), v also approaches 0.
- As u becomes more negative (approaching −∞), v approaches f.
Conclusion:
The correct graph between v and u for u<0 will show a curve that starts from v=0 when u=0 and approaches v=f as |u| increases.
- v is the image distance,
- u is the object distance (which is negative for real objects in this case),
- f is the focal length of the convex mirror (which is positive).
- For a convex mirror, the focal length f is positive.
- The object distance u is negative because the object is placed on the negative x-axis (to the left of the mirror).
- The image distance v will also be positive since the image formed by a convex mirror is virtual and located on the positive side of the axis.
- Rearranging the mirror formula:
1v=1f−1u
- Since u<0, we can express it as:
1v=1f+1|u|
Here, |u| is the magnitude of u.
- As |u| increases (meaning the object moves further away from the mirror), 1|u| decreases, which means 1v approaches 1f.
- Therefore, v approaches f as |u| approaches infinity.
- If u approaches 0 (which is not physically possible for a real object but helps in understanding the trend), then:
v→0
- If u approaches −∞, then v approaches f.
- The graph of v versus u will show that as u becomes less negative (approaching 0), v also approaches 0.
- As u becomes more negative (approaching −∞), v approaches f.
The correct graph between v and u for u<0 will show a curve that starts from v=0 when u=0 and approaches v=f as |u| increases.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-2
44 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-3
12 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B) (ASSERTION & REASON )
9 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A convex mirror has a focal length f. A real object is placed at a distance f in front of it from the pole produces an image at
View SolutionAn object is placed midway between a concave mirror of focal length f and a convex mirror of focal length f. The distance between the two mirrors is 6f. Trace the ray that is first incident on the concave mirror and then the convex mirror.
View Solution
Knowledge Check
A plano convex lens has focal length f=20cm. If its plane surface is silvered, then new focal length will be
A20cmB40 cmC30 cmD10 cm
An object is placed in front of a concave mirror of focal length f as shown in figure. Choose the correct shape of the image.

View SolutionA thin convex lens of focal length 30cm is placed in front of a plane mirror. An object is placed at a distance x from the lens (not in between lens and mirror ) so that its final image coincides with itself. Then, the value of x is
View SolutionA concave mirror of focal length 10cm and a convex mirror of focal length 15cm are placed facing each other 40cm apart. A point object is placed between the mirrors, on their common axis and 15cm from the concave mirror. Find the position and nature of the image produced by successive reflections, first at the concave mirror and then at the convex mirror.
View SolutionA point object is placed on the principal axis of a concave mirror of focal length 20 cm. At this instant object is given a velocity v towards the axis (event-I) or perpendicular to axis (event-2). Then, speed of image
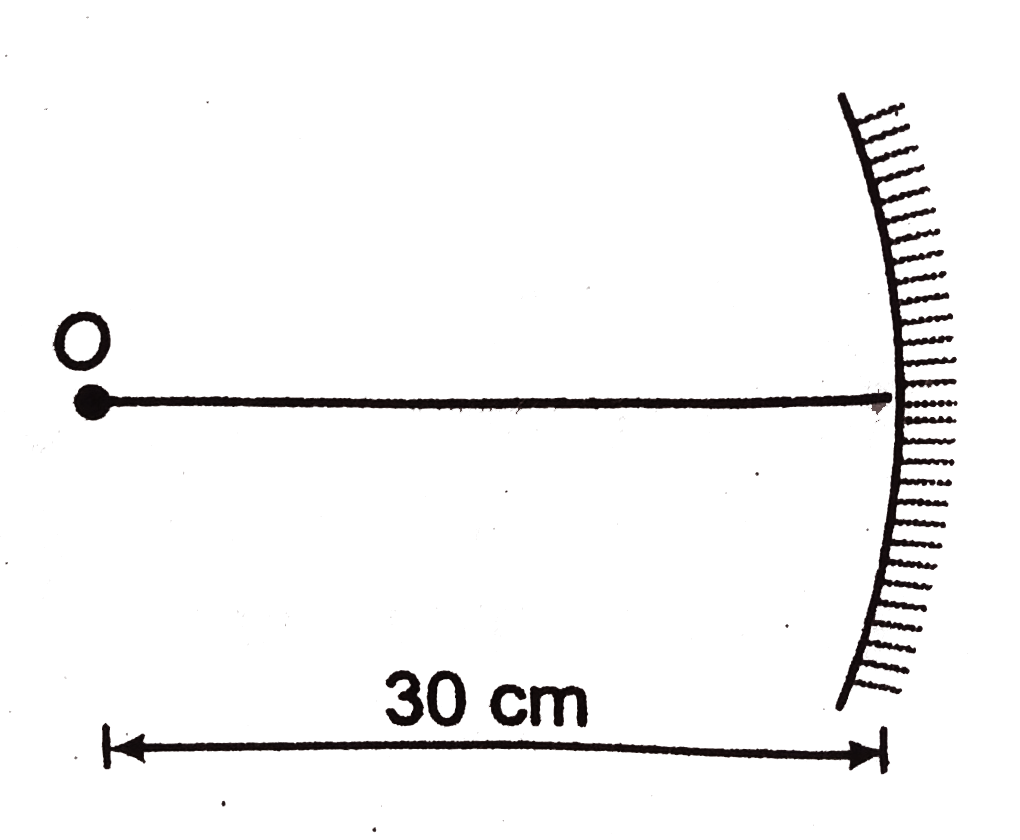
View SolutionIn an experiment for the determination of focal length of the convex mirror a convex lens of focal length 20cm is placed on the optical bench and an object pin is placed at a distance 30cm from the lens. When a convex mirror is introduced in between the lens and the real and inverted image of the object, the final image of object O is formed at O itself. If the distance between the lens and the mirror is 10cm, then the focal length of the mirror is
View SolutionA plane mirror is placed along the y-axis such that x-axis is normal to the plane of the mirror. The reflecting surface of the mirror is towards negative x-axis. The mirror moves in positive x-direction with uniform speed of 5 m/s and a point object P is moving with constant speed 3m/s in negative x-direction. find the speed of image with respect to mirror in m/s.
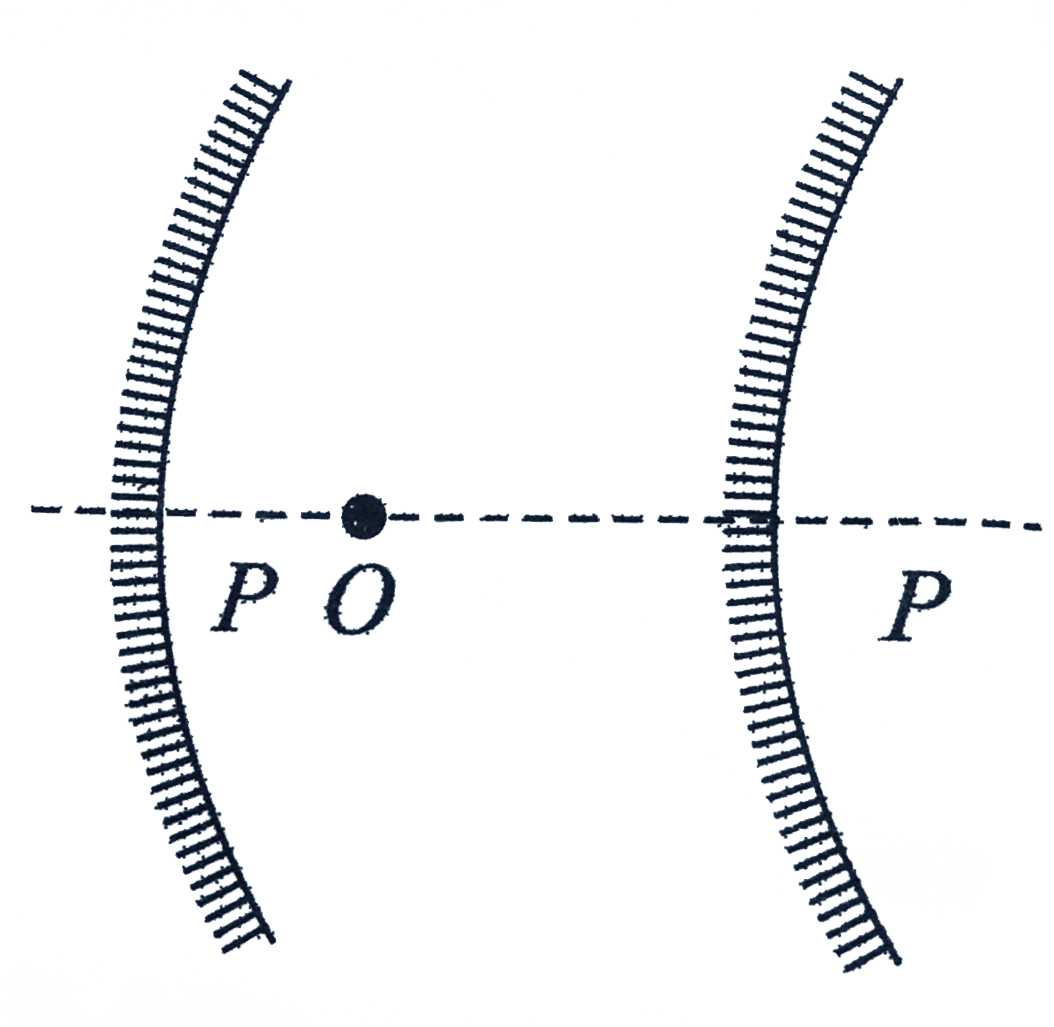
View SolutionA concave mirror of focal length 10cm is placed in front of a convex mirror of focal length 20 cm. The distance between the two mirrors is 20cm. A point object is placed 5cm from the concave mirror. Discuss the formation of image.
View Solution
