A particle is projected horizontally as shown from the rim of a large hemispherical bowl. The displacement of the particle when it strikes the bowl the first time is R. Find the velocity of the particle at that instant and the time taken.

The correct Answer is:uˆi+√√3Rgˆj,√√3Rg
∴ Vertical displacement of particle=R√32
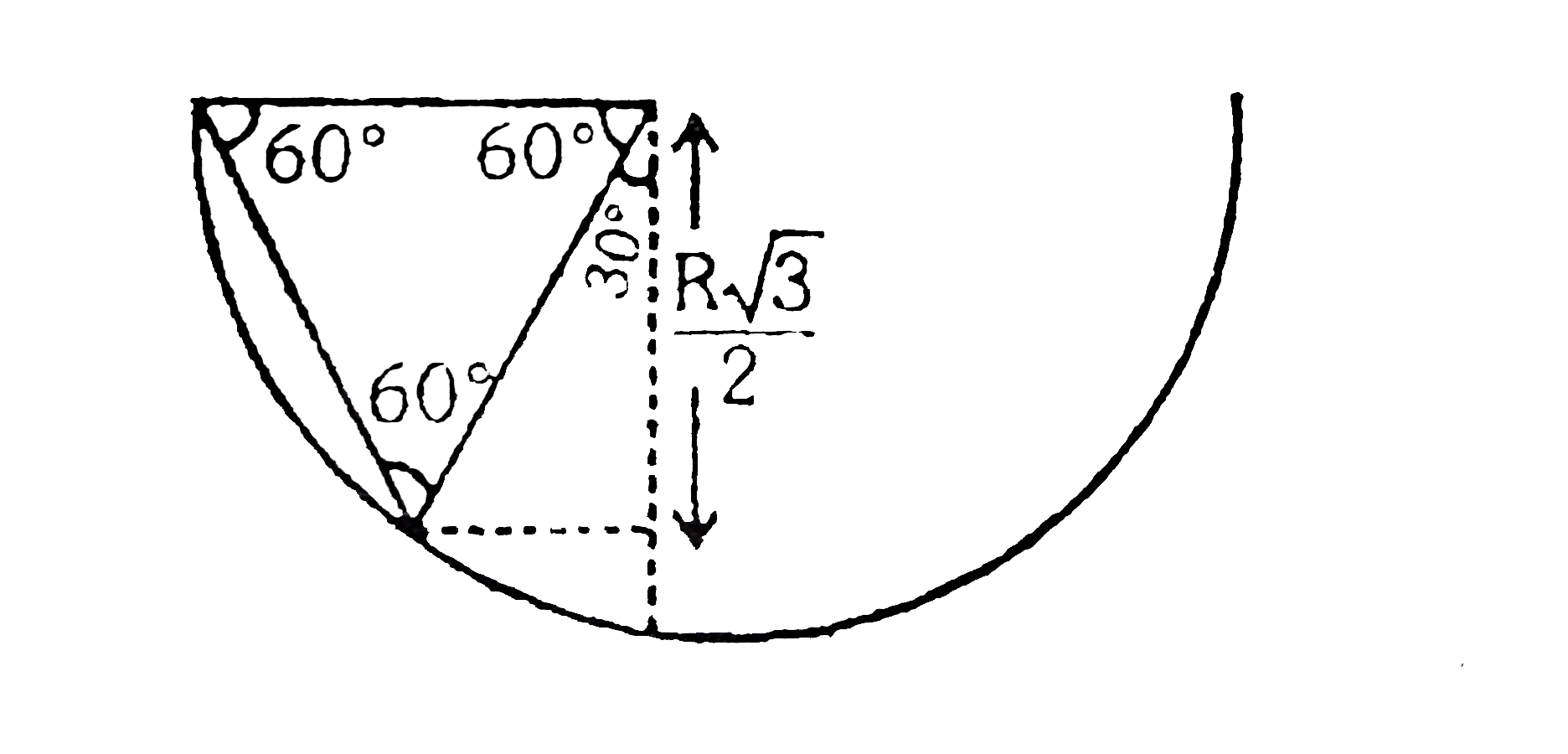
Time for this =
⎷2×R√32g=√√3Rg
→v(t)=uˆi+gtˆj=uˆi+g×√√3Rgˆj=uˆi+√√3Rgˆj
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-04[B]
14 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#7
3 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Knowledge Check
The displacement-time graph of moving particle is shown below
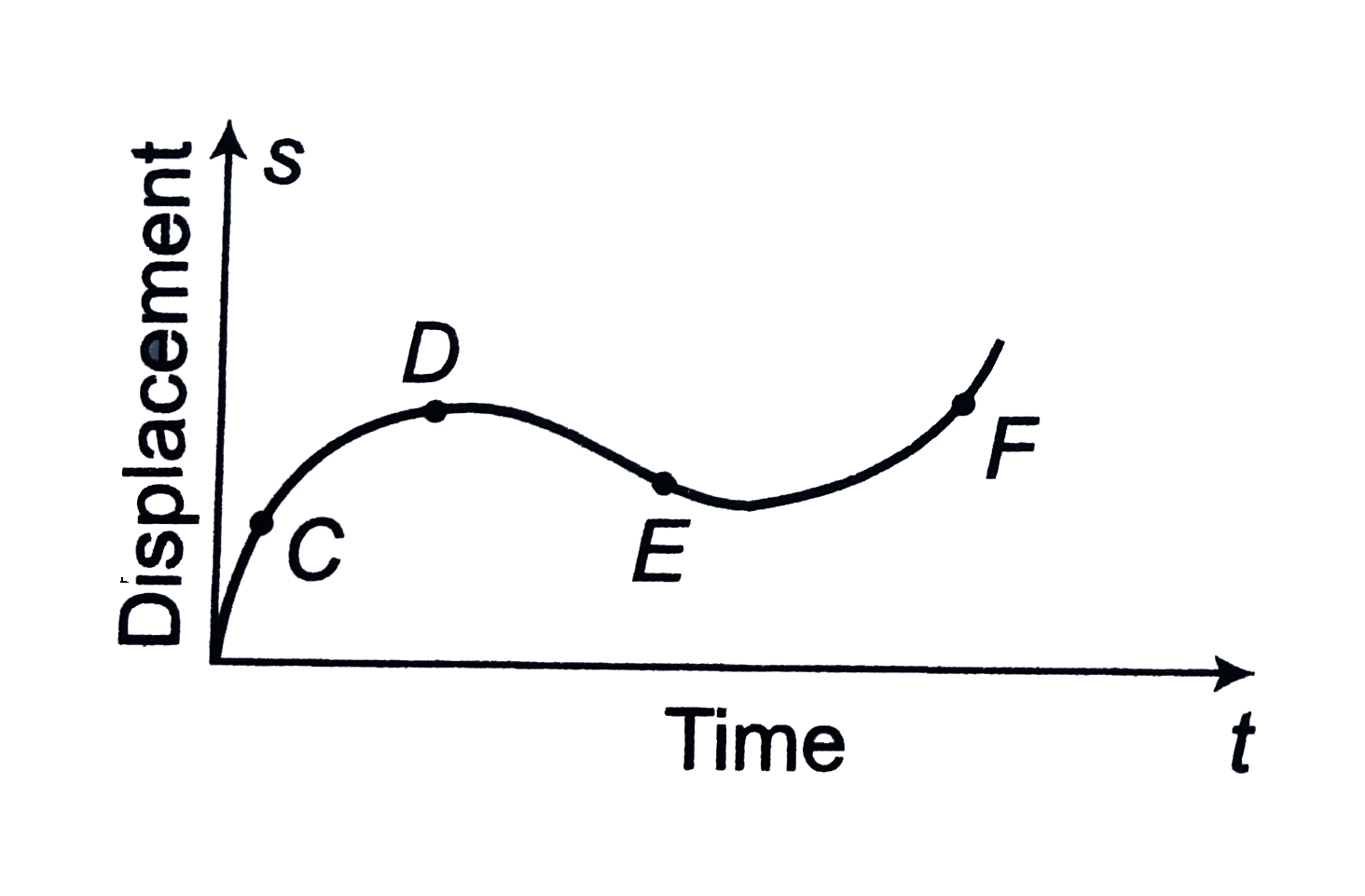
The instantaneous velocity of the particle in negative at the pointACBDCEDF
The displacement time graph of moving particle is shown below.
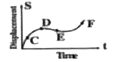
The instantaneous velocity of the particle is negative at the point.
View SolutionThe displacement time graph of a moving particle is shown below
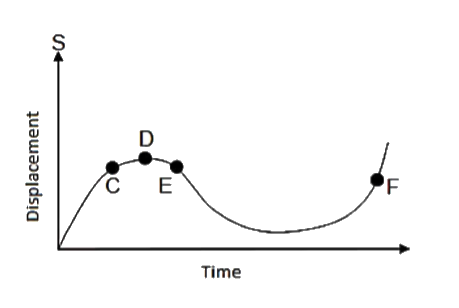
The instantaneous velocity of the particle is negative at the point
View SolutionThe displacement of a particle as a function of time is shown in . It indicates
 .
.
View SolutionThe position- time graph of a moving particle is shown. The instantaneous velocity of the particle is negative at the point
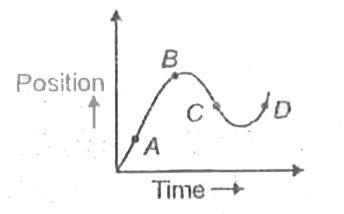
View SolutionThe displacement-time graph of a particle is as shown below. It indicates that
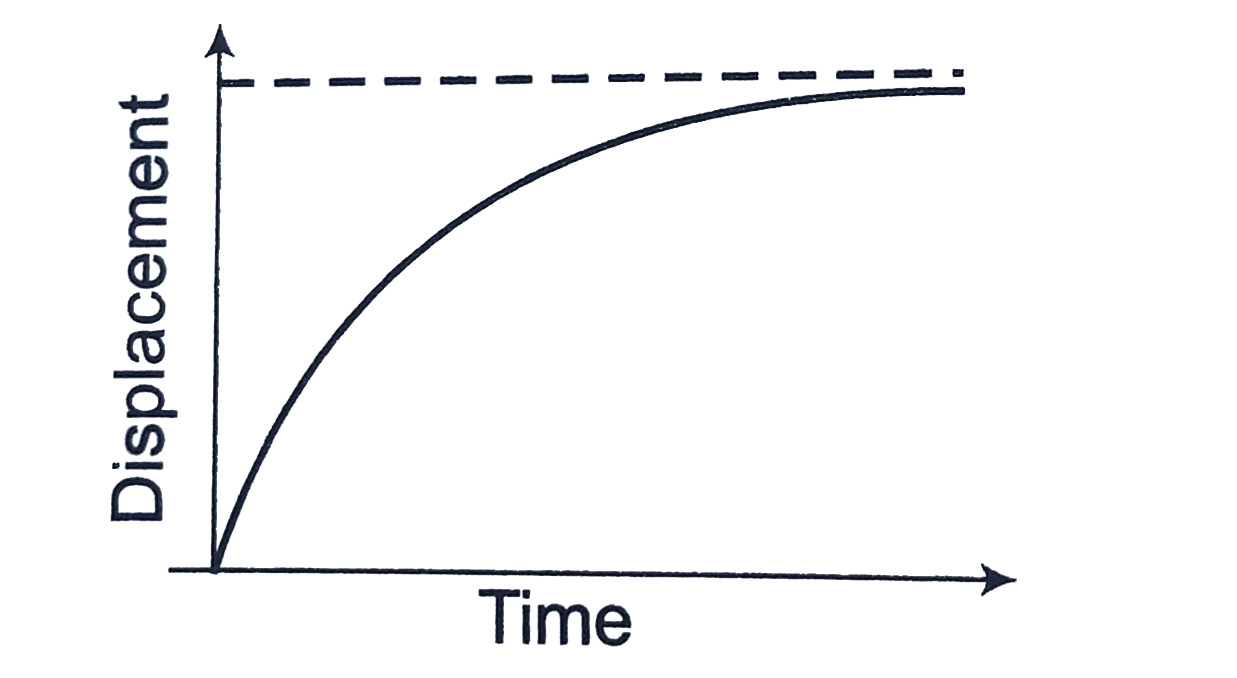
View SolutionA particle is projected horizontally from the top of a tower with a velocity v0. If v be its velocity at any instant, then the radius of curvature of the path of the particle at that instant is directely proportional to
View SolutionThe displacement of a particle starts from rest is proportional to the square of time, then the particle travels with
View Solution
