Particle is dropped form the height of 20m from horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
6m
10m
12m
24m
The correct Answer is:C
To solve the problem of finding the horizontal displacement of a particle dropped from a height of 20 meters with a horizontal acceleration of 6 m/s², we can follow these steps:
1. Determine the time taken to fall:
The time t taken for the particle to fall from a height h can be calculated using the formula:
t=√2hg
where g is the acceleration due to gravity (approximately 10m/s2).
Given:
- Height h=20m
- g=10m/s2
Substituting the values:
t=√2×2010=√4=2s
2. Calculate the horizontal displacement:
The horizontal displacement R can be calculated using the formula:
R=vx⋅t+12axt2
where:
- vx is the initial horizontal velocity (which is 0 since the particle is dropped),
- ax is the horizontal acceleration (given as 6m/s2),
- t is the time calculated in the previous step.
Since vx=0:
R=0⋅t+12⋅6⋅(2)2
Simplifying this:
R=0+12⋅6⋅4=12m
3. Final Result:
The horizontal displacement of the particle until it reaches the ground is 12m.
Summary:
The horizontal displacement of the particle is 12m.
The time t taken for the particle to fall from a height h can be calculated using the formula:
t=√2hg
where g is the acceleration due to gravity (approximately 10m/s2).
- Height h=20m
- g=10m/s2
t=√2×2010=√4=2s
The horizontal displacement R can be calculated using the formula:
R=vx⋅t+12axt2
where:
- vx is the initial horizontal velocity (which is 0 since the particle is dropped),
- ax is the horizontal acceleration (given as 6m/s2),
- t is the time calculated in the previous step.
R=0⋅t+12⋅6⋅(2)2
Simplifying this:
R=0+12⋅6⋅4=12m
The horizontal displacement of the particle until it reaches the ground is 12m.
The horizontal displacement of the particle is 12m.
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-02
57 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-03
6 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-2
89 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A stone is dropped from a height of 45 m on a horizontal level ground. There is horizontal wind blowing due to which horizontal acceleration of the stone becomes 10m/s2. (Take g=10m/s2). The time taken (t) by stone to reach the ground and the net horizontal displacement (x) of the stone form the time it is dropped an till it reaches the ground are respectively
View SolutionA ball is dropped from a height of 49 m. The wind is blowing horizontally. Due to wind a constant horizontal acceleration is provided to the ball. Choose the correct statement (s).
View SolutionA ball is dropped from a height of 49 m. The wind is blowing horizontally. Due to wind a constant horizontal acceleration is provided to the ball. Choose the correct statement (s).
View SolutionA particle is released from a certain height H=400m. Due to the wind, the particle gathers the horizontal velocity component vx=aywhere a =(√5)s−1 and y is the vertical displacement of the particle from the point of release, then find
(a) the horizontal drift of the particle when it strikes the ground ,
(b) the speed with which particle strikes the ground.
View SolutionIn the figure shown, find
(a) the time when the particle strikes the ground at P.
(b) the horizontal distance QP
(c) velocity of the particle at P.
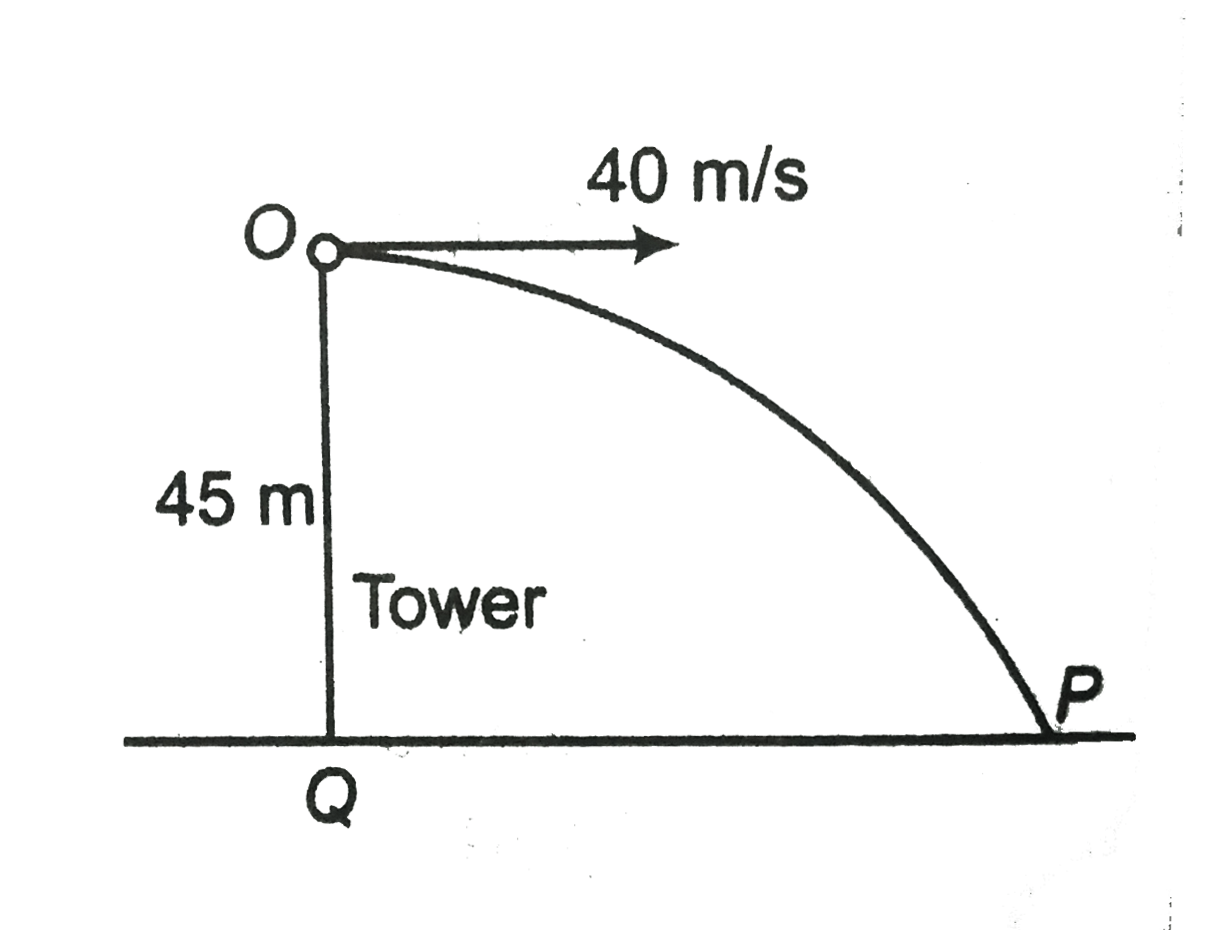
View SolutionA particle with a velcoity (u) so that its horizontal ange is twice the greatest height attained. Find the horizontal range of it.
View SolutionA particle is projected horizontally with a speed v0 at t = 0 from a certain point above the ground . What is the magnitude of tangential acceleration of the particle at t=t1 is ( Assume that the particle does not strike the ground between the time interval t = 0 to t=t1 )
View SolutionFrom the ground, a projectile is fired at an angle of 60 degree to the horizontal with a speed of 20 m s−1 The horizontal range of the projectile is
View SolutionA particle is dropped from a height h. Another particle which is initially at a horizontal distance d from the first is simultaneously projected with a horizontal velocity u and the two particles just collide on the ground. Then
View SolutionA particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.

View Solution
