Two stones A and B are projected simultaneously as shown in figure. It has been observed that both the stones reach the ground at the same place after 7 sec of their projection. Determine difference in their vertical components of initial velocities in m/s. (g=9.8m/s2)
The correct Answer is:7
To solve the problem, we need to determine the difference in the vertical components of the initial velocities of the two stones A and B, given that they reach the ground at the same place after 7 seconds and that the height difference between their launch points is 49 meters.
1. Identify the Variables:
- Let u1 be the initial velocity of stone A.
- Let u2 be the initial velocity of stone B.
- Let θ1 be the angle of projection for stone A.
- Let θ2 be the angle of projection for stone B.
- The vertical component of the initial velocity for stone A is u1sinθ1.
- The vertical component of the initial velocity for stone B is u2sinθ2.
2. Determine the Displacement:
- The vertical displacement for stone A after 7 seconds is 0 (it returns to the same height).
- The vertical displacement for stone B after 7 seconds is -49 meters (it falls 49 meters).
3. Use the Equation of Motion:
- For stone A:
yA=u1sinθ1⋅t−12gt2
Since yA=0 at t=7 seconds:
0=u1sinθ1⋅7−12⋅9.8⋅(7)2
Rearranging gives:
u1sinθ1=12⋅9.8⋅7=34.3m/s
- For stone B:
yB=u2sinθ2⋅t−12gt2
Since yB=−49 meters at t=7 seconds:
−49=u2sinθ2⋅7−12⋅9.8⋅(7)2
Rearranging gives:
u2sinθ2=12⋅9.8⋅7−497
Simplifying:
u2sinθ2=34.3+7=41.3m/s
4. Calculate the Difference:
- The difference in the vertical components of the initial velocities is:
Δu=u2sinθ2−u1sinθ1
Substituting the values:
Δu=41.3−34.3=7m/s
Final Answer:
The difference in their vertical components of initial velocities is 7m/s.
---
- Let u1 be the initial velocity of stone A.
- Let u2 be the initial velocity of stone B.
- Let θ1 be the angle of projection for stone A.
- Let θ2 be the angle of projection for stone B.
- The vertical component of the initial velocity for stone A is u1sinθ1.
- The vertical component of the initial velocity for stone B is u2sinθ2.
- The vertical displacement for stone A after 7 seconds is 0 (it returns to the same height).
- The vertical displacement for stone B after 7 seconds is -49 meters (it falls 49 meters).
- For stone A:
yA=u1sinθ1⋅t−12gt2
Since yA=0 at t=7 seconds:
0=u1sinθ1⋅7−12⋅9.8⋅(7)2
Rearranging gives:
u1sinθ1=12⋅9.8⋅7=34.3m/s
yB=u2sinθ2⋅t−12gt2
Since yB=−49 meters at t=7 seconds:
−49=u2sinθ2⋅7−12⋅9.8⋅(7)2
Rearranging gives:
u2sinθ2=12⋅9.8⋅7−497
Simplifying:
u2sinθ2=34.3+7=41.3m/s
- The difference in the vertical components of the initial velocities is:
Δu=u2sinθ2−u1sinθ1
Substituting the values:
Δu=41.3−34.3=7m/s
The difference in their vertical components of initial velocities is 7m/s.
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-01
55 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-02
57 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Two particles of equal mass are projected simultaneously with speeds 20m/s end 10√3m/s as shown in figure. Find the maximum height reached by the centre of mass of the particles
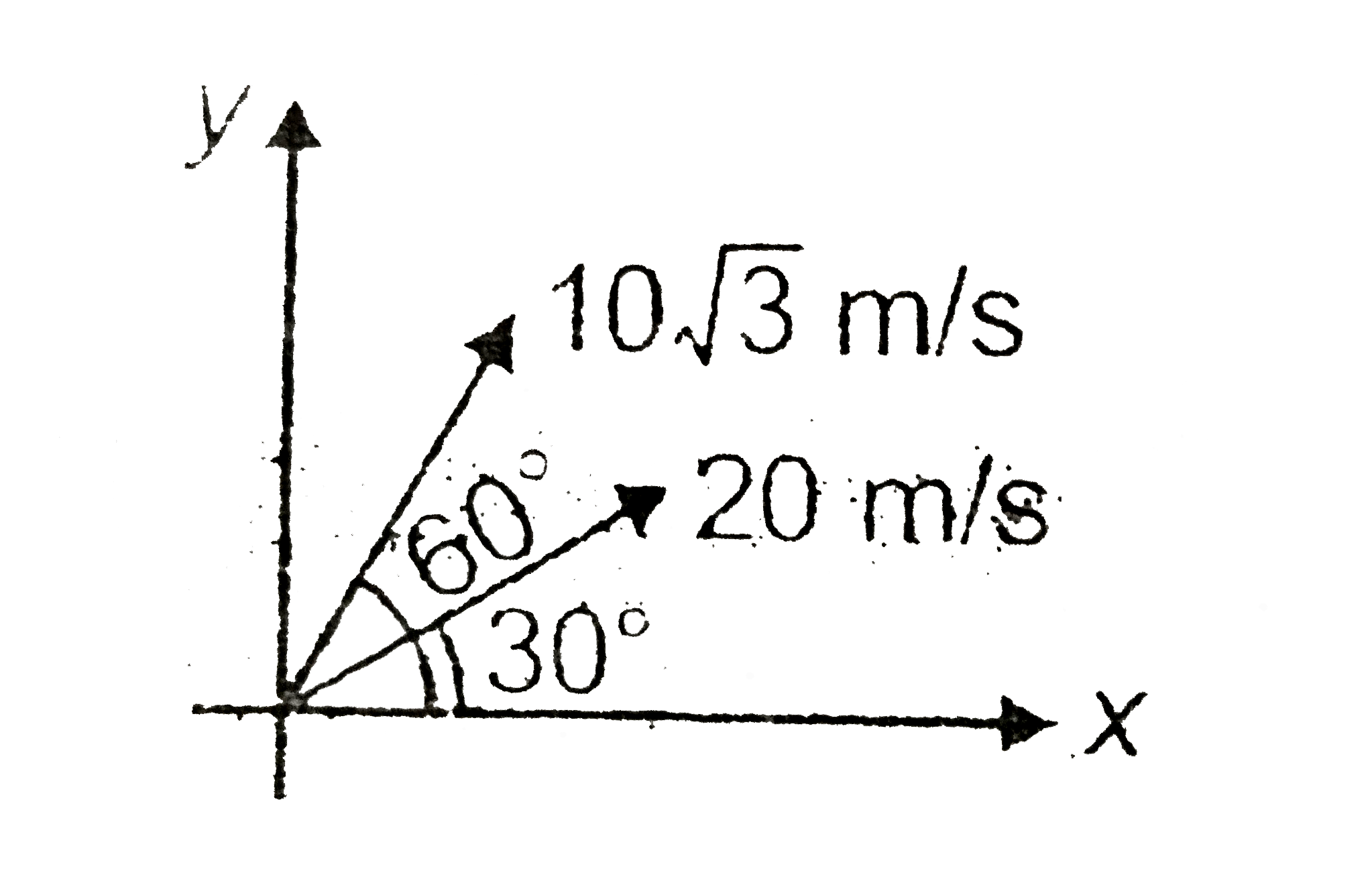
View SolutionTwo balls A and B are projected from the same location simultaneously. Ball A is projected vertically upwards and ball B at 30∘
to the vertical. They reach the ground simultaneously. The velocities of projection of A and B are in the ratio
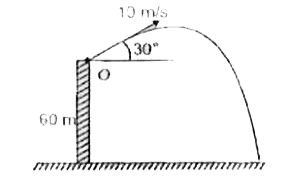
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
View SolutionTwo balls are dropped from different heights at different instants. Seconds ball is dropped 2 sec. after the first ball. If both balls reach the ground simultaneousl after 5 sec. of dropping the first ball. then the difference of initial heights of the two balls will be :- (g=9.8m/s2)
View SolutionTwo particles are projected with speed 4m/s and 3m/s simultaneously from same point as shown in the figure. Then:

View SolutionA body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its initial velocity vector is- (g=10 m/s2)
View SolutionA body is projected horizontally from the top of a tower with a velocity of 10m/s.If it hits the ground at an angle 45∘, then vertical component of velocity when it hits ground in m/s is
View SolutionTwo particles A and B are projected simultaneously in the directins shown in figure with velocities vA=20m/sandvB=10m/s respectively. They collide in air after 12 s. Find
(a) the angle θ
(b) the distance x.
 .
.
View SolutionA body is projected horizontally from the top of a tower with a velocity of 20 m/s. After what time the vertical component of velocity is four times the horizontal component of velocity ? (g = 10m/s2)
View SolutionTwo stones AandB are projected simultaneously from the top of a 100−m high tower. Stone B is projected horizontally with speed 10ms−1, and stone A is droppd from the tower. Find out the following :
(a) Time of flight of the two stone
(b) Distance between two stones after 3s
(c ) Angle of strike with ground
(d) Horizontal range of particle B.
View Solution
