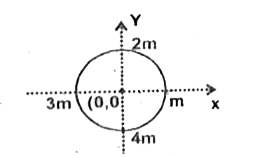
In the given figure four rods $A B, B C, C D$ and $D A$ have mass $m$ , $2 m$ , $3 m$ and $4 m$ respectively In Which of the regions (numberd $1, 2, 3, 4$ ) the centre of mass of system lies?

A

$1$

B

$2$

C

$3$

D

$4$

Video Solution

Text Solution

Verified by Experts

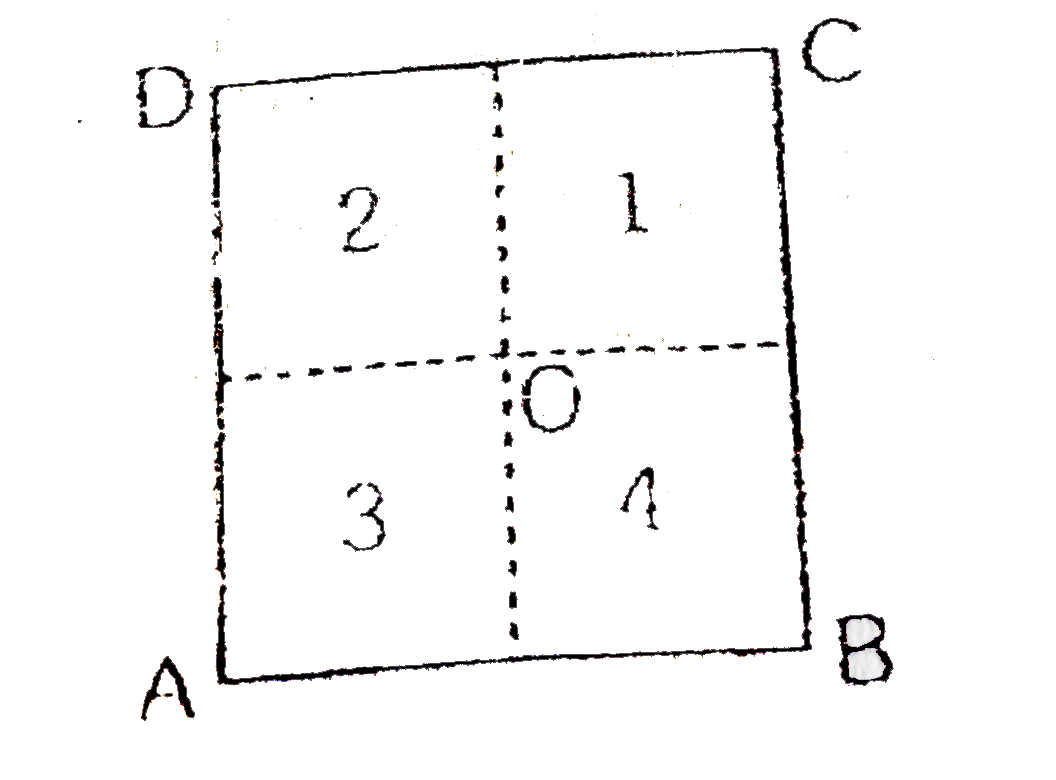
The correct Answer is:B

Ans. (2)

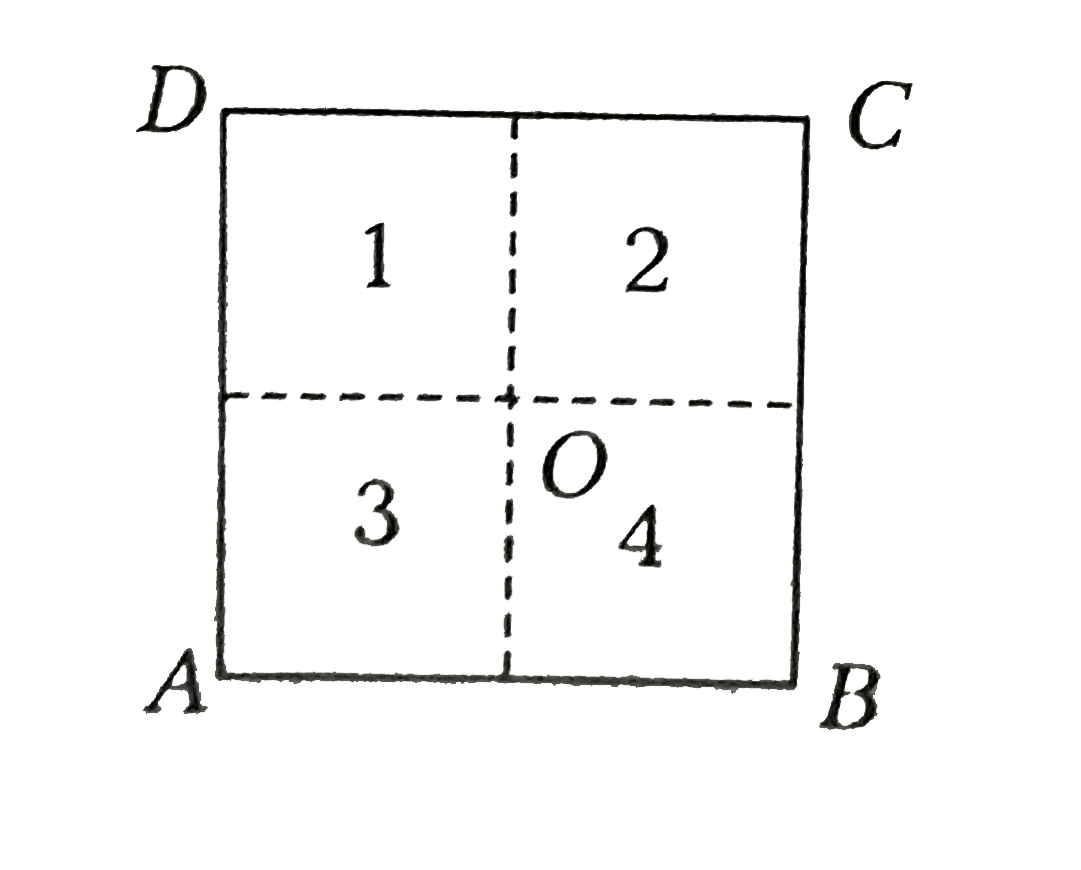
$\Rightarrow$ centre of mass lies in region $2$ .

Updated on:21/07/2023

|

Class 12 PHYSICS TEST PAPER

Topper's Solved these Questions

Similar Questions

Four rods AB, BC, CD and DA have mass m,2m,3m and 4m respectively. The center of mass of all the four rods

View Solution
Two homogeneous spheres A and B of masses m and 2m having radii 2a and a respectively are placed in touch . The distance of the centre of mass from the first sphere is
View Solution
Two bodies of mass 2 kg and 5 kg have position (1 m, 2 m, 1 m) and (3 m, 2 m, -1 m) respectively. The position vector of centre of mass is
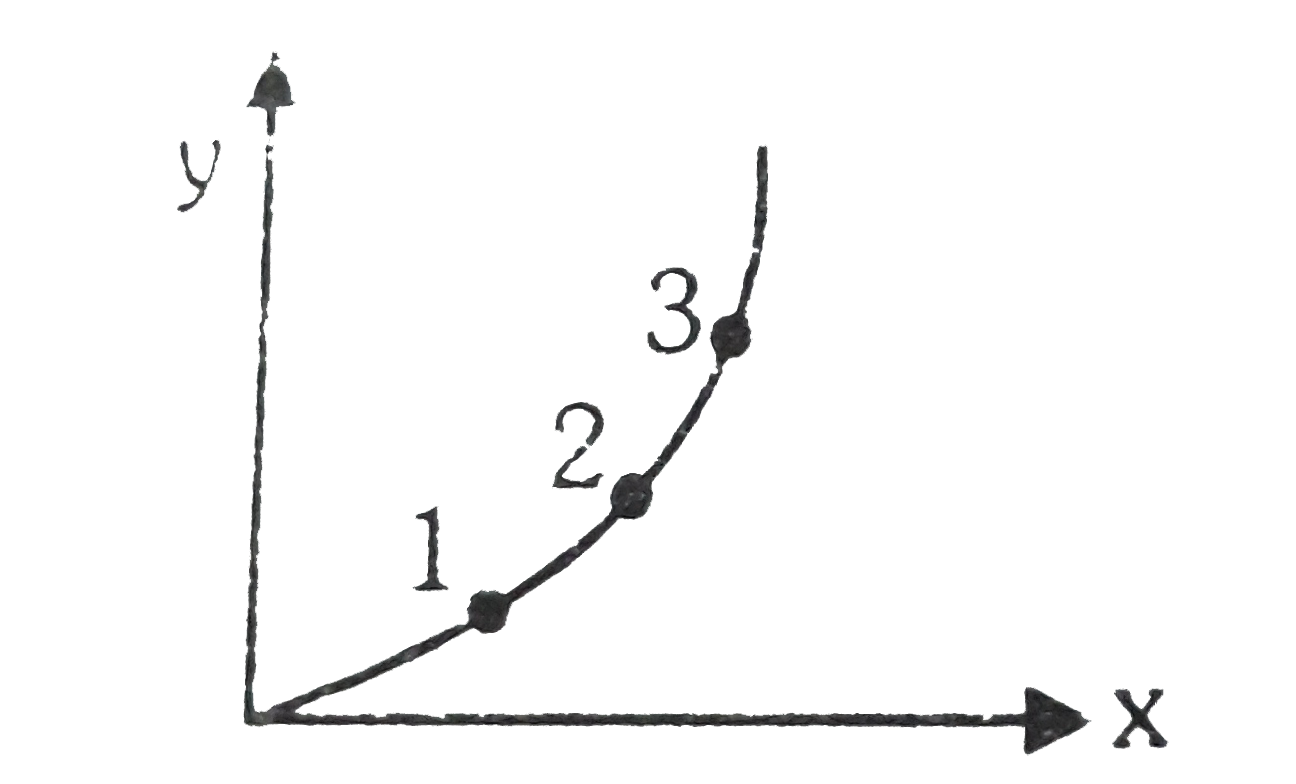
View Solution
The slope of graph as shown in figure at points 1,2 and 3 is $m_{1}, m_{2}$ and $m_{3}$ respectively then

View Solution
Four particles of masses m, 2m, 3m & 4m respectively are placed on the periphery of a circle of radius R as shown in the figure then CM of the system contains the particle is at

View Solution
Two identical thin rings each of radius R are coaxially placed at a distance R. If the rings have a uniform mass distribution and each has masses $2 m$ and $4 m$ respectively, then the work done in moving a mass $m$ from centre of one ring to that of the other is
View Solution
Three particles of masses $m$ , $m$ and $4 k g$ are kept at a verticals of triangle $A B C$ . Coordinates of $A$ , $B$ and $C$ are $(1, 2)$ , $(3, 2)$ and $(- 2, - 2)$ respectively such that the centre of mass lies at origin. Find the value of mass $m$ .
Hint. $x_{c m} = \frac{\sum_{i - 1}^{3} m_{i} x_{i}}{\sum_{i = 1}^{3} m_{i}}$ , $y_{c m} = \frac{\sum_{i = 1}^{3} m_{i} y_{i}}{\sum_{i = 1}^{3} m_{i}}$
View Solution
Three particles of masses $m$ , $m$ and $4 k g$ are kept at a verticals of triangle $A B C$ . Coordinates of $A$ , $B$ and $C$ are $(1, 2)$ , $(3, 2)$ and $(- 2, - 2)$ respectively such that the centre of mass lies at origin. Find the value of mass $m$ .
Hint. $x_{c m} = \frac{\sum_{i - 1}^{3} m_{i} x_{i}}{\sum_{i = 1}^{3} m_{i}}$ , $y_{c m} = \frac{\sum_{i = 1}^{3} m_{i} y_{i}}{\sum_{i = 1}^{3} m_{i}}$
View Solution
Three point like equal masses $m_{1}, m_{2}$ and $m_{3}$ are connected to the ends of a massless rod of length $L$ which lies at rest on a smooth horizontal plane. At $t = 0$ , an explosion occurs between $m_{2}$ and $m_{3}$ , and as a result, mass $m_{3}$ is detached from the rod, and moves with a known velocity v at an angle of $30^{\circ}$ with the $y$ -axis. Assume that the masses $m_{2}$ and $m_{3}$ are unchanged during the explosion. What is the velocity of the centre of mass of the system consisting of three masses, after the expulsion?

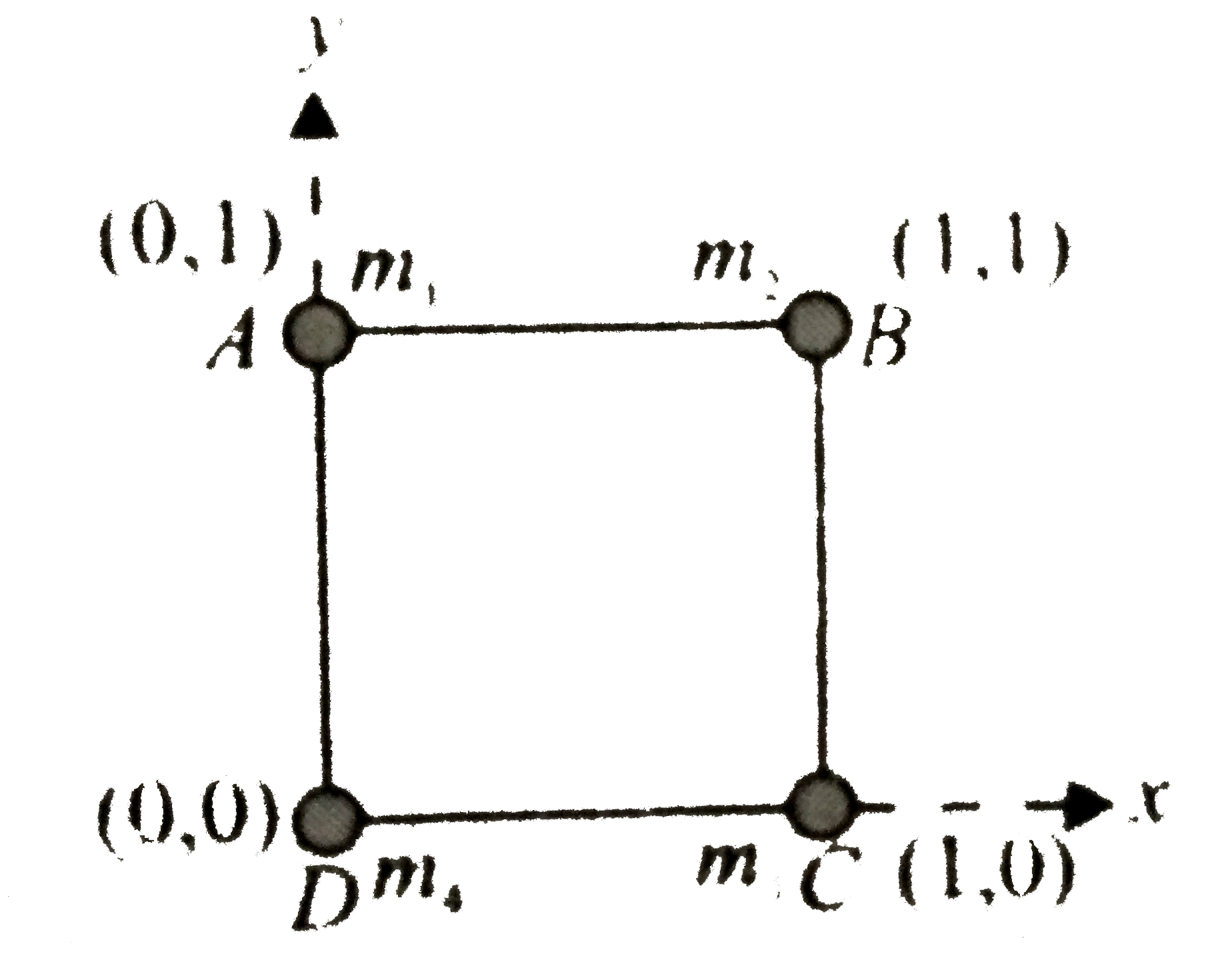
View Solution
Four particles of masses $1 k g, 2 k g, 3 k g$ and $4 k g$ are placed the four vertices $A, B, C$ and $D$ , respectively, of a square of side $1 m$ . Find the position of centre of mass of the particles.

View Solution

ALLEN-TEST PAPER-Exercise (Physics)

Home
Ask Doubt
Profile