In a second ODI match between England and India Bhuwnesh kumar bowled his medium fast bowling. He starts his run up from the distance of 50m from the stumps (bowling end) and at the time of bowling a ball,his speed is 36(km)/(hr) and the speed of ball is 144(km)/(hr).
If his mass is 70kg and mass of the ball is 14kg and the heat produce in his body is one tenth of the total work done by him, then
If he accelerates uniformly to the sumps find the average friction force acting on him :−
750 N
70.25 N
90.25 N
70 N
The correct Answer is:B
To solve the problem step by step, we will follow the principles of physics related to work, energy, and friction.
Step 1: Convert Speeds to Meters per Second
First, we need to convert the speeds from kilometers per hour to meters per second.
- Bhuvnesh's speed:
36 km/hr=36×10003600=10 m/s
- Ball's speed:
144 km/hr=144×10003600=40 m/s
Step 2: Calculate the Change in Kinetic Energy
Next, we need to calculate the change in kinetic energy of Bhuvnesh as he runs towards the stumps.
- Initial kinetic energy (KE_initial) = 0 (he starts from rest)
- Final kinetic energy (KE_final) = 12mv2
where m is the total mass (Bhuvnesh + ball) and v is his final speed.
- Total mass:
m=70 kg+14 kg=70.25 kg
- Final kinetic energy:
KEfinal=12×70.25×(10)2=12×70.25×100=3512.5 J
Step 3: Calculate the Work Done by Friction
According to the work-energy principle, the work done by the friction force is equal to the change in kinetic energy.
- Work done by friction (W) = Change in KE = KE_final - KE_initial = 3512.5 J−0=3512.5 J
Step 4: Relate Work Done to Friction Force
The work done by the friction force can also be expressed as:
W=Ffriction×d
where d is the distance traveled (50 m).
Rearranging gives:
Ffriction=Wd=3512.550=70.25 N
Step 5: Conclusion
The average friction force acting on Bhuvnesh is:
Ffriction=70.25 N
First, we need to convert the speeds from kilometers per hour to meters per second.
36 km/hr=36×10003600=10 m/s
144 km/hr=144×10003600=40 m/s
Next, we need to calculate the change in kinetic energy of Bhuvnesh as he runs towards the stumps.
- Final kinetic energy (KE_final) = 12mv2
where m is the total mass (Bhuvnesh + ball) and v is his final speed.
m=70 kg+14 kg=70.25 kg
KEfinal=12×70.25×(10)2=12×70.25×100=3512.5 J
According to the work-energy principle, the work done by the friction force is equal to the change in kinetic energy.
The work done by the friction force can also be expressed as:
W=Ffriction×d
where d is the distance traveled (50 m).
Ffriction=Wd=3512.550=70.25 N
The average friction force acting on Bhuvnesh is:
Ffriction=70.25 N
Topper's Solved these Questions
Similar Questions
In a second ODI match between England and India Bhuwnesh kumar bowled his medium fast bowling. He starts his run up from the distance of 50m from the stumps (bowling end) and at the time of bowling a ball,his speed is 36(km)/(hr) and the speed of ball is 144(km)/(hr).
If his mass is 70kg and mass of the ball is 14kg and the heat produce in his body is one tenth of the total work done by him, then
Select the incorrect statement :View SolutionIn a second ODI match between England and India Bhuwnesh kumar bowled his medium fast bowling. He starts his run up from the distance of 50m from the stumps (bowling end) and at the time of bowling a ball,his speed is 36(km)/(hr) and the speed of ball is 144(km)/(hr).
If his mass is 70kg and mass of the ball is 14kg and the heat produce in his body is one tenth of the total work done by him, then
Energy spent by bowler in throwing one ball is :−View SolutionA man goes 40m due north and then 50m due west. Find his distance from the starting point.
View SolutionRam covers a distance of 100 km with variable speed. During the first half of his journey his speed was 20 km/h and during the rest of his journey his speed was 40 km/h. Calculate the average speed of Ram during the whole course of his motion.
View SolutionA man starting from point P cross a 4km wide lagoon and reaches point Q in the shortest possible time by the path shown in the figure. If the person swims at a speed of 3km//hr and walks at a speed of 4km//hr, then find his time fo journey (in minutes).
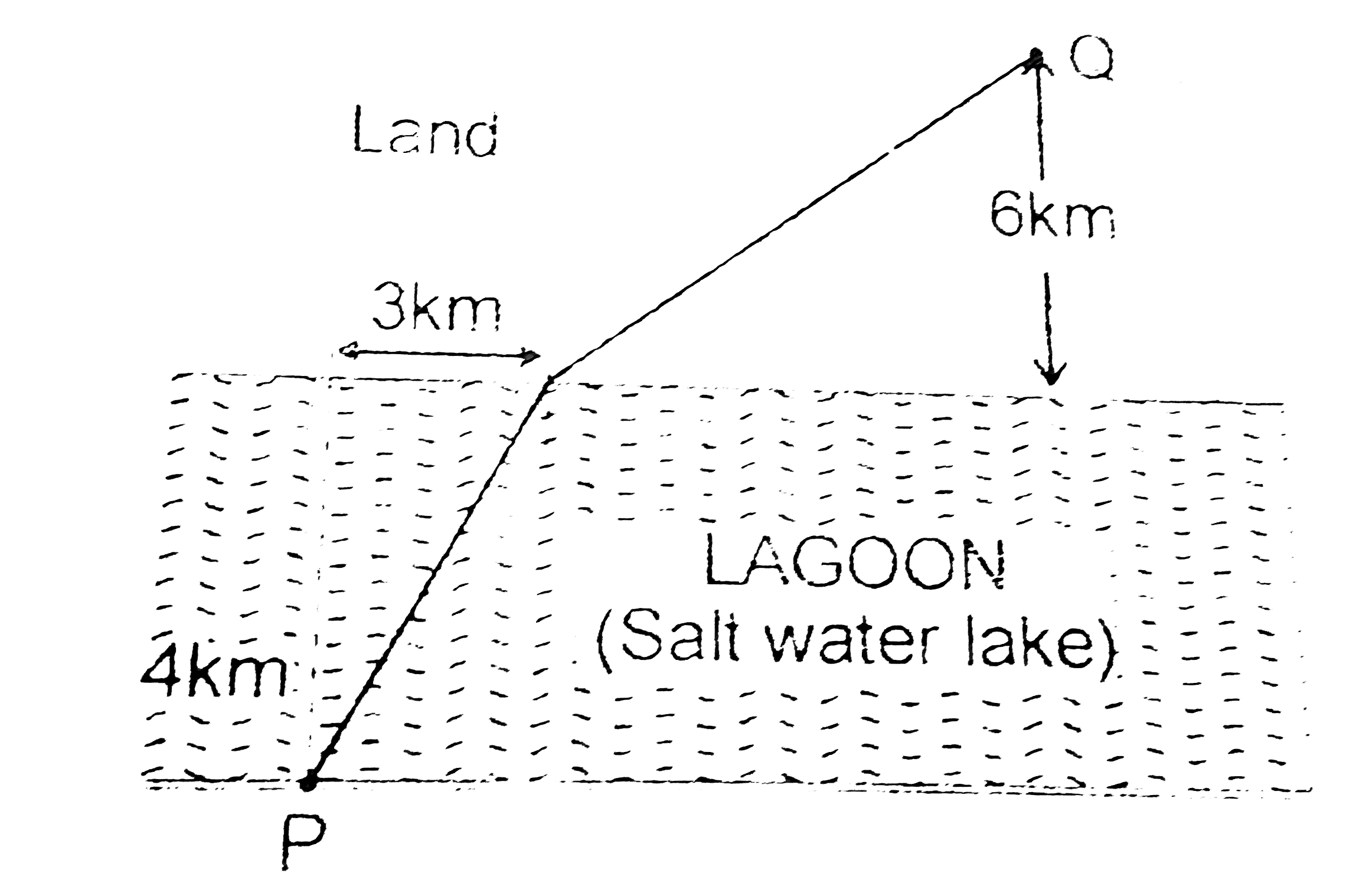 .View Solution
.View SolutionA man drives his car uniformly at 30 km/h for three hour After it he covered next 90 km distance with uniform speed of 45 km/hr. Find the average speed of car.
View SolutionA boy walks from his home to a post office at a distance of 0.5 km in 20 min. He then returns to his home in time 25 min. Find the average speed of the boy.
View SolutionIf the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
View SolutionIf the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
View SolutionA car travels the first half of a distance between two places a speed of 30 km/hr and the second half of the distance a 50 km/hr. The average speed of the car for the whole journey is
View Solution
