In a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by , the kinetic energy of a shot moving with velocity metres per second is denoted by , and its momentum by 10.
the unit of mass is
544.2 kg
54.42 kg
5442 kg
None of these
The correct Answer is:A
To find the unit of mass in the given system, we will follow these steps:
Step 1: Understand the given quantities
We know:
- The acceleration due to gravity is denoted by 3.
- The kinetic energy of a shot moving with velocity 448ms is denoted by 100.
- The momentum of the shot is denoted by 10.
- The mass of the shot in the conventional system is 272.1kg.
Step 2: Write the equations for kinetic energy and momentum
1. Kinetic Energy (KE) is given by the formula:
\(
KE = \frac{1}{2} m v^2
\)
In the new system, this is denoted as:
\(
\frac{1}{2} m' v'^2 = 100 \quad \text{(Equation 1)}
\)
2. Momentum (p) is given by the formula:
\(
p = mv
\)
In the new system, this is denoted as:
\(
m' v' = 10 \quad \text{(Equation 2)}
\)
Step 3: Solve for \( v' \)
From Equation 2, we can express \( v' \):
\(
v' = \frac{10}{m'}
\)
Step 4: Substitute \( v' \) into the kinetic energy equation
Substituting \( v' \) from Equation 2 into Equation 1:
\(
\frac{1}{2} m' \left(\frac{10}{m'}\right)^2 = 100
\)
This simplifies to:
\(
\frac{1}{2} m' \cdot \frac{100}{(m')^2} = 100
\)
\(
\frac{50}{m'} = 100
\)
Now, solving for \( m' \):
\(
m' = \frac{50}{100} = 0.5
\)
Step 5: Relate \( m' \) to the conventional mass
From the problem, we know that the mass of the shot in the conventional system is \( 272.1 kg \). Therefore, we can set up the relationship:
\(
m' = 0.5 \times m_{unit}
\)
Where \( m_{unit} \) is the unit of mass in the new system.
Step 6: Solve for the unit of mass
We can express this as:
\(
0.5 \times m_{unit} = 272.1
\)
Thus,
\(
m_{unit} = \frac{272.1}{0.5} = 544.2
\)
Conclusion
The unit of mass in the given system is:
\(
\text{Unit of mass} = 544.2 \text{ kg}
\)
We know:
- The acceleration due to gravity is denoted by 3.
- The kinetic energy of a shot moving with velocity 448ms is denoted by 100.
- The momentum of the shot is denoted by 10.
- The mass of the shot in the conventional system is 272.1kg.
1. Kinetic Energy (KE) is given by the formula:
\(
KE = \frac{1}{2} m v^2
\)
In the new system, this is denoted as:
\(
\frac{1}{2} m' v'^2 = 100 \quad \text{(Equation 1)}
\)
\(
p = mv
\)
In the new system, this is denoted as:
\(
m' v' = 10 \quad \text{(Equation 2)}
\)
From Equation 2, we can express \( v' \):
\(
v' = \frac{10}{m'}
\)
Substituting \( v' \) from Equation 2 into Equation 1:
\(
\frac{1}{2} m' \left(\frac{10}{m'}\right)^2 = 100
\)
This simplifies to:
\(
\frac{1}{2} m' \cdot \frac{100}{(m')^2} = 100
\)
\(
\frac{50}{m'} = 100
\)
Now, solving for \( m' \):
\(
m' = \frac{50}{100} = 0.5
\)
From the problem, we know that the mass of the shot in the conventional system is \( 272.1 kg \). Therefore, we can set up the relationship:
\(
m' = 0.5 \times m_{unit}
\)
Where \( m_{unit} \) is the unit of mass in the new system.
We can express this as:
\(
0.5 \times m_{unit} = 272.1
\)
Thus,
\(
m_{unit} = \frac{272.1}{0.5} = 544.2
\)
The unit of mass in the given system is:
\(
\text{Unit of mass} = 544.2 \text{ kg}
\)
Topper's Solved these Questions
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:DATA SUFFICIENCY QUESTIONS
Explore 3 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Exercise-04 [A]
Explore 28 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Comprehension 3
Explore 5 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-7
Explore 8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLENChapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:EXERCISE-IV
Explore 8 Videos
Similar Questions
In a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by 3, the kinetic energy of a 272.1kg shot moving with velocity 448 metres per second is denoted by 100, and its momentum by 10.
The unit of length is
View SolutionIn a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of mass is :-
View SolutionIn a certain system of absoulte units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of length is :-
View Solutionn a certain system of absoulte units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of time is :-
View SolutionFind the kinetic energy of a body of mass 1 kg moving with a uniform velocity of 10 ms−1
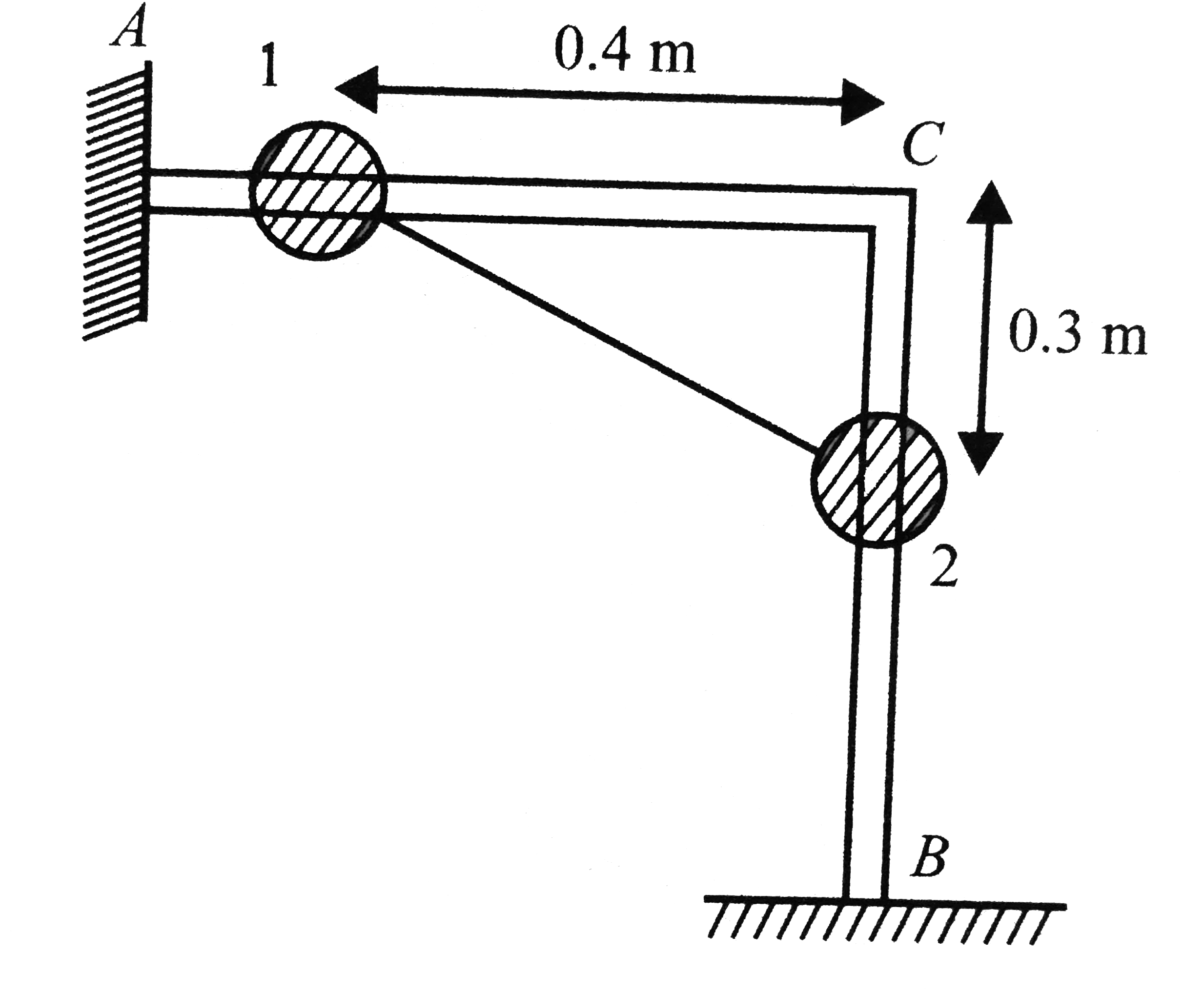
View SolutionTwo identical beads, each of m=100g, are connected by an inextensible massless string, which can slide along the two arms AC and BC of a rigid smooth wire frame in a vertical plane. If the system is released from rest, the kinetic energy of the first bead when the second bead has moved down by a distance of 0.1m is ×x10−3J. Find the value of x(g=10ms−2) (shown situation is after movement of 0.1m).
View SolutionA graph is plotted by taking transnational kinetic energy of body as ordinate and velocity as abscissa. Slope of the graph at an instant represents its
A. Velocity
B. Mass
C. Momentum
D. Kinetic energy pre unit mass
View Solution1 joule of energy is to be converted into new system of units in which length is measured in 10 metre, mass in 10 kg and time in 1 minute. The numerical value of 1 J in the new system is :-
View SolutionIn ancient time, deffernt system of units was following in which unit of mass was "ser", unit of length was "gaj" & unit of time was "kall" 1ser=900gm, 1gaj=90cm, 1kaal=3hr. A ball of mass 9kg is moving with velocity 10m/s. Then its momentum (mv) in ancient system will be :−
View SolutionThree spheres, each of mass m, can slide freely on a frictionless, horizontal surface. Spheres A and B are attached to an inextensible, inelastic cord of length l and are at rest in the position shown where sphere B is struck by sphere C which is moving to the right with a velocity v0. Knowing that the cord is taut where sphere B is struck by sphere C and assuming 'head on' inelastic impact between B and C, we cannot conserve kinetic energy of the entire system.
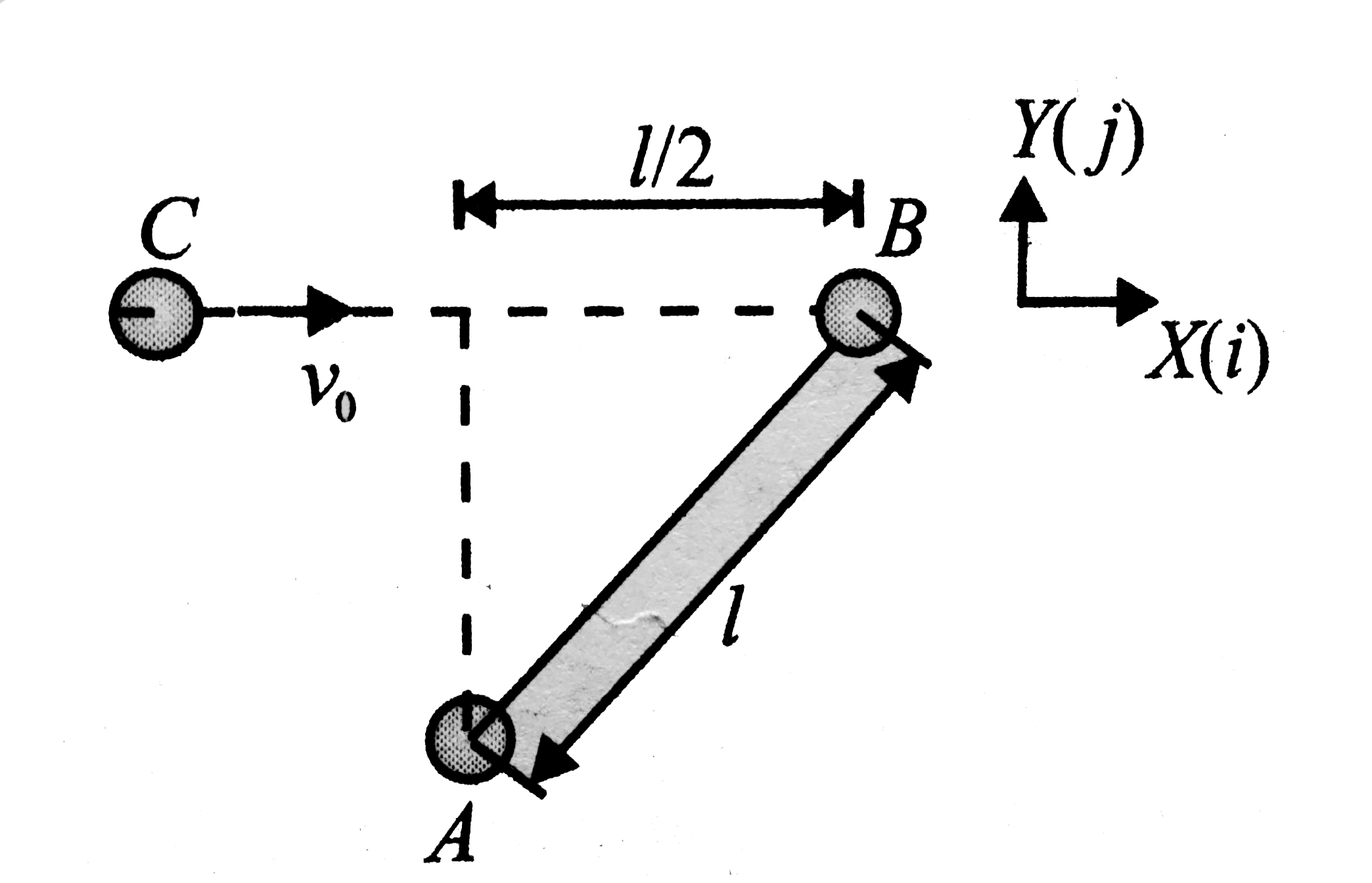 The velocity of B immediately after collision is along unit vector
The velocity of B immediately after collision is along unit vector
View Solution