It is easier to draw up a wooden block along an inclined plane than haul it up vertically because
a) The friction is reduced
b) The mass becomes smaller
c) g becomes smaller
d) Only a part of the weight has to be overcome
The correct Answer is:C
To solve the question, "It is easier to draw up a wooden block along an inclined plane than haul it up vertically because...", we can analyze the forces acting on the block in both scenarios.
1. Understanding the Forces:
- When a block of mass m is held vertically, the force required to lift it is equal to its weight, which is given by W=mg, where g is the acceleration due to gravity.
2. Analyzing the Inclined Plane:
- When the same block is drawn up an inclined plane at an angle θ, the weight of the block can be resolved into two components:
- One component acts perpendicular to the inclined plane.
- The other component acts parallel to the inclined plane, which is responsible for pulling the block down the slope.
3. Calculating the Parallel Component:
- The component of the weight acting parallel to the inclined plane is given by:
W∥=mgsin(θ)
- This means that when pulling the block up the inclined plane, you only need to overcome this parallel component of the weight.
4. Comparison of Forces:
- The force required to pull the block up the inclined plane is less than the force required to lift it vertically. This is because:
W∥=mgsin(θ)<mg
- Therefore, the force needed to draw the block up the inclined plane is less than the weight of the block.
5. Conclusion:
- Since only a part of the weight (the parallel component) needs to be overcome when pulling the block up the inclined plane, it is easier to draw it up this way compared to lifting it straight up vertically.
Final Answer:
The correct option is D: Only a part of the weight has to be overcome.
---
- When a block of mass m is held vertically, the force required to lift it is equal to its weight, which is given by W=mg, where g is the acceleration due to gravity.
- When the same block is drawn up an inclined plane at an angle θ, the weight of the block can be resolved into two components:
- One component acts perpendicular to the inclined plane.
- The other component acts parallel to the inclined plane, which is responsible for pulling the block down the slope.
- The component of the weight acting parallel to the inclined plane is given by:
W∥=mgsin(θ)
- This means that when pulling the block up the inclined plane, you only need to overcome this parallel component of the weight.
- The force required to pull the block up the inclined plane is less than the force required to lift it vertically. This is because:
W∥=mgsin(θ)<mg
- Therefore, the force needed to draw the block up the inclined plane is less than the weight of the block.
- Since only a part of the weight (the parallel component) needs to be overcome when pulling the block up the inclined plane, it is easier to draw it up this way compared to lifting it straight up vertically.
The correct option is D: Only a part of the weight has to be overcome.
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 A (Conceptual Subjective Exercise)
14 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 B (Brain Storming Subjective Exercise)
20 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 2 (Brain Teasers)
27 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
Knowledge Check
It is easier to liquify ammonia than oxygen because
A(a) it is easier to compress oxygen than NH3B(b) NH3 has a very low critical temperature as compared to O2C(c) O2 has a higher value of der Waals constant a and higher critical temperature than NH3D(d) NH3 has a higher value of van der Waals constant a and higher critical temperature than oxygen .
500 J of work is done in sliding a 4 kg block up along a rough inclined plane to a vertical height of 10 m slowly. The work done against friction is (g=10ms2)
View SolutionA weight w is to be moved from the bottom to the top of an inclined plane of inclination θ to the horizontal. If a smaller force is to be applied to drag it along the plane in comparison to lift it vertically up, the coefficient of friction should be such that.
View SolutionA block of mass m is moving down with constant velocity along an inclined plane of inclination θ. What is the work done by external force in pulling the block along the inclined plane through a height h with constant velocity?
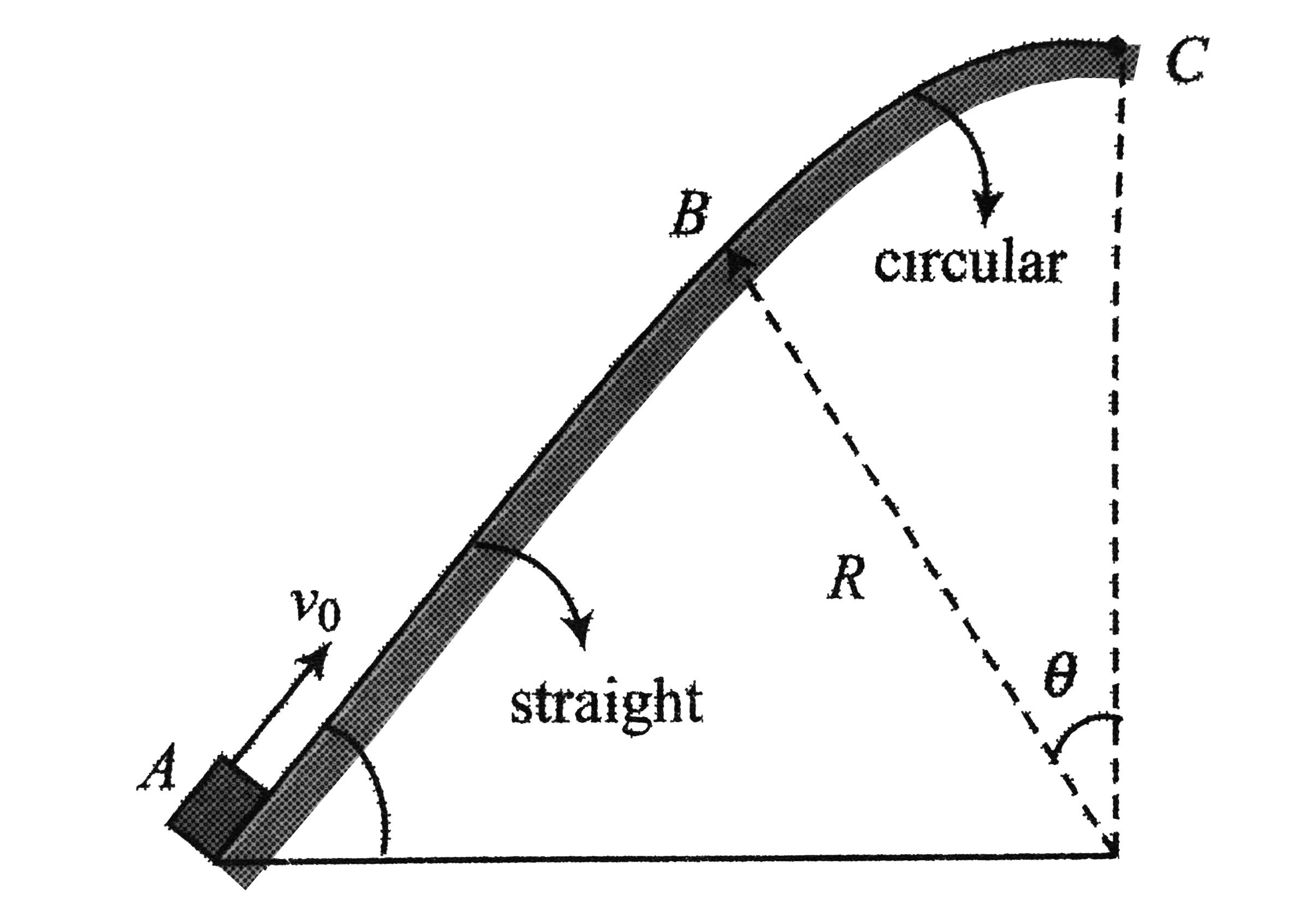
View SolutionA block of mass m is projected up with a velocity v0 along an inclined plane of angle of inclination θ=37∘. The coefficient of friction between the inclined plane AB and blocks is μ(=tanθ). Find the values of v0 so that the block moves in a circular path from B to C.
View SolutionSTATEMENT-1: The maixmum range along the inclined plane, when thrown downward is greater than that when thrown upward along the same inclined plane with constant velocity.
STATEMENT-2: The maximum range along inclined plane is independent of angle of inclination.
View SolutionIt is easier to pull a lawn roller than to push it because pulling
View SolutionA man of mass m speeds up while running from rest to a speed v in a straight track along an inclined plane, after raising through a height h.
Wgravity=work done by gravity on the man
Wiction=work done by friction on the man
Wman=work done by man
If instead of moving up the plane, the man increases his speed to the value v while moving down the inclined plane through the same vertical distance h, then
View Solution
