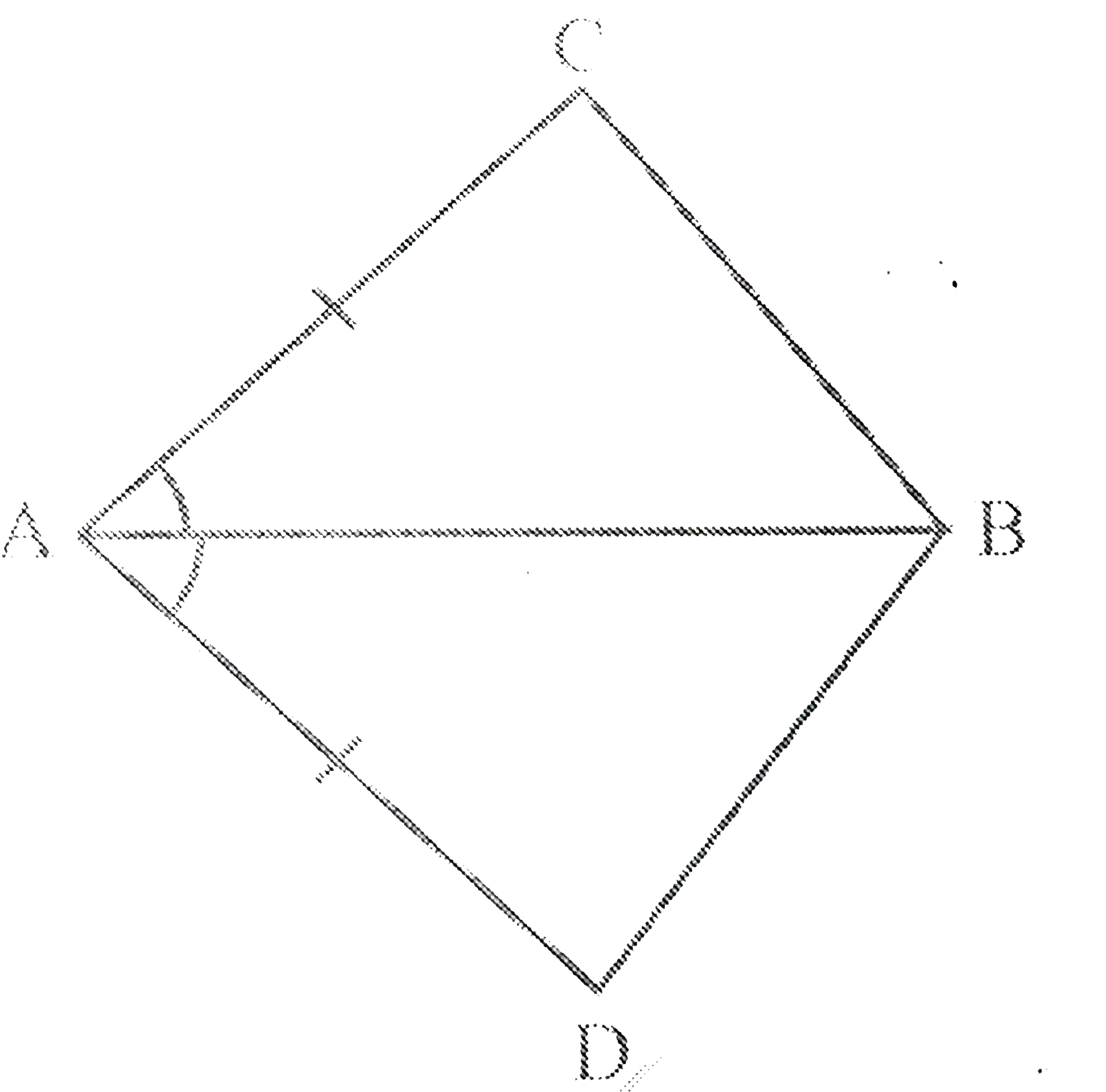
In quadrilateral $A C B D, A C = A D a n d A B$ bisects $∠ A$ . Show that $A B C ≅ A B D .$ What can you say about $B C a n d B D ?$

Video Solution

Text Solution

Verified by Experts

$3 x - 7 y^{2} + 3 x y z = 3 (2) - 7 {(- 4)}^{2} + 3 (2) (- 4) (1)$
$= 6 - 7 \times 16 - 6 \times 4$
$= 6 - 112 - 24$
$= 6 - 136$
$= 130$

Updated on:21/07/2023

|

Class 9 MATHS QUESTION BANK

Similar Questions

In quadrilateral ACBD, $A C = A D$ and AB bisects $∠ A$ (see Fig. 7.16). Show that $Δ A B C ≅ Δ A B D$

View Solution
The diagonals of quadrilateral $A B C D, A C and B D$ intersect in $O .$ Prove that if $B O = O D,$ the triangles $A B C and A D C$ are equal in area.
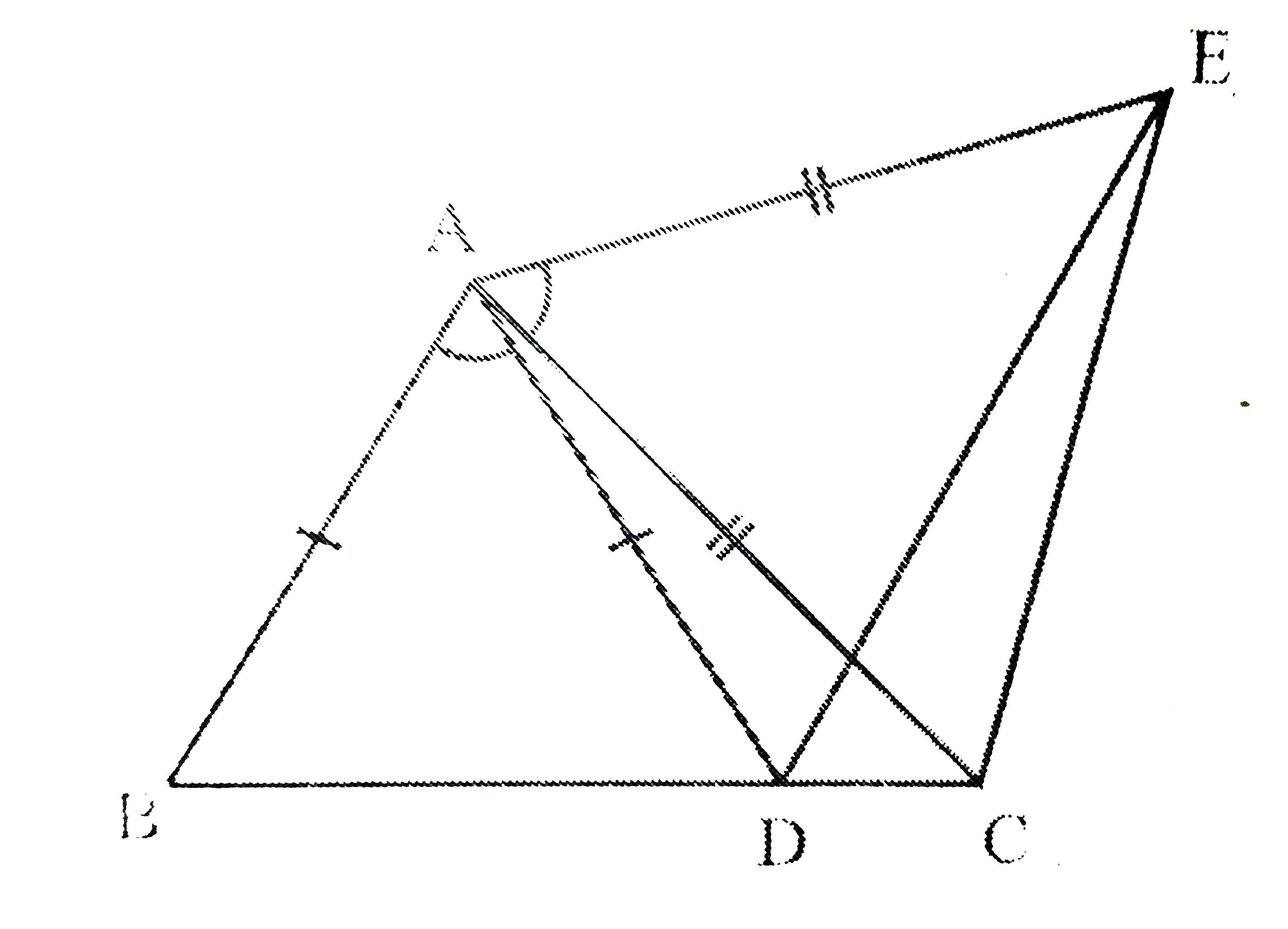
View Solution
In Fig. 7.21, $A C = A E$ , $A B = A D$ and $∠ B A D = ∠ E A C$ . Show that $B C = D E$ .

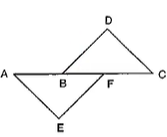
View Solution
In Figure, it is given that $A B = C F, E F = B D a n d ∠ A F E = ∠ C B D .$ Prove that $A F E = ≅ C B D$

View Solution
In a quadrilateral $A B C D$ , given that $∠ A + ∠ D = 90 o$ . Prove that $A C^{2} + B D^{2} = A D^{2} + B C^{2}$ .
View Solution
In a quadrilateral $A B C D,$ bisectors of angles $A a n d B$ intersect at $O$ such that $∠ A O B = 75^{\circ},$ then write the value of $∠ C + ∠ D$
View Solution
In $A B C, ∠ B = 60^{0}, ∠ C = 40^{0}, A L ⊥ B C a n d A D$ bisects $∠ A$ such that $L$ and $D$ lie on side $B C .$ Find $∠ L A D .$
View Solution
$A B C D$ is a cyclic quadrilateral whose diagonals $A C a n d B D$ intersect at $P .$ If $A B = D C,$ prove that:
(i) $P A B ≅ P D C$
(ii) $P A = P D a n d P C = P B$ (iii) $A D ∣ ∣ B C$
View Solution
$B D$ is one of the diagonals of a quadrilateral $A B C D . A M a n d C N$ are the perpendiculars from $A a n d C$ , respectively, on $B D .$ Show That $a r (q u a .. A B C D) = \frac{1}{2} B D . A M + C N$
View Solution
In a cyclic quadrilateral $A B C D$ , if $A B$ $∣ ∣$ $C D a n d ∠ B = 70^{\circ},$ find the remaining angles.
View Solution

Recommended Questions

Home
Ask Doubt
Profile