The direction rations of the diagonals of a cube which joins the origin to the opposite corner are (when the three concurrent edges of the cube are coordinate axes)
2√3,2√3,2√3
1,1,1
2,-2,1
1,2,3
The correct Answer is:B
Step by step video, text & image solution for The direction rations of the diagonals of a cube which joins the origin to the opposite corner are (when the three concurrent edges of the cube are coordinate axes) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PROBLEM SET (2)
12 Videos
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PROBLEM SET (3)
50 Videos
- CO-ORDINATE GEOMETRY OF THREE DIMENSIONBOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PASSAGE 4(THE SPHERE)( ANSWER THE FOLLOWING QURSTION BASED UPON ABOVE PASSAGE: )
3 Videos
- BINOMIAL THEOREM AND MATHEMATICAL INDUCTION BOOK - ML KHANNACHAPTER - BINOMIAL THEOREM AND MATHEMATICAL INDUCTION EXERCISE - Self Assessment Test
35 Videos
- COMPLEX NUMBERS BOOK - ML KHANNACHAPTER - COMPLEX NUMBERS EXERCISE - Assertion / Reason
2 Videos
Similar Questions
Find the angle between the diagonals of a cube with edges of length "a".
View SolutionA cube is placed so that one corner is at the origin and three edges are along the x-,y-, and ,z-axes of a coordinate system (figure).Use vector to compute
a.The angle between the edge along the z-axis (line ab) and the diagonal from the origin to the opposite corner (line ad).
b. The angle between line ac (the diagonal of a face ) and line ad.
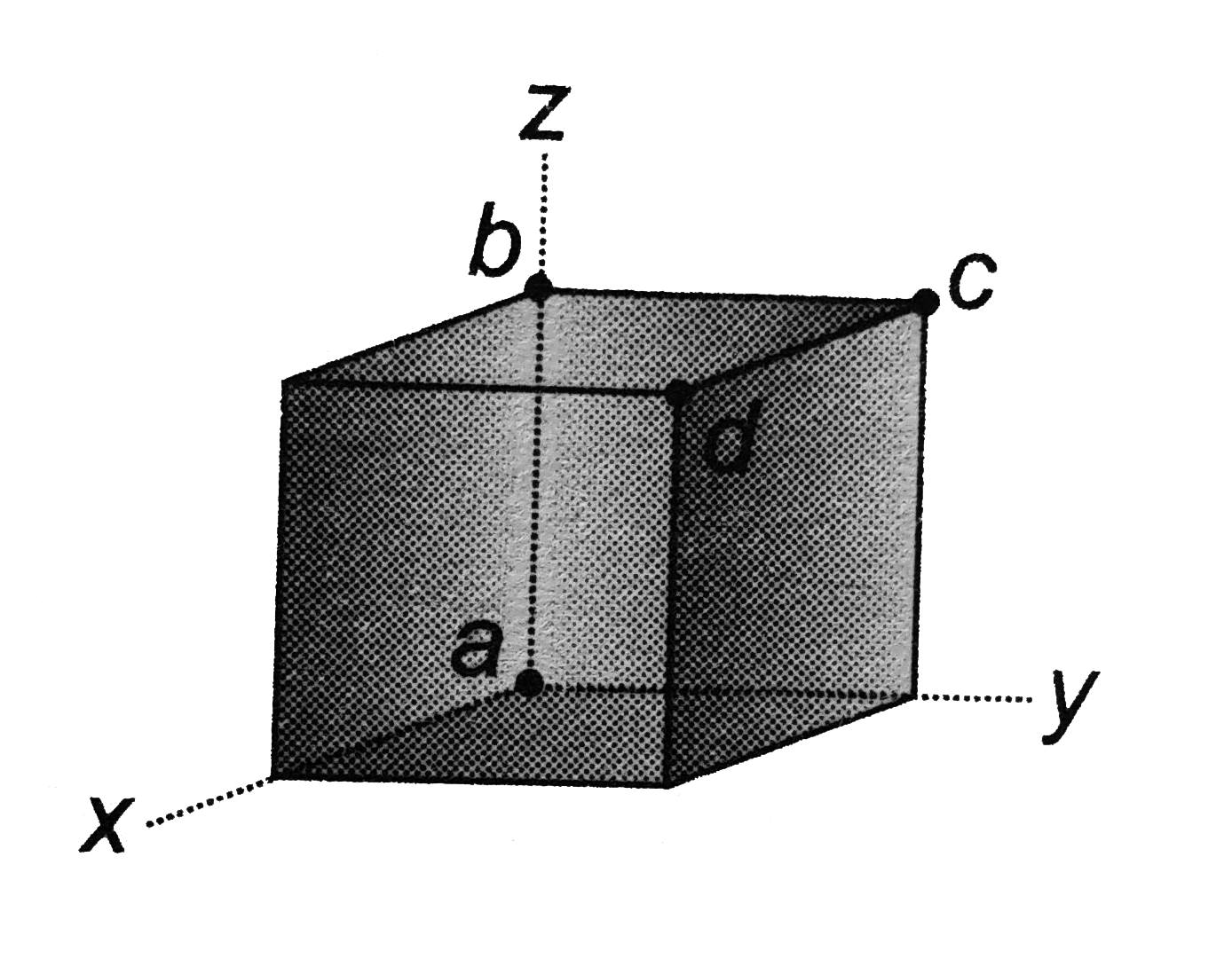
View Solution
Knowledge Check
The direction rations of the diagonals of a cube which joins the origin to the opposite corner are (when the three concurrent edges of the cube are coordinate axes)
A2√3,2√3,2√3B1,1,1C2,-2,1D1,2,3If the length of the diagonal of a cube is 6√3 cm , then length of the edge of the cube is 3 cm .
A2 cmB3 cmC6 cmD36√3 cmIf each edge of a cube is 6 cm, then find the diagonal of cube.
A6√2cmB6√3cmC36√2cmD36√3cm
If the length of the diagonal of a cube is 6√3 cm , then length of the edge of the cube is
View SolutionThe shortest distance between a diagonal of a unit cube and the edge skew to it, is
View SolutionThe angle between a diagonal of a cube and one of its edges is
View SolutionWhich of these is the length of the longest diagonal of a cube of edge a.
View SolutionIf three concurrent edges of a parallelopiped of volume V represent vectors →a,→b,→c then the volume of the parallelopiped whose three concurrent edges are the three concurrent diagonals of the three faces of the given parallelopiped is
View Solution
