Let A be a 2×2 matrix of the form A=[ab11], where a, b are integers and −50≤b≤50. The number of such matrices A such that A−1, the inverse of A, exists and A−1 contains only integer entries is
101
200
202
1012
The correct Answer is:C
Step by step video, text & image solution for Let A be a 2 xx 2 matrix of the form A = [[a,b],[1,1]], where a, b are integers and -50 le b le 50. The number of such matrices A such that A^(-1), the inverse of A, exists and A^(-1) contains only integer entries is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUESTION PAPER 2020Book:KVPY PREVIOUS YEARChapter:QUESTION PAPER 2020Exercise:PART-II(MATHEMATICS)
Explore 10 Videos
- QUESTION PAPER 2020Book:KVPY PREVIOUS YEARChapter:QUESTION PAPER 2020Exercise:PART-II : MATHEMATICS
Explore 7 Videos
- QUESTION PAPER 2013Book:KVPY PREVIOUS YEARChapter:QUESTION PAPER 2013Exercise:PART-II ( MATHEMATICS)
Explore 10 Videos
- SOLVED PAPER 2018Book:KVPY PREVIOUS YEARChapter:SOLVED PAPER 2018Exercise:EXAMPLE
Explore 27 Videos
Similar Questions
Knowledge Check
Let A be a 2×2 matrix of the form A=[ab11], where a, b are integers and −50≤b≤50. The number of such matrices A such that A−1, the inverse of A, exists and A−1 contains only integer entries is
A101B200C202D1012Let A+B=[235−1], where A is a symmetric matrix and B is a skew symmetric materix, then A×B is equal to
A[4214]B[−4214]C[4−2−1−4]D[−421−4]The inequality −1≤2x+4<5, where x is an integer can be represented on the number line as:
A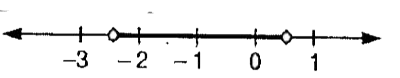 B
B C
C D
D
The number of ordered pairs (a,b) of positive integers such that2a−1b and 2b−1a are both integers is
View SolutionLet A be a square matrix all of whose entries are integers. If det A=±1 then Prove A−1 exists and all its entries.
View SolutionLet A be a symmetric matrix of order 2 with integer entries. If the sum of the diagonal elements of A2 is 1, then the possible number of such matrices is
View SolutionIf B is a 3×3 matrics such that B2=0 then det[(1+B)50−50B]=0
View SolutionIf f(x) =[x],where x is the greatest integer not greater than x,−2≤x≤2, then at x= 1
View Solution