Find all the values of the parameter 'a' for which the inequality 4x−a2x−a+3≤0 is satisfied by at least one real x.Solution in Punjabi
Putting 2x=t>0 then the original equation reduced in the form
t2−at−a+3=0………i
that the quadratic eq. I should have atleast one positive root (t>0) then
Discriminant D=(−a)2−4.1.(−a+3)≥0
⇒a2+4a−12≥0
⇒(a+6)(a−2)≥0
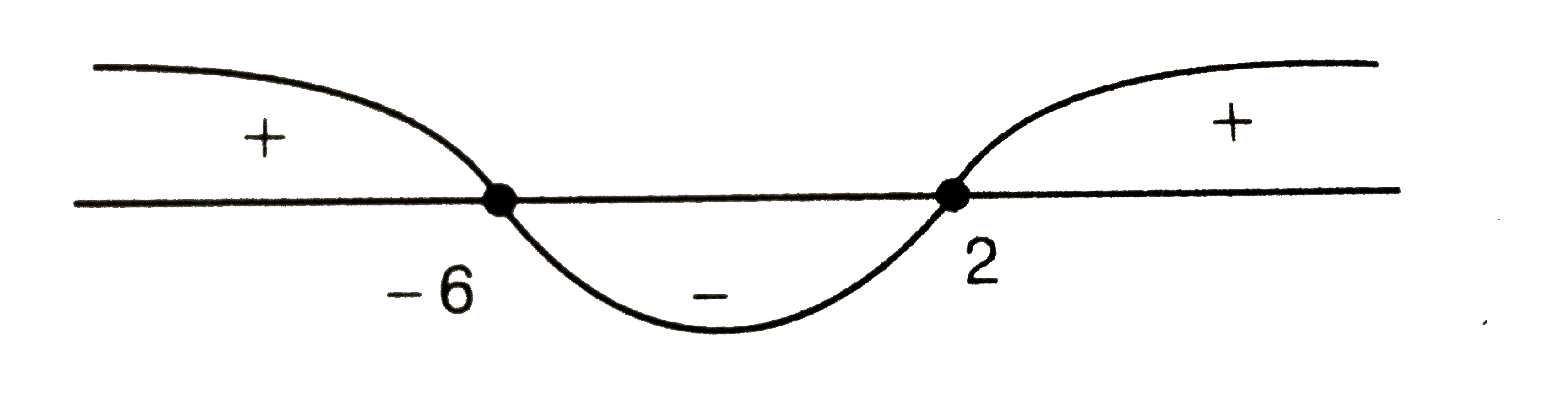
∴aε(−∞,−6]∪[2,∞)
If roots of eq i are t1 and t2 then
{t1+t2=at1t2=3−a
For aε(−∞,−6]
t1+t2<0 and t1t2>0. Therefore both roots are negatie and consequently the original equation has no solutions.
for aε[2,∞)
t1+t2>0 and t1t2>0 consequently atleast one of the roots t1 or t2 is greater than zero.
Thus, for aε[2,∞) the given equation has atleast one solution.
Topper's Solved these Questions
- THEORY OF EQUATIONSBOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise For Session 1
11 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise For Session 2
10 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHSCHAPTER - THE STRAIGHT LINESEXERCISE - The Straight Lines Exercise 8 : (Questions Asked in Previous 13 years Exams)
1 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
Similar Questions
If (a2−1)x2+(a−1)x+a2−4a+3=0 is identity in x , then find the value of a .
View SolutionIf α and β are the roots of the equation 2x2−3x+4=0, then the equation whose roots are α2 and β2, is a. 4x2+7x+16=0 b. 4x2+7x+6=0 c. 4x2+7x+1=0 d. 4x2−7x+16=0
View SolutionSolve the following system of inequalities graphically
x+5y≤25,2x+2y≥2,2x−2y≤0,x≥0,y≥0
View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
View SolutionIllustration 1.2 Find the set of all possible real values of a such that the inequality (x (a 1) (x (a2 2) 0 holds for all x E (-1,3)
View Solution3 The set of all real x satisfying the inequality (3-|x|)/(4-|x|)>=0
View SolutionFind m for which the inequality m2^(2x)-4.2^(x)+3m+1<0 is satisfied for at least one real x
View SolutionThe set of values of 'a' for which the inequality x^(2)-(a+2)x-(a+3)<0 is satisfied by atleast one positive real x,
View SolutionFind all values of the parameter a for which the inequality a.9x+4(a−1)3x+a>1 is satisfied for all real values of x
View SolutionFind all values of a for which the inequality (a+4)x^(2)-2ax+2a-6<0 is satisfied for all x in R.
View SolutionFind the values of the parameter a for which 4^(x)-a.2^(x)-a+3<=0 for atleast one real x.
View SolutionConsider the inequation x2+x+a−9<0
The values of the real parameter a so that the given inequations has at least one negative solution.
View SolutionConsider the inequation 9x−a3x−a+3≤0, where a is real parameter.
The given inequality has at least one negative soluiton for a∈
View SolutionConsider the inequation 9x−a3x−a+3≤0, where a is real parameter.
The given inequality has at least one real solutions for a∈.
View SolutionFind all values of x that satisfies the inequality 2x−3(x−2)(x−4)< 0.
View SolutionFind all values of x that satisfies the inequality 2x−3(x−2)(x−4)<0
View Solution
