A square has a rotational symmetry of order
1
2
3
4
The correct Answer is:D
To determine the order of rotational symmetry of a square, we can follow these steps:
1. Understand the Definition of Rotational Symmetry:
- A shape has rotational symmetry if it can be rotated around a central point and still look the same at certain angles.
2. Identify the Shape:
- In this case, we are dealing with a square. A square has four equal sides and four equal angles.
3. Determine the Center of Rotation:
- The center of a square is the point where the diagonals intersect. This is the point around which we will rotate the square.
4. Rotate the Square:
- Start with the square in its original position.
- Rotate the square by 90 degrees (a quarter turn). The square looks the same.
- Rotate it again by another 90 degrees (now 180 degrees total). The square still looks the same.
- Rotate it again by another 90 degrees (now 270 degrees total). The square still looks the same.
- Finally, rotate it one last time by 90 degrees (now 360 degrees total). The square returns to its original position.
5. Count the Rotations:
- We have found that the square looks the same after 90 degrees, 180 degrees, 270 degrees, and 360 degrees of rotation.
- This means there are 4 distinct positions where the square looks the same during a full rotation.
6. Conclusion:
- Therefore, the order of rotational symmetry of a square is 4.
Final Answer:
The order of rotational symmetry of a square is 4.
- A shape has rotational symmetry if it can be rotated around a central point and still look the same at certain angles.
- In this case, we are dealing with a square. A square has four equal sides and four equal angles.
- The center of a square is the point where the diagonals intersect. This is the point around which we will rotate the square.
- Start with the square in its original position.
- Rotate the square by 90 degrees (a quarter turn). The square looks the same.
- Rotate it again by another 90 degrees (now 180 degrees total). The square still looks the same.
- Rotate it again by another 90 degrees (now 270 degrees total). The square still looks the same.
- Finally, rotate it one last time by 90 degrees (now 360 degrees total). The square returns to its original position.
- We have found that the square looks the same after 90 degrees, 180 degrees, 270 degrees, and 360 degrees of rotation.
- This means there are 4 distinct positions where the square looks the same during a full rotation.
- Therefore, the order of rotational symmetry of a square is 4.
The order of rotational symmetry of a square is 4.
Topper's Solved these Questions
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - Exercise (Match the following)
1 Videos
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - Exercise (Very Short Answer Type)
10 Videos
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - NCERT SECTION (Exercise -13.3)
7 Videos
- SIMPLE EQUATIONSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SIMPLE EQUATIONSEXERCISE - Olympiad /Hots Corner
18 Videos
- THE TRIANGLE AND ITS PROPERTIES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - THE TRIANGLE AND ITS PROPERTIES EXERCISE - Olympiad/Hots Corner
20 Videos
Similar Questions
Knowledge Check
A figure has a rotational symmetry of order more than 1, the angle of rotation can be
A21∘B22∘C23∘D24∘A figure has a rotational symmetry of order more than 1, the angle of rotation can be
A25∘B33∘C42∘D40∘
Rhombus is a figure that has _______lines of symmetry and has a rotational symmetry of order__________.
View SolutionEach of the letters H,N,S and Z has a rotational symmetry of order ____________.
View SolutionA ceiling fan having 4 blades has a rotational symmetry of the order 2×K. Find K.
View SolutionShow that each of the letters H,I,N,S,X and Z has a rotational symmetry of order 2. Also, mark the point of rotational symmetry in each case.
View SolutionThe rectangle has rotational symmetry of order (a) 1 (b) 2 (c) 3 (d) 4
View SolutionWhich of the following figures have rotational symmetry of order more than 1?
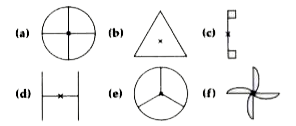
View Solution