Question

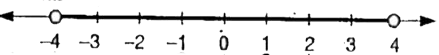
The absolute value of a number is its distance from 0 on a number line. The number line representing the inequaltiy $\left|x\right|<4$ is

Options

A.
B.
C.
D.

Answer

A

Explanation

To solve the inequality |x| < 4, we need to understand what the absolute value means. The absolute value of a number x, denoted as |x|, represents the distance of x from 0 on the number line.

Here’s how we can solve the inequality step by step:

Step 1: Understand the definition of absolute value
The inequality |x| < 4 means that the distance of x from 0 is less than 4. This can be interpreted as x being between -4 and 4.

Step 2: Split the absolute value inequality
We can express the inequality |x| < 4 in two parts:
1. When x is positive: x < 4
2. When x is negative: -x < 4, which can be rewritten as x > -4 (after multiplying by -1 and reversing the inequality).

Step 3: Combine the inequalities
From the two parts, we have:
- From the first part: x < 4
- From the second part: x > -4

Combining these two inequalities gives us:
-4 < x < 4

Step 4: Represent the solution on a number line
On a number line, we will represent the solution -4 < x < 4. This means:
- We will place open circles (hollow circles) at -4 and 4 to indicate that these points are not included in the solution.
- The region between -4 and 4 will be shaded to show all the numbers that satisfy the inequality.

Final Answer
The number line representing the inequality |x| < 4 is shown with open circles at -4 and 4, and the region between them shaded.

---