A function f:R→R satisfy the equation f(x)f(y)−f(xy)=x+y for all x,y∈R and f(y)>0, thenSolution in Bengali
f(x)f−1(x)=x2−4
f(x)f−1(x)=x2−6
f(x)f−1(x)=x2−1
none of these
The correct Answer is:C
Taking x = y = 1, we get
f(1)f(1)−f(1)=2
⇒ f2(1)−f(1)−2=0
⇒ (f(1)−2)(f(1)+1)=0
⇒ f(1)=2
Taking y = 1, we get
f(x).f(1)−f(x)=x+1
⇒ f(x)=x+1
⇒ f−1(x)=x−1
∴ f(x).f−1(x)=x2−1
Topper's Solved these Questions
- FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - Multiple Correct Answers Type
9 Videos
- FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - Comprehension Type
7 Videos
- EQUATION OF PLANE AND ITS APPLICATIONS -IIBOOK - CENGAGE PUBLICATIONCHAPTER - EQUATION OF PLANE AND ITS APPLICATIONS -IIEXERCISE - DPP 3.4
8 Videos
- GETTING STARTED WITH GRAPHSBOOK - CENGAGE PUBLICATIONCHAPTER - GETTING STARTED WITH GRAPHSEXERCISE - Exercises 1.18
1 Videos
Similar Questions
If f(x)=3x2−x+1, then find f(−1)
View SolutionLet f(x)=x2−2x−1∀x∈R.Letf:(−∞,a]→[b,∞), where a is the largest real number for which f(x) is bijective.
If f:R→R, then range of values of k for which equation f(|x|)=k has 4 distinct real roots is
View SolutionLet A(0,6,6), B(6,6,0) and C(6,0,6) are three points and point D is moving on the line x+z−3=0=y. If G is centroid of ΔABC, then minimum value of GD is
View SolutionFollowing is the graph of y = f'(x) and f(0) = 0 .
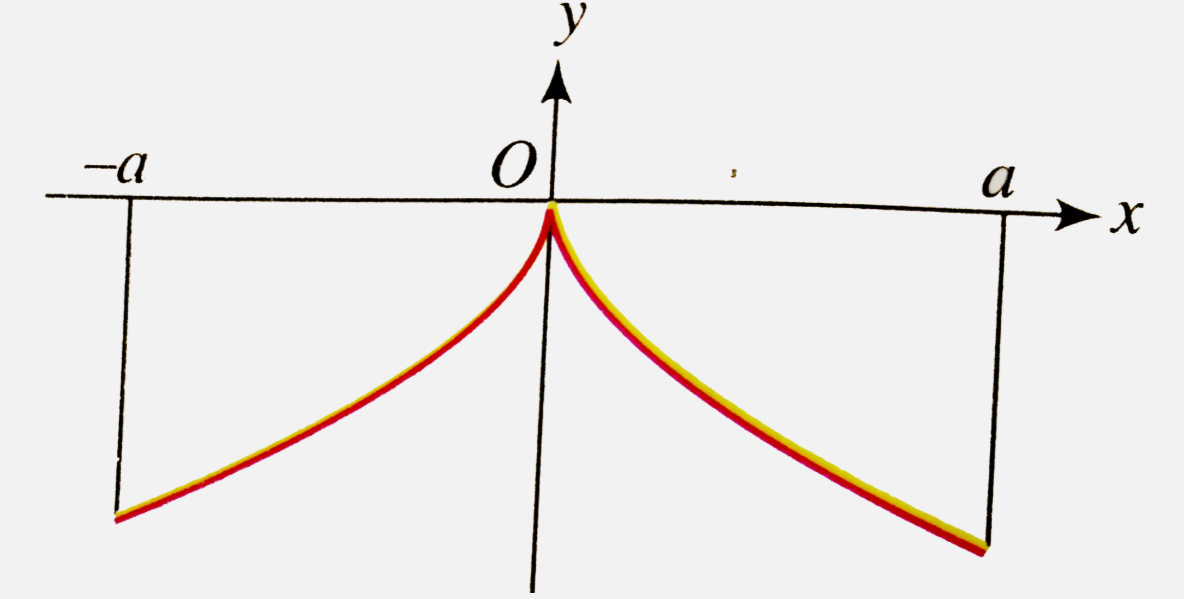
(a) What type of function y = f'(x) is ? Odd or even?
(b) What type of function y = f(x) is ? Odd or even?
(c) What is the value of ∫a−af(x)dx?
(d) Has y = f(x) point of inflection?
(e) What is the nature of y = f(x)? Monotonic or non-monotonic?
View SolutionA function f:R rarr R satisfy the equation f(x)f(y)-f(xy)=x+y for all x,y in R and f(y)>0, then
View SolutionIf a real valued function f(x) satisfies the equation f(x+y)=f(x)+f(y) for all x,y in R then f(x) is
View SolutionA function f:R→R satisfies the equation f(x+y)=f(x).f(y) for all, f(x)≠0. Suppose that the function is differentiable at x = 0 and f'(0) = 2. Then,
View Solutionमाना लीजिये f:R→R एक फलन है, जो समीकरण
f(x+y)=f(x)+f(y),∀x,y∈R
को संतुष्ट करता है। यदि x=0 पर फलन f(x) अवकलनीय (differentiable) है, तो -
View SolutionIf for a function f : R→Rf(x+y)=F(x)+f(y) for all x and y then f(0) is
View SolutionA function f:R→R satisfies that equation f(x+y)=f(x)f(y) for all x, y∈R , f(x)≠0 . Suppose that the function f(x) is differentiable at x=0 and f′(0)=2 . Prove that f′(x)=2 f(x) .
View SolutionA function f : R→R satisfies the equation f(x)f(y)−f(xy)=x+y∀x,y∈Randf(1)>0, then
View SolutionA function f:R→R satisfy the equation f(x)f(y)−f(xy)=x+y for all x,y∈R and f(y)>0, then
View SolutionA function f:R→R satisfies that equation f(x+y)=f(x)f(y) for all x, y∈R , f(x)≠0 . Suppose that the function f(x) is differentiable at x=0 and f′(0)=2 . Prove that f′(x)=2 f(x) .
View Solutionमाना लीजिये f:R→R एक फलन है, जो समीकरण
f(x+y)=f(x)+f(y),∀x,y∈R
को संतुष्ट करता है। यदि x=0 पर फलन f(x) अवकलनीय (differentiable) है, तो -
View SolutionA function f:R→R satisfies the equation f(x+y)=f(x).f(y) for all, f(x)≠0. Suppose that the function is differentiable at x = 0 and f'(0) = 2. Then,
View Solution
