The area (in sq. units) of the region described by {x,y):y2≤2xandy≥4x−1} is Solution in Bengali
A. 732
B. 564
C. 1564
D. 932
The correct Answer is:D
y2≤2x represents the interior of parabola y2=2x (towards focus) and y≥4x−1 represents the half region of plane to the left of the line y=4x−1.
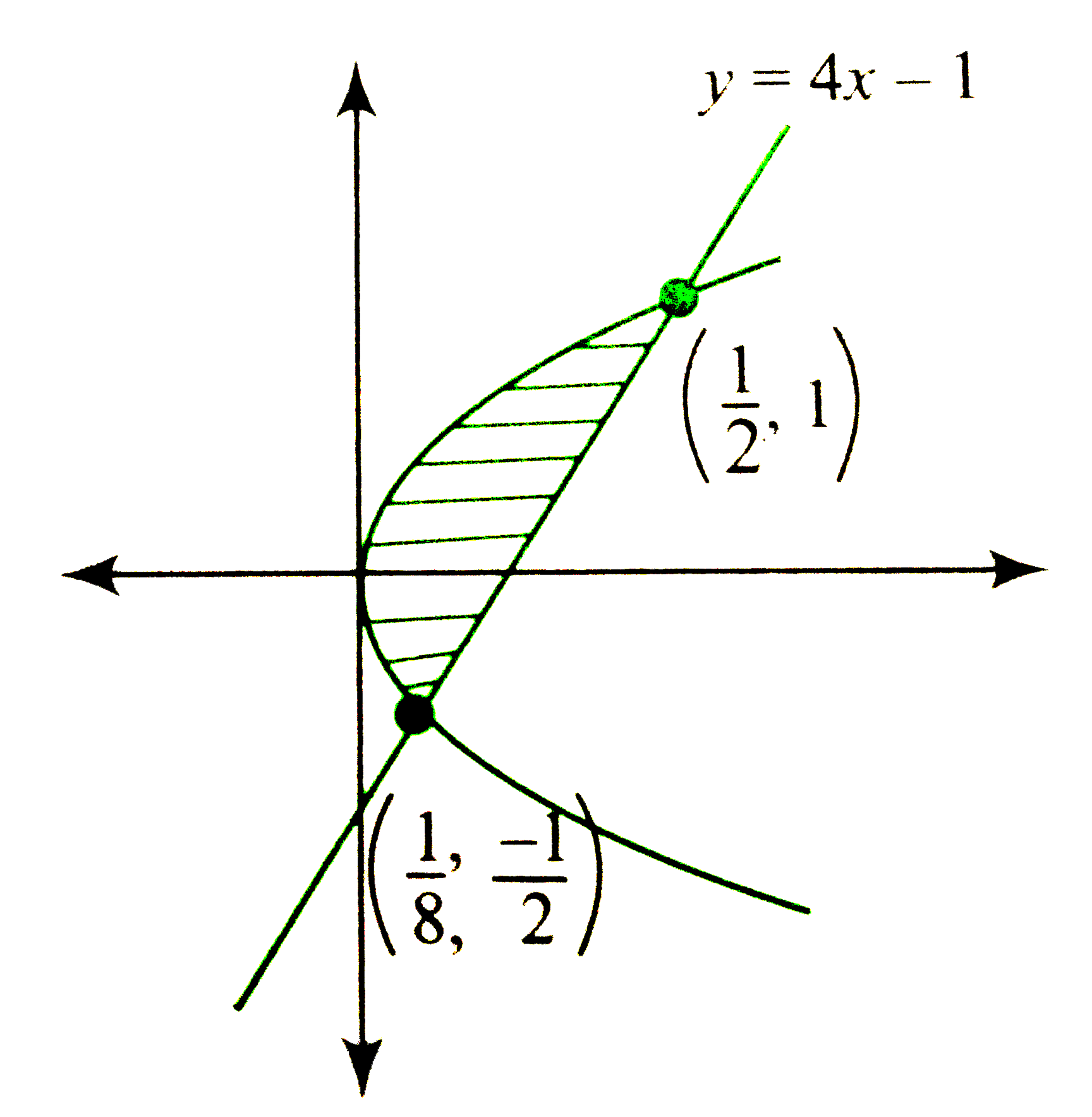
1∫−1/2(y+14−y22)dy=14{y22+y}1−1/2−16{y3}1−1/2
=14{(12+1)−(18−12)}−16{1+18}
=14{32+38}−16{98}=1532−632=932
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Single Correct Answer Type30videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Multiple Correct Answer Type3videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Numerical Value Type18videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type2videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
Similar Questions
Let the straight line x= b divide the area enclosed by y=(1−x)2,y=0,andx=0 into two parts R1(0≤x≤b)andR2(b≤x≤1) such that R1−R2=14. Then b equals
03:59View SolutionIf the area of bounded between the x-axis and the graph of y=6x−3x2 between the ordinates x=1andx=a is 19 units, then a can take the value 4or−2 two value are in (2,3) and one in (−1,0) two value are in (3,4) and one in (−2,−1) none of these
08:10View SolutionThe area enclosed by the curve c:y=x√9−x2(x≥0) and the x-axis is________
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
01:25View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is04:17View SolutionThe area (in sq units) of the region {(x,y):y^(2)>=2x and x^(2)+y^(2) =0,y>=0} is
07:21View SolutionThe area (in sq units) of the region described by {(x,y):y2≤2xandy≥4x−1} is
03:37View SolutionThe area (in sq. units) of the region
A=[(x,y):0≤y≤x|x|+1 and −1≤x≤x] isView SolutionThe area (in sq. units) of the region described by A={(x,y):y≥x2−5x+4,x+y>1,y≤0} is
07:05View SolutionThe area (in sq units) of the region described by {(x,y):y2≤2xandy≥4x−1} is
06:16View SolutionThe area (in sq. units) of the region described by {(x,y):y2≤2x and y≥4x−1} is-
02:07View SolutionThe area (in sq units) of the region described by A={(x,y):x2+y2≤1 and y2≤1−x} is :
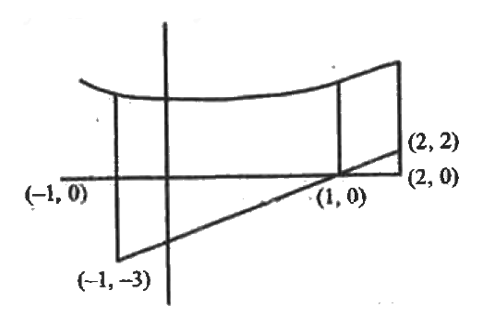
03:10View SolutionThe area (in sq. units) of the region bounded by x=−1,x=2,y=x2+1andy=2x−2 is
 04:43View Solution
04:43View SolutionThe area ( in sq. units ) of the region A={xy|x|+|y∣∣≤1,2y2≥∣∣x∣} is :
05:22View SolutionThe area (in sq. units) of the region described by {(x,y):y(2)≤2xandy2≤2xandy≥4x−1}
10:50View SolutionThe area (in sq. units) of the region described by : {(x,y):y2≤2x and y≥4x−1 } is :
07:27View Solution
