Number of points with integral co-ordinates that lie inside a triangle whose co-ordinates are (0, 0), (0, 21) and (21,0).Solution in Bengali
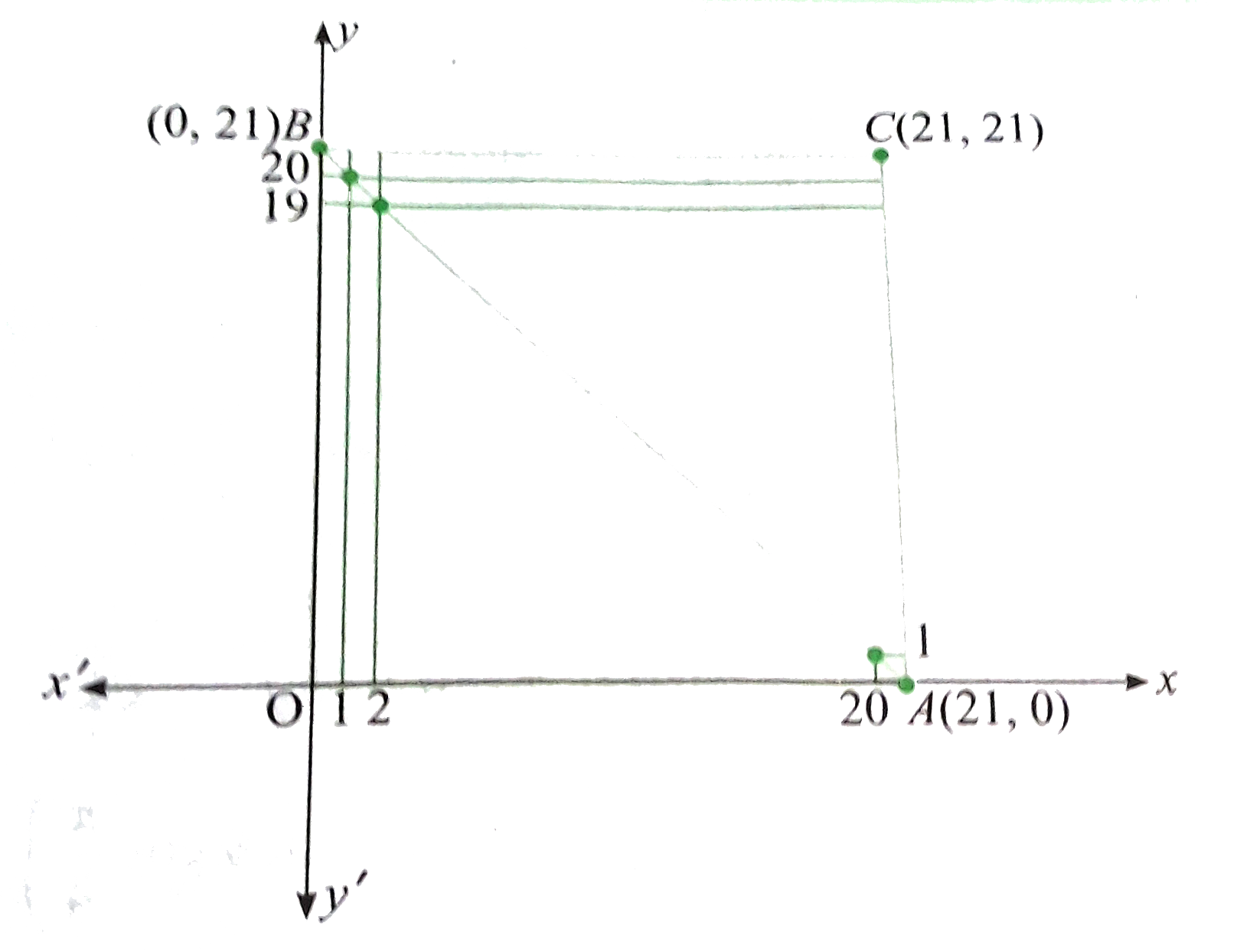
Total number of points within the square OACB=20×20=400 there are 20 points on the line AB which are (1,20),(2,19),......(20,1).
∴ Points within ΔOAB and ΔABC=400−20=380 by symmetry, points within ΔOAB=3802=190
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.2
1 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Illustration1.3
1 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
The point (4,1) undergoes the following three transformations successively
(a) Reflection about the line y=x
(b)Translation through a distance 2 units along the positive direction of the x-axis
( c) Rotation through an angle π/4 about the origin in the anti clockwise direction.
The final position of the point is given by the co-ordinates
View SolutionAt what point should the origin be shifted if the coordinates of a point (4,5) become (−3,9)?
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionNumber of points with integral co-ordinates that lie inside a triangle whose co-ordinates are (0,0),(0,21) and (21,0) .
View SolutionIf (a,b) are the co-ordinates of a point where b=0, where will the point lie.
View SolutionThe polar co-ordinates of the point whose cartesian co-ordinates are (5,0), are
View SolutionThe polar co-ordinates of the point whose cartesian co-ordinates are (−3,0), are
View SolutionThe polar co-ordinates of the point whose cartesian co-ordinates are (0,−2), are
View SolutionTriangle is formed by the co-ordinates (0, 0), (0, 21) and (21, 0). Find the number of integral co-ordinates strictly inside the triangle (integral co-ordinates has both x and y) :
View SolutionThe x-co-ordinate of the incentre of the triangle that has the co-ordinates of mid-points of its sides as (0, 1), (1, 1) and (1, 0) is :
View SolutionThe number of points, having both co-ordinates as integers, that lie in the interior of the triangle with vertices (0, 0), (0, 41) and (41, 0), is :
View SolutionNumber of points with integral co-ordinates that lie inside a triangle whose co-ordinates are (0, 0), (0, 21) and (21,0).
View SolutionNumber of points with integral co-ordinates that lie inside a triangle whose co-ordinates are (0, 0), (0, 21) and (21,0).
View SolutionThe number of points, having both co-ordinates as integers, that lie in the interior of the triangle with vertices (0,0), (0,41) and (41,0) is
View SolutionThe x co-ordinate of the incentre of the triangle that has the co-ordinates of mid points of its sides as (0, 1) (1, 1) (1, 0) is
View Solution
