Triangle ABC with AB=13,BC=5, and AC=12 slides on the coordinates axes with AandB on the positive x-axis and positive y-axis respectively. The locus of vertex C is a line 12x−ky=0. Then the value of k is_____________Solution in Bengali

Since ∠BCA=90∘, points A, O, B, C are concyclic.
Let ∠AOC=θ.
∠BOC=∠BAC
tan(π2−θ)=512
xy=512 or 12−5y=0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE MAIN)
9 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE ADVANCED)
3 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Video
Similar Questions
The line L given by x5+yb=1 passes through the point (13,32).the line K is parallel to L and has the equation xc+y3=1 then the distance between L and K is
View SolutionThe locus of the orthocentre of the triangle formed by the lines (1+p)x−py+p(1+p)=0,(1+q)x−qy+q(1+q)=0 and y = 0, where p≠⋅q, is (A) a hyperbola (B) a parabola (C) an ellipse (D) a straight line
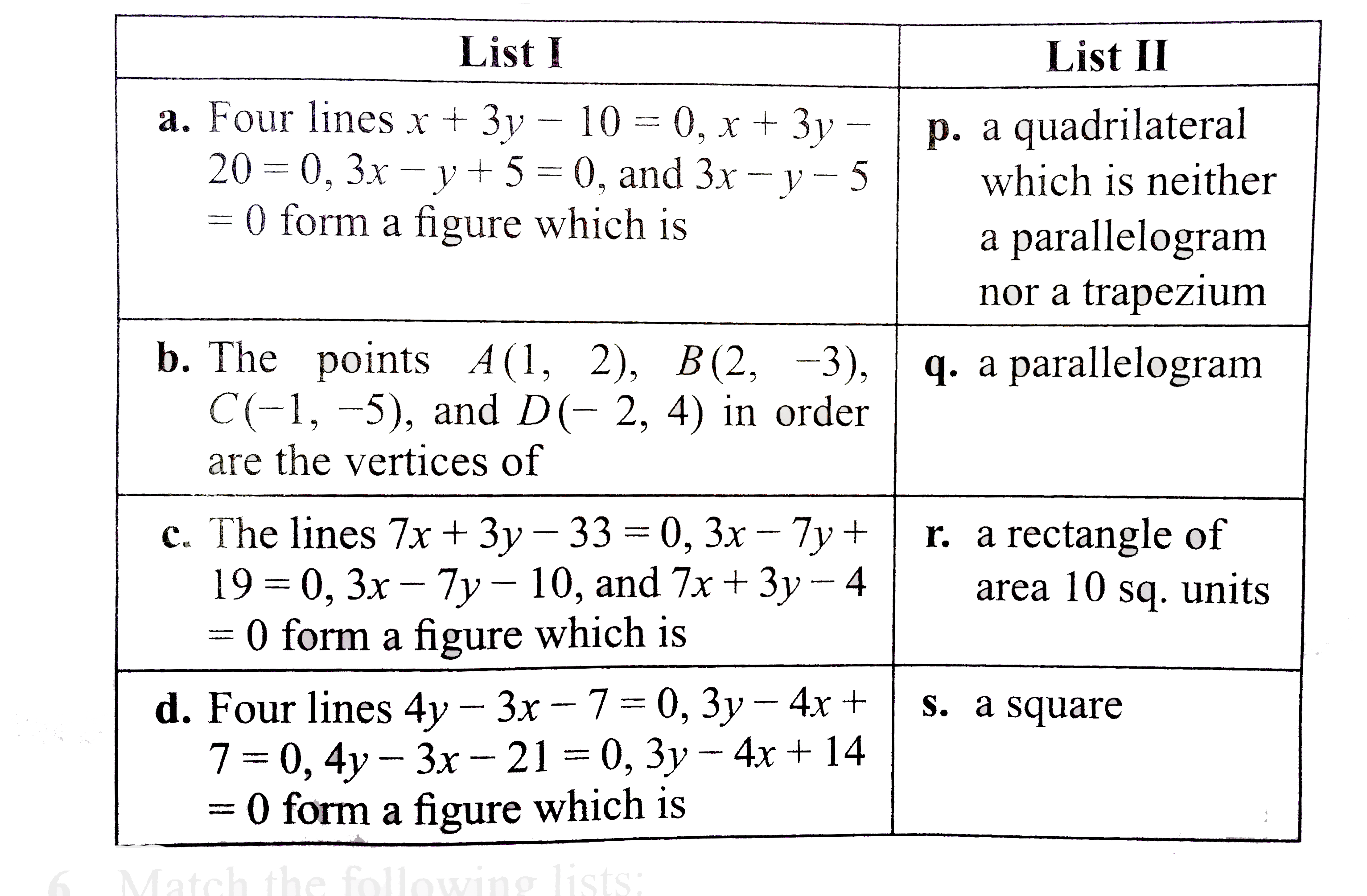
View SolutionMatch the following lists:
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionTriangle ABC with AB=13,BC=5, and AC=12 slides on the coordinates axes with AandB on the positive x-axis and positive y-axis respectively. The locus of vertex C is a line 12x−ky=0. Then the value of k is_____________
View SolutionThe line 3x+4y=12 cuts x -axis at A and y_(-) axis at B. The equation of the excircle of the triangle formed by this line and coordinate axes,opposite to the vertex B is
View SolutionIf a,b,c are in G.P. write te area of the triangle formed by the line ax+by+c=0 with the coordinates axes.
View SolutionOne vertex of a triangle is a fixed point from where three lines pass (a-b)x-(b-c)y+(c-a)=0,(b-c)x-(c-a)y+(a-b)=0,(c-a)x-(a-b)y+(b-c) Another vertex is (3,4) and the third vertex lies on x- axis Then locus of the centroid of triangle is
View SolutionIf C is the reflection of A(2,4) in x -axis and B is the reflection of C in y-axis then |AB| is 4sqrt(k) then k=
View SolutionB and C are the vertex of a triangle ABC with coordinate (2 , 0) and (8 , 0) respectively. Another vertex A moves in such a way that it satisfies the relation 4 tanB2tanC2=1. If the equation of locus of A is (x−5)252+y2k2=1 , then the value fo k is _
View SolutionThe vertices A and D of square ABCD lie on the positive sides of xandy axis , respectively. If the vertex C is the point (12,17) , then the coordinates of vertex B are (a)(14,16) (b)(15,3) (c)(17,5) (d) (17,12)
View SolutionTriangle ABC with AB=13,BC=5, and AC=12 slides on the coordinates axes with AandB on the positive x-axis and positive y-axis respectively. The locus of vertex C is a line 12x−ky=0. Then the value of k is_____________
View SolutionThe vertices A and D of square ABCD lie on the positive sides of x− and y-axis , respectively. If the vertex C is the point (12,17) , then the coordinates of vertex B are (a) (14,16) (b) (15, 3) (c) 17,5) (d) (17,12)
View SolutionIf a,b,c are in G.P. write the area of the triangle formed by the line ax+by+c=0 with the coordinates axes.
View SolutionABC is a variable triangle with a fixed centriod (5,5) the side BC = 13 and B,C move on x and y - axis respectively. Find the equation of locus of vertex 'A'.
View Solution
