The equations of two sides of a triangle are 3y−x−2=0andy+x−2=0. The third side, which is variable, always passes through the point (5,−1) . Find the range of the values of the slope of the third side, so that the origin is an interior point of the triangle.Solution in Bengali
We have two sides of triangle as 3x-x-2=0 and y+x-2=0.
The third side is passing through the point A(5,-1).
Different lines through point A are drawn as shown in the following figure.
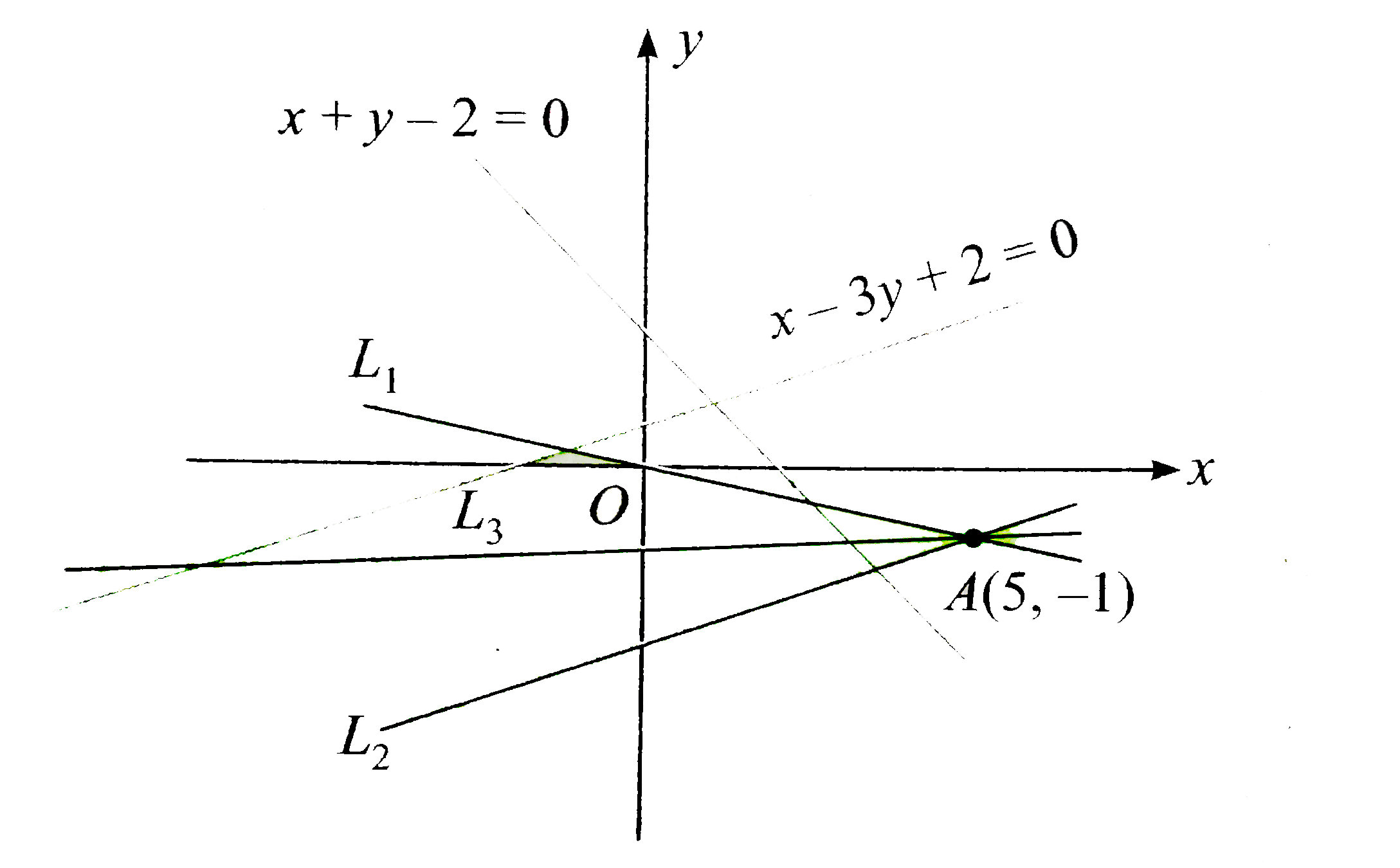
Line L2 through A is parallel to the line x-3y+2=0, which has slope 1/3.
In this case, triangle is not formed.
Line L1 through A goes through origin, which has slope -1/5.
In this case triangle is formed but origin lies on the side of the triangle.
Line L3 through A is such that triangle is formed and origin lies inside the triangle.
Thus, required values of slope of line are (-1/5,1/3).
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.2
4 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)
1 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Find the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
View SolutionTwo particles start from point (2, -1), one moving two units along the line x+ y = 1 and the other 5 units along the line x - 2y = 4, If the particle move towards increasing y, then their new positions are:
View SolutionFor a point P in the plane, let d1(P)andd2(P) be the distances of the point P from the lines x−y=0andx+y=0 respectively. The area of the region R consisting of all points P lying in the first quadrant of the plane and satisfying 2≤d1(P)+d2(P)≤4, is
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionTwo sides of an isosceles triangle are given by the equations 7x−y+3=0andx+y−3=0 and its third side passes through the point (1,−1). Determine the equation of the third side.
View SolutionThe sides of a triangle have the combined equation x2−3y2−2xy+8y−4=0 . The third side, which is variable, always passes through the point (−5,−1) . Find the range of values of the slope of the third line such that the origin is an interior point of the triangle.
View SolutionThe equations of two sides of a triangle are 3y−x−2=0andy+x−2=0. The third side, which is variable, always passes through the point (5,−1) . Find the range of the values of the slope of the third side, so that the origin is an interior point of the triangle.
View SolutionTwo sides of a triangle are (a+b)x+(a-b)y-2ab=0 and (a-b)x+(a+b)y-2ab=0 .If the triangle is isosceles and the third side passes through point (b-a,a-b), then the equation of third side can be
View SolutionTwo sides of a triangle have the joint equation x2−2xy−3y2+8y−4=0 The third side , which is variable always passes through the point (−5,−1) .Find the range of values of the slope of the third side , so that the origin is an interior point of the triangle .
View SolutionThe equation of the lines through the origin which form two of the sides of the equilateral triangle having x=2 as the third side is
View SolutionThe sides of a triangle have the combined equation x2−3y2−2xy+8y−4=0 . The third side, which is variable, always passes through the point (−5,−1) . Find the range of values of the slope of the third line such that the origin is an interior point of the triangle.
View SolutionThe equations of two sides of a triangle are 3y−x−2=0andy+x−2=0. The third side, which is variable, always passes through the point (5,−1) . Find the range of the values of the slope of the third side, so that the origin is an interior point of the triangle.
View SolutionThe sides of a triangle have the combined equation x2−3y2−2xy+8y−4=0 . The third side, which is variable, always passes through the point (−5,−1) . Find the range of values of the slope of the third line such that the origin is an interior point of the triangle.
View SolutionThe sides of a triangle have the combined equation x2−3y2−2xy+8y−4=0 . The third side, which is variable, always passes through the point (−5,−1) . Find the range of values of the slope of the third line such that the origin is an interior point of the triangle.
View SolutionThe equation of the lines through the origin which form two of the
sides of the equilateral triangle having x=2 as the third side is
View Solution
