A line through A(-5,-4) meets the lines x+3y+2=0, 2x+y+4=0 and x-y-5=0 at the points B, C and D respectively. If ${(\frac{15}{A B})}^{2} + {(\frac{10}{A C})}^{2} = {(\frac{6}{A D})}^{2}$ find the equation of the line.Solution in Bengali

Video Solution

Text Solution

Verified by Experts

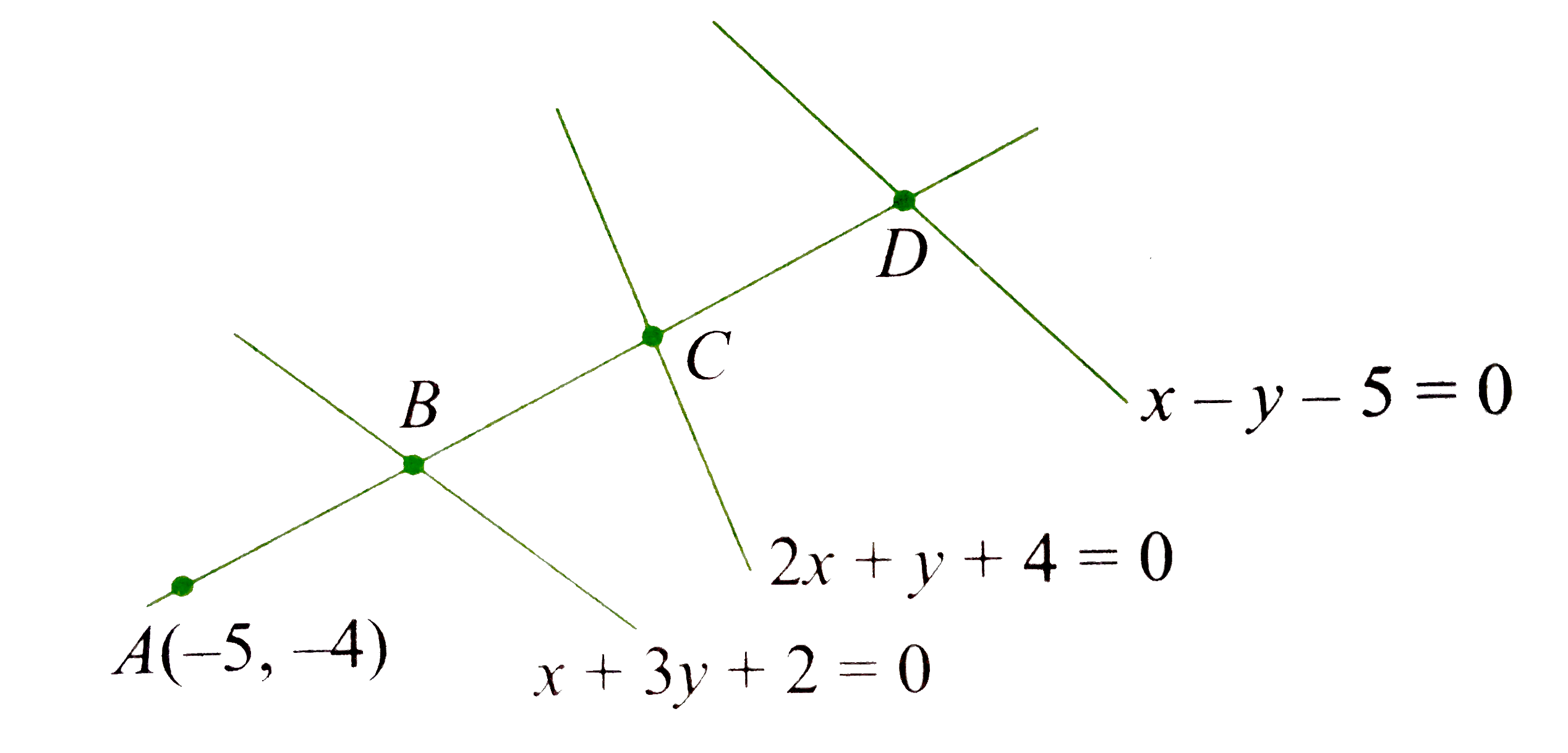
Let $θ$ be the inclination of line through A(-5,-4).
Therefore, equation of this line is $\frac{x + 5}{cos θ} = \frac{y + 4}{sin θ} = r (1)$
Any point on this line at distance r from point A (-5,-4) is given by
$(- 5 + r cos θ, - 4 + r sin θ)$
$If A B = r_{1}, A C = r_{2} and A D = r_{3}, then$
$B (r_{1} cos θ - 5, r_{1} sin θ - 4)$
$C (r_{2} cos θ - 5, r_{2} sin θ - 4)$
$D (r_{3} cos θ - 5, r_{3} sin θ - 4)$
But B lies on x+3y+2 = 0. Therefore,
$r_{1} cos θ - 5 + 3 r_{1} sin θ - 12 + 2 = 0$
$or \frac{15}{cos θ + 3 sin θ} = r_{1}$
$or \frac{15}{A B} = cos θ + 3 sin θ$ (1)
As C lies on 2x+y+4 = 0, we have
$2 (r_{2} cos θ - 5) + (r_{2} sin θ - 4) + 4 = 0$
$or r_{2} = \frac{10}{2 cos θ + sin θ} = A C$
$or \frac{10}{A C} = 2 cos θ + sin θ$ (2)
Similarly, D lies on x-y-5=0. Therefore,
$r_{3} cos θ - 5 - r_{3} sin θ + 4 - 5 = 0$
$or r_{3} = \frac{6}{cos θ - \sin θ} = A D$
$or \frac{6}{A D} = cos θ - sin θ (3)$
Now, given that
${(\frac{15}{A B})}^{2} + {(\frac{10}{A C})}^{2} = {(\frac{6}{A D})}^{2}$
$or {(cos θ + 3 sin θ)}^{2} + {(2 cos θ + sin θ)}^{2} = {(cos θ - sin θ)}^{2} [Using (1), (2), and (3)]$
$or 4 {cos}^{2} θ + 9 {sin}^{2} θ + 12 sin θ cos θ = 0$
$or {(2 cos θ + 3 sin θ)}^{2} = 0$
$or tan θ = - \frac{2}{3}$
Hence, the equation of the required line is
$y + 4 = - \frac{2}{3} (x + 5)$
or 3y+12 = -2x-10
or 2x+3y+22=0

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-STRAIGHT LINES-EXAMPLE

Home
Ask Doubt
Profile