Statement 1: there exists a unique sphere which passes through the three non-collinear points and which has the least radius. Statement 2: The centre of such a sphere lies on the plane determined by the given three points.Solution in Bengali
(a) Both the statements are true, and Statement 2 is the correct explanation for Statement 1.
(b) Both the Statements are true, but Statement 2 is not the correct explanation for Statement 1.
(c) Statement 1 is true and Statement 2 is false.
(d) Statement 1 is false and Statement 2 is true.
The correct Answer is:c
Obiviously the answer is (b).
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - LINKED COMPREHENSION TYPE
12 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MATRIX-MATCH TYPE
5 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CORRECT ANSWER TYPE
17 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions
291 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
Similar Questions
Given four points A(2,1,0),B(1,0,1),C(3,0,1)andD(0,0,2). Point D lies on a line L orthogonal to the plane determined by the points A, B and C.
The equation of the plane ABC is
View Solution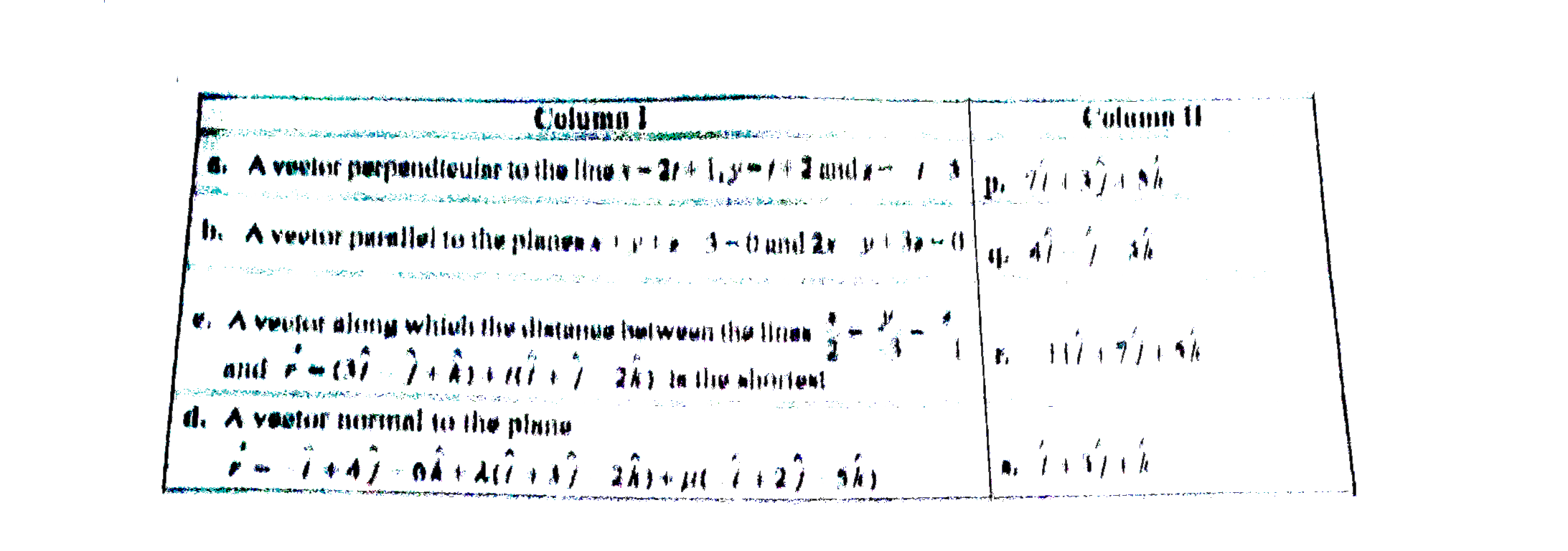
View SolutionLet PM be the perpendicular from the point P(1,2,3) to the x−y plane. If →OP makes an angle θ with the positive direction of the z− axis and→OM makes an angle φ with the positive direction of x− axis, whereO is the origin and θandφ are acute angels, then a. cosθcosφ=1/√14 b. sinθsinφ=2/√14 c. tanφ=2 d. tanθ=√5/3
View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
View SolutionStatement 1: there exists a unique sphere which passes through the three non-collinear points and which has the least radius. Statement 2: The centre of such a sphere lies on the plane determined by the given three points.
View SolutionWhat is the number of planes passing through three non-collinear points?
View SolutionConsider a sphere passing through the origin and the points (2,1,-1),(1,5,-4),(-2,4,-6).
Consider the following statements :
1. The sphere passes through the point (0,4,0).
2. The point (1,1,1) is at a distance of 5 unit from the centre of the sphere.
Which of the above statement is/are correct?
View SolutionStatement-1: There are p≥8 points in space no four of which are in the same with exception of q≥3 points which are in the same plane, then the number of planes each containing three points is .pC3−.qC3.
Statement-2: 3 non-collinear points alwasy determine unique plane.
View SolutionThe centre of circle passing through three non collinear points A,B,C is the concurrent point of
View SolutionPlane|Equation of Plane in Normal Form#!#Equation of a plane perpendicular to a given vector and passing through a given point#!#Equation of a plane passing through three non collinear points#!#Intercept form of the equation of a plane#!#Examples
View SolutionStatement 1: there exists a unique sphere which passes through the three non-collinear points and which has the least radius. Statement 2: The centre of such a sphere lies on the plane determined by the given three points.
View SolutionStatement 1: there exists a unique sphere which passes through the three non-collinear points and which has the least radius. Statement 2: The centre of such a sphere lies on the plane determined by the given three points.
View SolutionI : Through three non-collinear points only one plane exists
II : Through three collinear points only one plane exists
Which of the following is correct?
View SolutionStatement-1: There are p≥8 points in space no four of which are in the same with exception of q≥3 points which are in the same plane, then the number of planes each containing three points is .pC3−.qC3.
Statement-2: 3 non-collinear points alwasy determine unique plane.
View Solution
