A rod of length 2 units whose one end is and other end touches the plane then a. the rod sweeps the figure whose volume is d. cubic units. e. the area of the region which the rod traces on the plane is i. j. the length of projection of the rod on the plane is q. units. r. the centre of the region which the rod traces on the plane is kk.Solution in Bengali
the rod sweeps the figure whose volume is cubic units.
the area of the region which the rod traces on the plane is .
the length of projection of the rod on the plane is units.
the centre of the region which the rod traces on the plane is
The correct Answer is:b
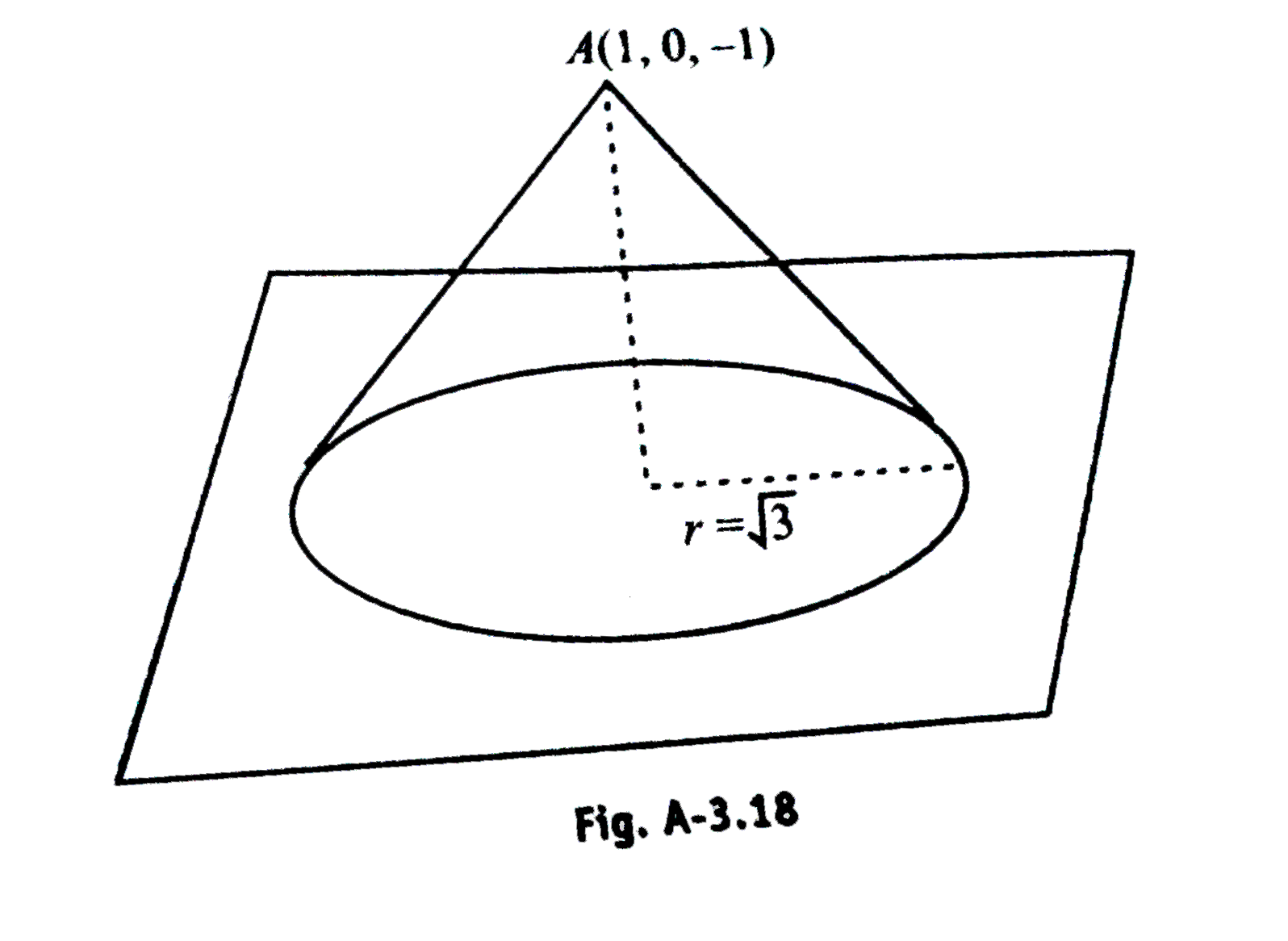
The rod sweeps out the figure which is a cone.
The distance of point from the plane is
unit
The slant height of the cone is 2 units.
Then the radius of the base of the cone is
Hence, the volume of the cone is
cubic units
Area of the circle on the plane which the rod traces is .
Also, the centre of the circle is . Then
or .
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - REASONING TYPE
10 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - LINKED COMPREHENSION TYPE
12 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - SINGLE CORRECT ANSWER TYPE
86 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions
291 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
Similar Questions
Statement 1: Lines →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj)and→r=4ˆi−ˆk+μ(2ˆi+3ˆk) intersect. Statement 2: →b×→d=0 , then lines →r=→a+λ→band→r=→c+λ→d do not intersect.
View SolutionGiven four points A(2,1,0),B(1,0,1),C(3,0,1)andD(0,0,2). Point D lies on a line L orthogonal to the plane determined by the points A, B and C.
The equation of the plane ABC is
View SolutionIn a three-dimensional xyz space , the equation x2−5x+6=0 represents a. Points b. planes c. curves d. pair of straight lines
View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
View SolutionIf the linear density (mass per unit length) of a rod of length 3m is proportional to x, where x, where x is the distance from one end of the rod, the distance of the centre of gravity of the rod from this end is.
View SolutionA rod of length 2 units whose one ends is (1,0,−1) and other end touches the plane x−2y+2z+4=0, then which statement is false
View SolutionThe thin rod shown below has mases M and length L. A force F acts at one end as shown and the rod is free to rotate about the other end in horizontal plane. Initial angular acceleration of the rod is :
View SolutionA rod of length 2units whose one end is (1,0,−1) and other end touches the plane x−2y+2z+4=0, then
View SolutionM द्रव्यमान तथा L लंबाई की एक छड़ को क्षैतिज तल में उसके एक सिरे पर ऊर्ध्व अक्ष के प्रति कीलकित किया गया है एक क्षैतिज बल F=Mg2 कीलकित सिरे से 5L6 दूरी पर छड़ पर आरोपित किया जाता है । छड़ का कोणीय त्वरण कितना होगा ?
View SolutionA particle is attached to the lower end of a uniform rod which is hinged at its other end as shown in the figure. The minimum speed given to the particle so that the rod performs circular motion in a vertical plane will be ___________ (in m/s)
[length of the rod is l=5m consider masses of both rod and particle to be same, g=10m/s2]

View SolutionA rod of length k slides in a vertical plane, its ends touching the coordinate axes. Prove that the locus of the foot of the perpendicular from the origin to the rod is (x2+y2)3=k2x2y2.
View SolutionA rod of length 2 units whose one ends is (1,0,−1) and other end touches the plane x−2y+2z+4=0, then which statement is false
View SolutionA rod of length k slides in a vertical plane, its ends touching the coordinate axes. Prove that the locus of the foot of the perpendicular from the origin to the rod is (x2+y2)3=k2x2y2.
View Solution2 इकाई लम्बाई की एक छड़ जिसका एक अंतिम सिरा (1,0,-1) तथा दूसरा सिरा एक तल x−2y+2z+4=0 को स्पर्श करता है, तो
View SolutionA rod of length 2 units whose one end is (1,0,−1) and other end touches the plane x−2y+2z+4=0, then
View Solution
