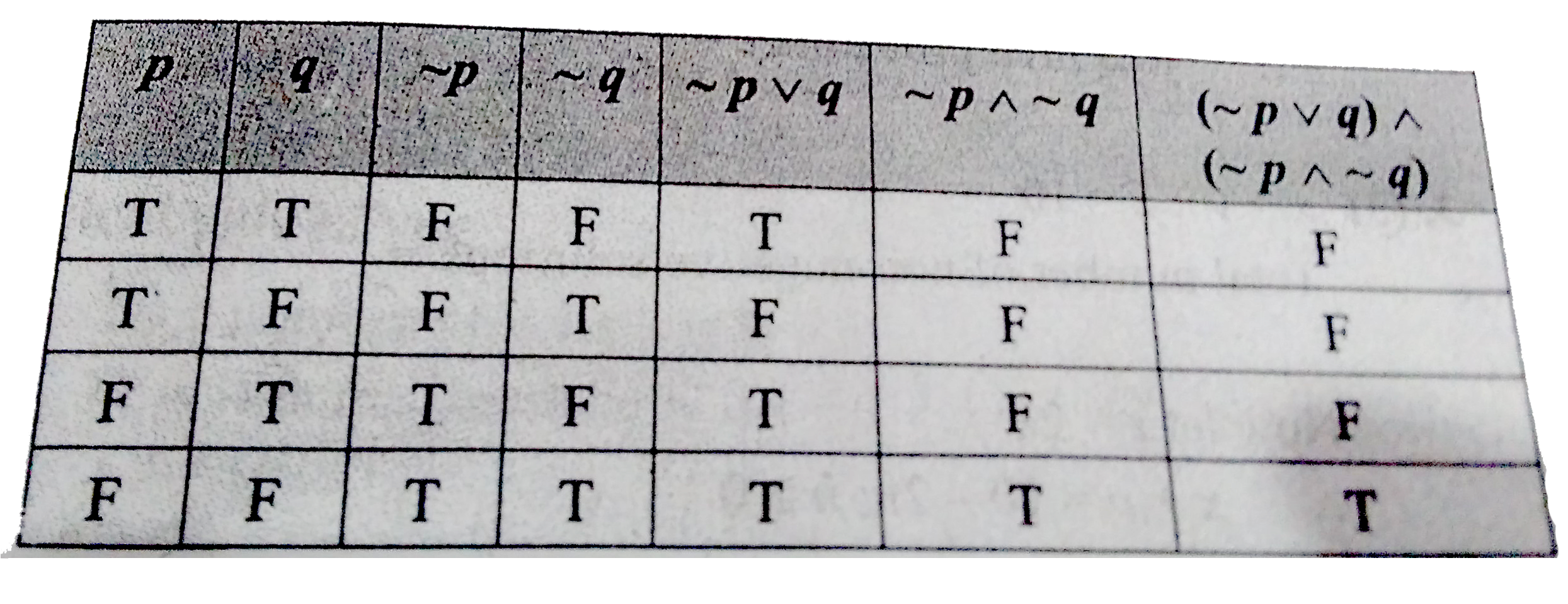
Write down the truth table for the compound statements :
(~p∨q)∧(~p∧~q) Solution in Bengali
Topper's Solved these Questions
- MATHMETICAL REASONINGBook:CENGAGE PUBLICATIONChapter:MATHMETICAL REASONINGExercise:Single correct answer type
Explore 38 Videos
- MATHMETICAL REASONINGBook:CENGAGE PUBLICATIONChapter:MATHMETICAL REASONINGExercise:Archives
Explore 10 Videos
- MATHMETICAL REASONINGBook:CENGAGE PUBLICATIONChapter:MATHMETICAL REASONINGExercise:Archives
Explore 10 Videos
- LOGARITHM AND ITS PROPERTIESBook:CENGAGE PUBLICATIONChapter:LOGARITHM AND ITS PROPERTIESExercise:JEE ADVANCED
Explore 1 Video
- MATRICESBook:CENGAGE PUBLICATIONChapter:MATRICESExercise:All Questions
Explore 509 Videos
Similar Questions
Which of the following is not a statement.
View SolutionStatements -1 : ~(p↔~q) is equivalent to p↔q
Statement-2: ~(p↔~q) is a tautology.
View SolutionStatements -1 : ~(p↔~q) is equivalent to p↔q
Statement-2: ~(p↔~q) is a tautology.
View SolutionLet (x0,y0) be the solution of the following equations: (2x)1n2=(3y)1n3 31nx=21ny The x0 is 16 (b) 13 (c) 12 (d) 6
View SolutionIn which of the following type of matrix inverse does not exist always? a. idempotent b. orthogonal c. involuntary d. none of these
View SolutionWrite down the truth table for the compound statements :
(~p∨q)∧(~p∧~q)
View SolutionConstruct the truth table for the followings statements :
(a) (p∧q)→~p (b)(p∧q)→(p∨q)
(c) (p∧q)→r (d)[p∧(~r)]→(q∨r)
View SolutionWrite down the truth table of ~p∧q
View SolutionWrite down the truth table for the statement
(~p∨q)∧(~p∧~q)
View SolutionLet p and q be two component statement of the compound statement p∨q . If the truth value of p∨q be " F" then the truth value of p and q are respectively -
View Solutionमिश्रित कथन ~(p∧q) के लिए सत्यमान सारणी बनाये
View SolutionProve by Truth Table: ~(p∨q)=~p∧~q.
View SolutionConstruct the truth table for (p∨q)∨~q
View SolutionWrite down the truth table for the compound statements :
(~p∨q)∧(~p∧~q)
View SolutionConstruct the truth table for the followings statements :
(a) (p∧q)→~p (b)(p∧q)→(p∨q)
(c) (p∧q)→r (d)[p∧(~r)]→(q∨r)
View Solution