At what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.Solution in Kannada
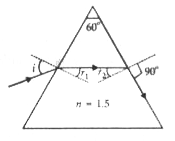
Critical angle :ic=sin−1(1n)
ic=sin−1(11.5)=sin−1(0.6667)
=41∘49.
Angle of incidence onn the second face:
r2=41∘49.
Angle of refraction at the first face:
r1A−r2
=60∘−41∘40.=18∘11
Applying Snell.s law to the first face:
n=sinisinr1
[OR using n1 sin i =n2 sin r1,n1=1,n2=1.5]
⇒ sin i=n sin r1
⇒ sin i=1.5 in 18∘11
1.5(0.3121)=0.4682
ANgle of incidence on the first face:
i=sin1(0.482)=27∘55.
(Award full marks for the answwers rounded off to 2 significant figures :1/1.5=0.67 , ic=42∘,i=28∘)
Detailed Answer:
Refractive index =μ =1.5
Critical angke =θC
∴sinθC=1μ
or siθC=23
∴θC=42∘
∴∠ADP=90∘−42∘=48∘
∠BAC=60∘
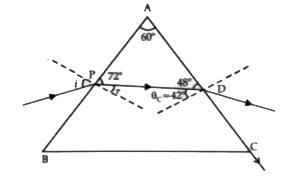
∴∠APD=180∘−60∘−48∘=72∘
∴∠r=90∘−72∘=18∘
sinisinr=μ
Or, sinisin18∘=1.5
OR, sin i=1.5×sin18∘
OR, sini=1.5×0.31
Or,sini=0.465
∴i=27.7∘
The angl of incidence should be 27.7∘
Topper's Solved these Questions
- KARNATAKA II PUC APRIL 2020 CLASS -XIIBOOK - OSWAAL PUBLICATIONCHAPTER - KARNATAKA II PUC APRIL 2020 CLASS -XIIEXERCISE - PART-D (Answer any two of the following questions:)
6 Videos
- II PUC MARCH-2018BOOK - OSWAAL PUBLICATIONCHAPTER - II PUC MARCH-2018EXERCISE - PART-D
11 Videos
- MAGNETISM & MATTER BOOK - OSWAAL PUBLICATIONCHAPTER - MAGNETISM & MATTER EXERCISE - TOPIC-2 Magnetism ( Short Answer Type questions-II)
3 Videos
Similar Questions
Derive σ=ne2τm
where the symbols have their usual meaning.
View SolutionGive an expression for the electric potential at a point due to a point charge.
View SolutionWrite any two differences between diamagnetic and paramaganetic substances.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) , so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524 .
View SolutionA ray of monochromatic light is incident on one refracting face of a prism of angle 75^(@) . It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the material of the prism is sqrt2 , the angle of incidence on the first face of the prism is
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View SolutionA ray of light is incident on a glass prism of refractive index mu and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face ? The refractive index of the material of the prism is 1.524.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refractive angle 60^(@) so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524 .
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face ? The refractive index of the material of the prism is 1.524
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60^(@) so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
View SolutionAt what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face ? The refractive index of the material of the prism is 1.524.
View SolutionA ray of light is incident on a glass prism of refractive index μ and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
View SolutionA ray of monochormatic light is incident on one refracting face of a prism of angle 75∘. It passes through the prism an is incident on the other at the critical angle. If the refractive index of the material of the prism is √2, the angle of the first face of the prism is
View Solution
