Question

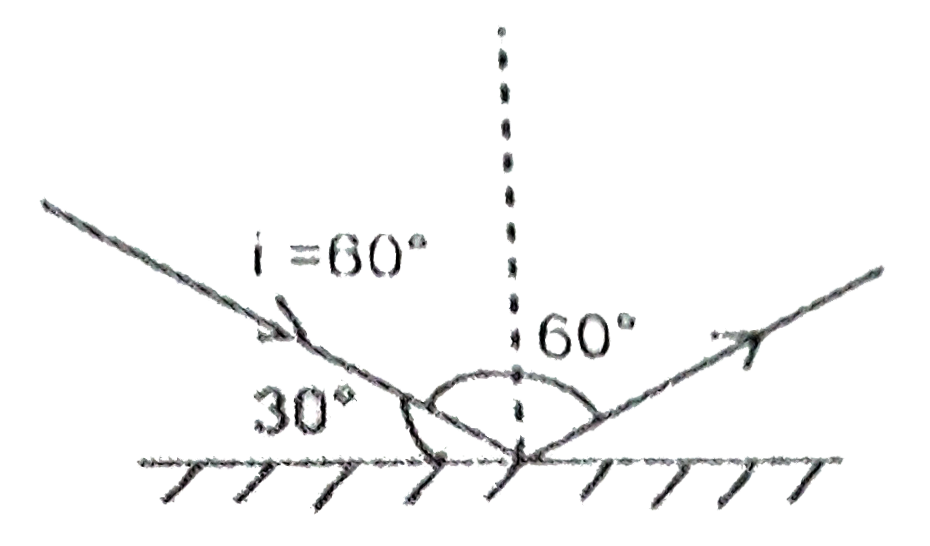
If a ray of light incident on an opaque surface makes an angle of ${30}^{\circ }$ with the mirror, what will be the angle of reflection of its corresponding reflected ray?

Explanation

To solve the problem, we need to understand the relationship between the angle of incidence and the angle of reflection when light interacts with a surface. Here’s the step-by-step solution:

Step 1: Understand the given information
We know that a ray of light is incident on an opaque surface at an angle of 30 degrees with respect to the surface.

Step 2: Identify the normal line
The normal line is an imaginary line that is perpendicular to the surface at the point of incidence. Since the angle of incidence is measured from the normal, we need to find the angle between the incident ray and the normal.

Step 3: Calculate the angle of incidence
Since the angle between the incident ray and the surface is given as 30 degrees, we can find the angle of incidence (i) using the relationship:
- Angle of incidence (i) = 90 degrees - Angle with surface
- i = 90 degrees - 30 degrees = 60 degrees

Step 4: Apply the law of reflection
According to the law of reflection, the angle of incidence is equal to the angle of reflection (r). Therefore:
- Angle of reflection (r) = Angle of incidence (i)
- r = 60 degrees

Step 5: Conclusion
The angle of reflection of the corresponding reflected ray is 60 degrees.

Final Answer:
The angle of reflection of its corresponding reflected ray is 60 degrees.

---