A football player kicks a ball at an angle of with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, Solution in Gujrati
(a) Time taken by the ball to reach the highest point
s
(b) The maximum height attained
m
(c) The horizontal range
m
(d) The time of flight
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-69videos
KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-289videos
KINEMATICS
- BOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question1videos
MISCELLANEOUS
Similar Questions
A particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
06:14View SolutionA student performs an experiment to determine how the range of a ball depends on the velocity with which it is projected. The "range" is the distance between the points where the ball lends and from where it was projected, assuming it lands at the same height from which it was projected. It each trial, the student uses the same baseball, and launches it at the same angle. Table shows the experimental results.
∣∣ ∣ ∣ ∣ ∣ ∣∣TrailLaunch speed(m/s)Range(m)110822031.833070.7440122.5∣∣ ∣ ∣ ∣ ∣ ∣∣
Based on this data, the student then hypothesizes that the range, R, depends on the initial speed v0 according to the following equation : R=Cvn0, where C is a constant and n is another constant.
Based on this data, the best guess for the value of n is :-04:43View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
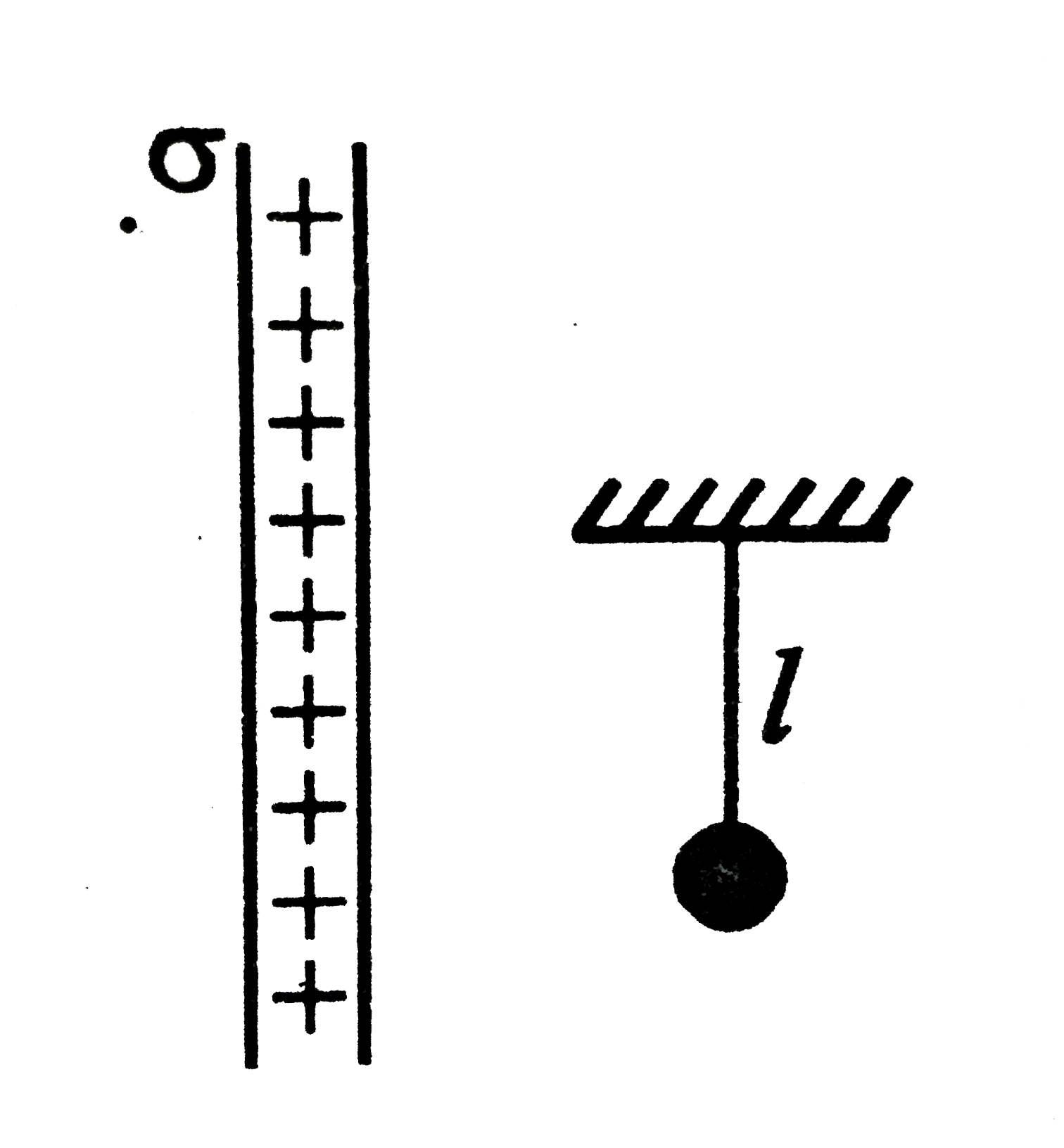 .05:35View Solution
.05:35View SolutionA ball is thrown at a speed of 40 m/s at an angle of 600 with the horizontal. Find a. the maximum height reached and b. the range of te ball. Take g=10ms2.
10:14View SolutionA batsman hits a ball at an angle of 306∘ with an initial speed of 30ms−1. Assuming that the ball travels in a verticle plane, calculate.
(a) The time at which the ball reaches the highest point.
(b) The maximum height reached.
( c) The horizontal range of the ball.
(d) The time for which the ball is in the air.03:07View SolutionA football player kicks a ball at ball at an angle of 30 ^(0) with the horizontal with an initial speed of 20 m//s . Assuming that the ball travels in a vertical plance, calculate (a) the time at which the ball reaches the highest point (b) maximum height reached (c ) the horizontal range of the ball (d) the time for which the ball is in air. g =10 m//s^(2) .
03:24View SolutionA football player kicks a ball at an angle of 37^(@) to the horizontal with an initial speed of 15 ms^(-1) . Assuming that the ball travels in a vertical plane, calculate (i) the time at which the ball reaches the highest point (ii) the maximum height reached (iii) the horizontal range of the projectile and (iv) the time for which the reached (iii) the horizontal range of the projectile and (iv) the time for which the ball is in air.
02:04View Solutionएक गेंद 15 मीटर/सेकण्ड के वेग से क्षैतिज से 30∘ का कोण बनाते हुए फेकि जाती है | ज्ञात कीजिए : (i) गेंद की उड़ान का समय तथा (ii) गेंद द्वारा प्राप्त अधिकतम ऊँचाई | (g = 10 मीटर/सेकण्ड2)
01:26View Solutionएक फुटबॉल के क्षैतिज से 45∘ का कोण बनाते हुए 20 m/s के वेग से किक किया जाता है । (a) मैदान पर पुनः पहुँचने में गेंद को कितना समय लगेगा ? (b) गेंद द्वारा प्राप्त अधिकतम ऊँचाई निकले । (c) किक करने के स्थान से कितनी दूरी गिरेगी ? g=10m/s2 माने।
04:35View SolutionA ball is thrown at a speed of 20 m/s at an angle of 30∘ with the horizontal . The maximum height reached by the ball is
(Use g=10m/s2)02:30View SolutionA cricket player can throw a ball with an initial speed of 30 m/s. What is the maximum range of the player can throw the ball? Neglect air reistance. [Take g =10m/s2]
02:31View SolutionA juggler bounces 5 balls in one hand in such a way that at any moment 4 balls are in the air and 1 ball stays in the hand. If the ball reaches a height of 20, the ball will have a stoppage time (g = 10 m / s).
02:57View Solutionएक खिलाड़ी फ़ुटबाल को 20 मीटर/सेकंड के प्रारंभिक वेग से क्षैतिज से 30∘कोण पर मारता है | निम्न की गणना कीजिए -
फ़ुटबाल द्वारा प्राप्य अधिकतम ऊँचाईView SolutionA ball is thrown at a speed of 40 m/s at an angle of 600 with the horizontal. Find a. the maximum height reached and b. the range of te ball. Take g=10ms2.
02:14View SolutionA football player kicks a ball at an angle of 37∘ to the horizontal with an initial speed of 15ms−1. Assuming that the ball travels in a vertical plane, calculate the maximum height reached.
03:35View SolutionA ball is thrown at a speed of 20 m/s at an angle of 30∘ with the horizontal. The maximum height reached by the ball is (use g=10m/s2)
03:35View Solution
