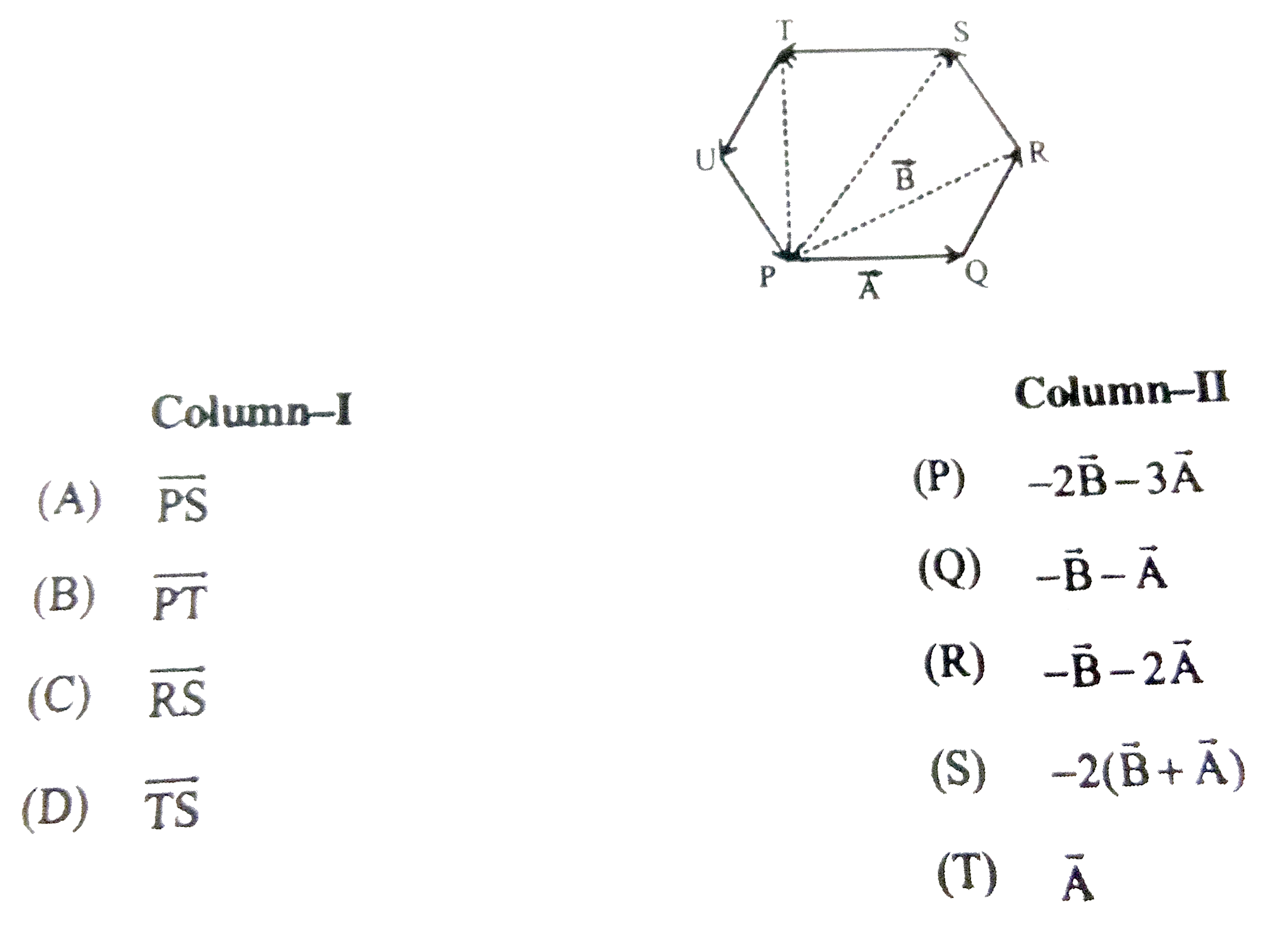
In a regular hexagon two −−→PQ=→A,−−→RP=→B. Express other vectors's in term of them :-
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:(A)-(S);(B)-(P);(C)-(R);(D)-(T)
Step by step video & image solution for In a regular hexagon two vec(PQ)=vecA, vec(RP)=vecB. Express other vectors's in term of them :- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-2) Single Correct Type Questions.17videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-2) Multiple Correct Type Questions.7videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) ParagraphType Questions.2videos
UNIT & DIMENSIONS, BASIC MATHS AND VECTOR
- BOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - MATHS1videos
TEST PAPERS
- BOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Previous Year Questions)7videos
WAVE OPTICS
Similar Questions
In a certain system of units, 1 unit of time is 5 sec, 1 unit of mass is 20 kg and 1 uint of length is 10m. In this system, one unit of power will correspond to :-
02:40View SolutionFour forces acting on a particle keep it in equilibrium, then :-
05:53View SolutionFor any particle moving with some velocity (→v) & acceleration (→a), tangential acceleration & normal acceleration are defined as follows.
Tangential acceleration - The component of acceleration in the direction of velocity.
Normal acceleration - The component of acceleration in the direction perpendicular to velocity.
If at a given instant, velocity & acceleration of a particle are given by .
→c=4ˆi+3ˆj
vaca=10ˆi+15ˆj+20ˆk
Find the tangential acceleration of the particle at the given instant :-01:40View SolutionIf complex number z(z≠2) satisfies the equation z2=4z+|z|2+16|z|3, then what is the value of |z|4 ?
02:05View SolutionElectromagnetic waves are transverse is nature is evident by
06:51View SolutionWrite →PQ+→RP+→QR in the simplified form.
03:25View SolutionIf →aand→b are the vectors determined by two adjacent sides of a regular hexagon ABCDEF, find the vector determined by the ther sides taken in order. Also find −−→ADand−−→CE in terms of →aand→b.
View SolutionIn trapezium PQRS, given that QR∣∣PSand2QR=PS.If−−→PQ=→a,−−→QR=→band−−→RS=→c, express →q in terms →band→c
03:02View SolutionWhat is the property of two vectores vec A and vec B if: (A) |vecA + vecB|= |vecA - vecB| (b) vecA + vecB = vecA - vecB
View SolutionIn a regular hexagon two −−→PQ=→A,−−→RP=→B. Express other vectors's in term of them :-
 View Solution
View SolutionLet △PQR be a triangle. Let →a=−−→QR,→b=−−→RPand→c=−−→PQ. if |→a|=12,∣∣→b∣∣=4√3and→b,→c=24 , then which of the following is ( are ) true ?
01:10View SolutionLet the vectors −−→PQ,−−→QR,−−→RS,−→ST,−−→TU and −−→UP represent the sides of a regular hexagon.
Statement 1: −−→PQ×(−−→RS+−→ST)≠→0
Statement 2: −−→PQ×−−→RS=→0 and −−→PQ×−→ST≠→002:58View SolutionIf →a,→b,→c are unit vectors such that →a+→b+→c=→o, then the value of →a⋅→b+→b⋅→c+→c⋅→a is -
04:44View SolutionIf →a⋅→b=→b⋅→c=→c⋅→a=0, then the value of [→a,→b,→c] is :
06:10View SolutionIf [→a,→b,→c]=1, then the value of →a⋅(→b×→c)(→c×→a)⋅→b+→b⋅(→c×→a)(→a×→b)⋅→c+→c⋅(→a×→b)(→c×→b)⋅→a is
04:03View SolutionIf [→a+→b,→b+→c,→c+→a]=8 then [→a,→b,→c] is
09:30View Solution

