A square wire of each side l carries a current I. What is the magnetic field at the midpoint of the square ?
4√2μo4πlI
8√2μo4πlI
16√2μo4πlI
32√2μo4πlI
The correct Answer is:B
B=B1+B2+B3+B4 B=4[μ04π.la(sinθ1+sinθ2)]
B=4×μ04πl(l/2)[1√2+1√2]
Here, a=l2,θ1=θ2=45∘
∴ B=16√2[μ04πll]=8√2(μ04πll)
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISM BOOK - PHYSICS WALLAHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - NEET PAST 5 YEARS QUESTIONS
12 Videos
- MOVING CHARGES AND MAGNETISM BOOK - PHYSICS WALLAHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - NEET PAST 5 YEARS QUESTIONS
12 Videos
- MOTION IN A STRAIGHT LINEBOOK - PHYSICS WALLAHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - NEET Past 5 Years Questions
5 Videos
- OSCILLATIONS BOOK - PHYSICS WALLAHCHAPTER - OSCILLATIONS EXERCISE - NEET PAST 5 YEARS QUESTIONS
5 Videos
Similar Questions
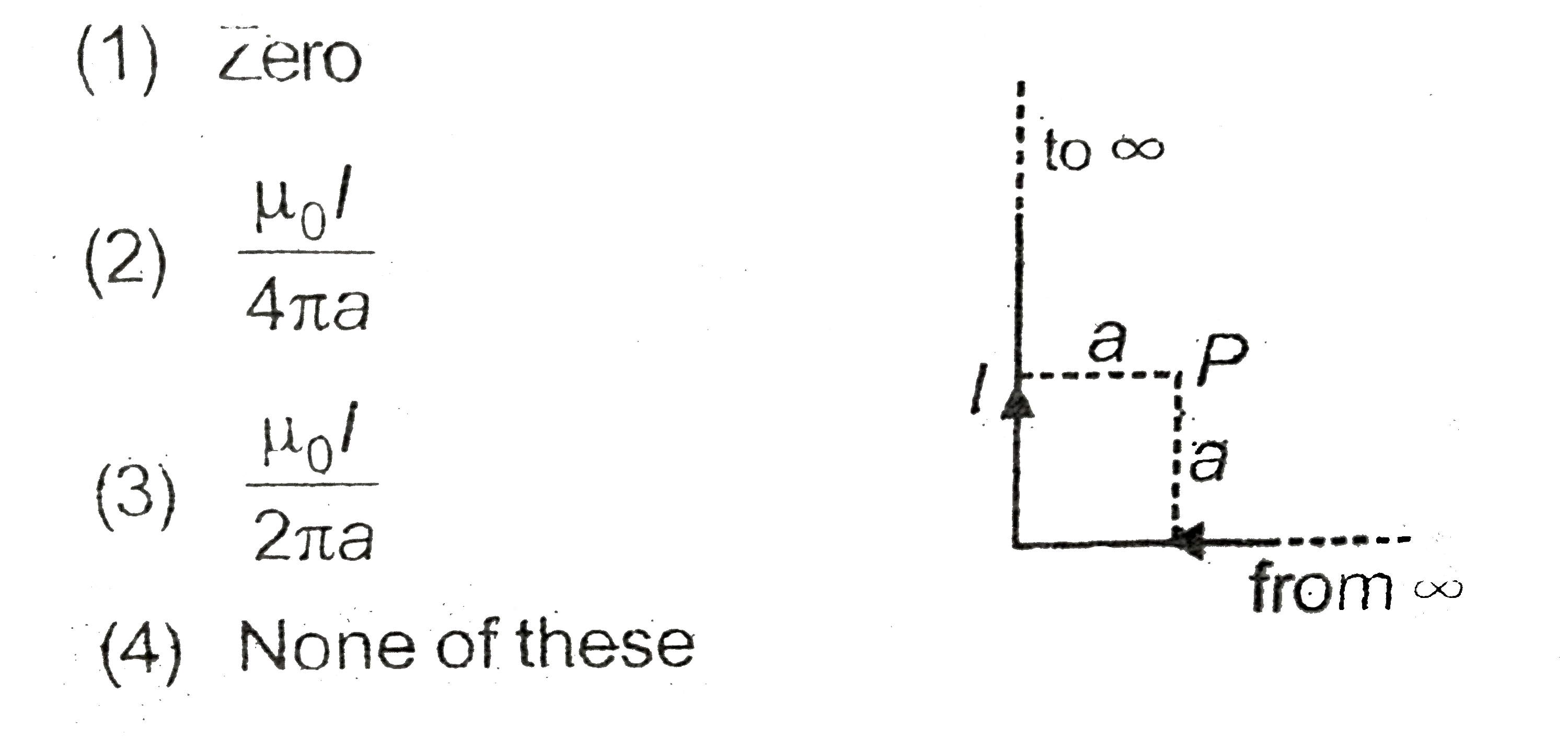
A wire of infinite length bent as shown in figure carries current l. What is the magnetic field at the point P ?
View SolutionA square loop of side length L carries a current which produces a magnetic field B0 at the centre (O) of the loop. Now the square loop is folded into two parts with one half being perpendicular to the other (see fig). Calculate the magnitude of magnetic field at the centre O.

View Solution
Knowledge Check
The magnetic field at the centre of square of side a is
 A√2μ0πaB√2μ0I3πaC23μ0IaDzero
A√2μ0πaB√2μ0I3πaC23μ0IaDzeroA square loop of side a carries a current I. The magnetic field at the center of the loop is
A2μ0I√2πaBμ0I√2πaC4μ0I√2πaDμ0IπaA wire in the from of a square of side 'a' carries a current i. Then the magnetic induction at the centre of the square wire is (Magnetic permeability of free space=μ0)
Aμ0i2πaBμ0i√2πaC2√2μ0iπaDμ0i√2πa
A wire in the form of a square of side '2m' carries a current 2A. Then the magentic induction at the centre of the square wire is ( magnetic permeability of free space =μ0)
View SolutionA square loop of edge 'a' carries a current l. The magnetic field at the centre of loop is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionAn equilateral triangular loop of wire of side / carries a current I. The magnetic field produced at the circumcentre of the loop is
View SolutionA square frame of side 1 m carries a current I, produces a magnetic field B at its centre. The same current is passed through a circular coil having the same perimeter as the square. The magnetic field at the centre of the circular coil is B'. The ratio B/B is
View Solution
