The area of the quadrilateral formed by the tangents at the endpoint of the latuc rectum to the ellipse x29+y025=1 is 27/4 sq. unit (b) 9 sq. units 27/2 sq. unit (d) 27 sq. unitSolution in Tamil
274
18
272
27
The correct Answer is:D
given equation of ellipse is
x29+y25=1
∴a2=9,b2=5⇒a=3,b=√5
Now, e=√1+b2a2=√1−59=23
FOci =(±ae,0)=(±2,0)andb2a=53
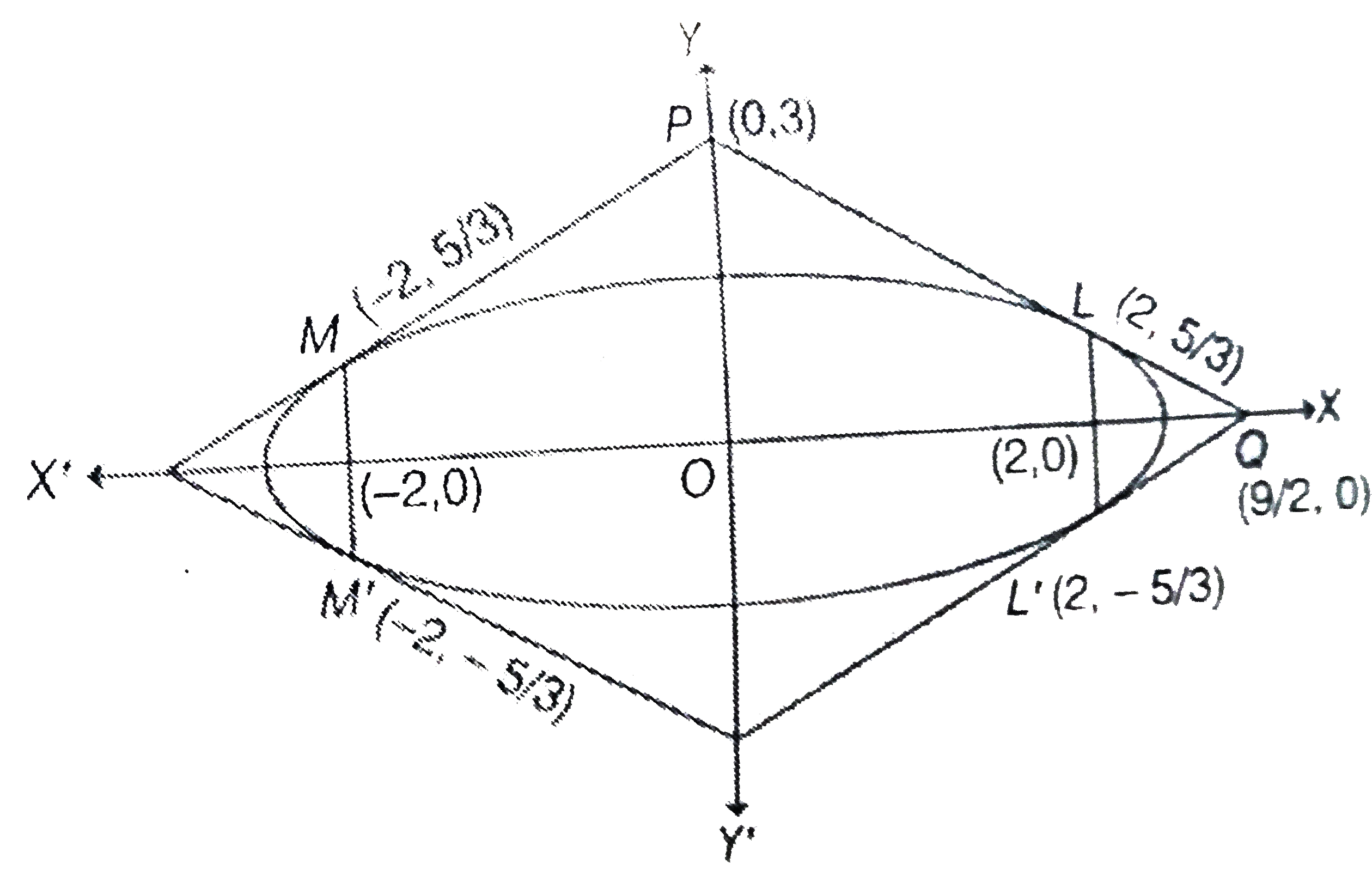
∴ Extremities of one of latusrectum are
(2,53)and(2,−53)
∴ Equation of tangent at (a,53)is
x(2)9+y(5/3)5=1or2x+3y=9
since Eq. (ii) intersects X and Y - axes at (92,0) and (0,3) ., respectively .
∴ Area of qudarlateral =4×Area of ΔPOQ
=4×(12×92×3)=27sq units
Similar Questions
The area bounded by the curves y=|x|−1andy=−|x|+1 is 1 sq. units (b) 2 sq. units 2√2 sq. units (d) 4 sq. units
View SolutionThe area (in sq. units) of the quadrilateral formed by the tangents at the end points of the latus rectum to the ellipse x29+y25=1 is (a) 274 (b) 18 (c) 272 (d) 27
View SolutionThe area of the triangle formed by the line x=3, y=4 and x=y is (a)12sq.unit (b)1sq.unit (c)2sq.unit (d) None of these
View SolutionThe area between the curve y=1−|x| and the x axis is equal to a)1 sq unit b)12 sq unit c)13 sq unit d)2 sq unit
View SolutionThe area of the region bounded by the curves y=|x−1| and y=3−|x| is (A) 6 sq. units (B) 2 sq. units (C) 3 sq. units (D) 4 sq. units
View SolutionIf one side of a rhombus has endpoints (4, 5) and (1, 1), then the maximum area of the rhombus is 50 sq. units (b) 25 sq. units 30 sq. units (d) 20 sq. units
View SolutionThe area of the quadrilateral formed by the tangents at the endpoint of the latus rectum to the ellipse (x^2)/9+(y^2)/5=1 is (A) 27/4 sq. unit (B) 9 sq. units (C) 27/2 sq. unit (D) 27 sq. unit
View SolutionThe area bounded by the curves y=|x|-1a n dy=-|x|+1 is 1 sq. units (b) 2 sq. units 2sqrt(2) sq. units (d) 4 sq. units
View Solution
