Suppose a parabola y=x2−ax−1 intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of ΔABC intersects the y-axis again at the point D(0,t). Then the value of t is Solution in Tamil
1/2
1
3/2
2
The correct Answer is:B
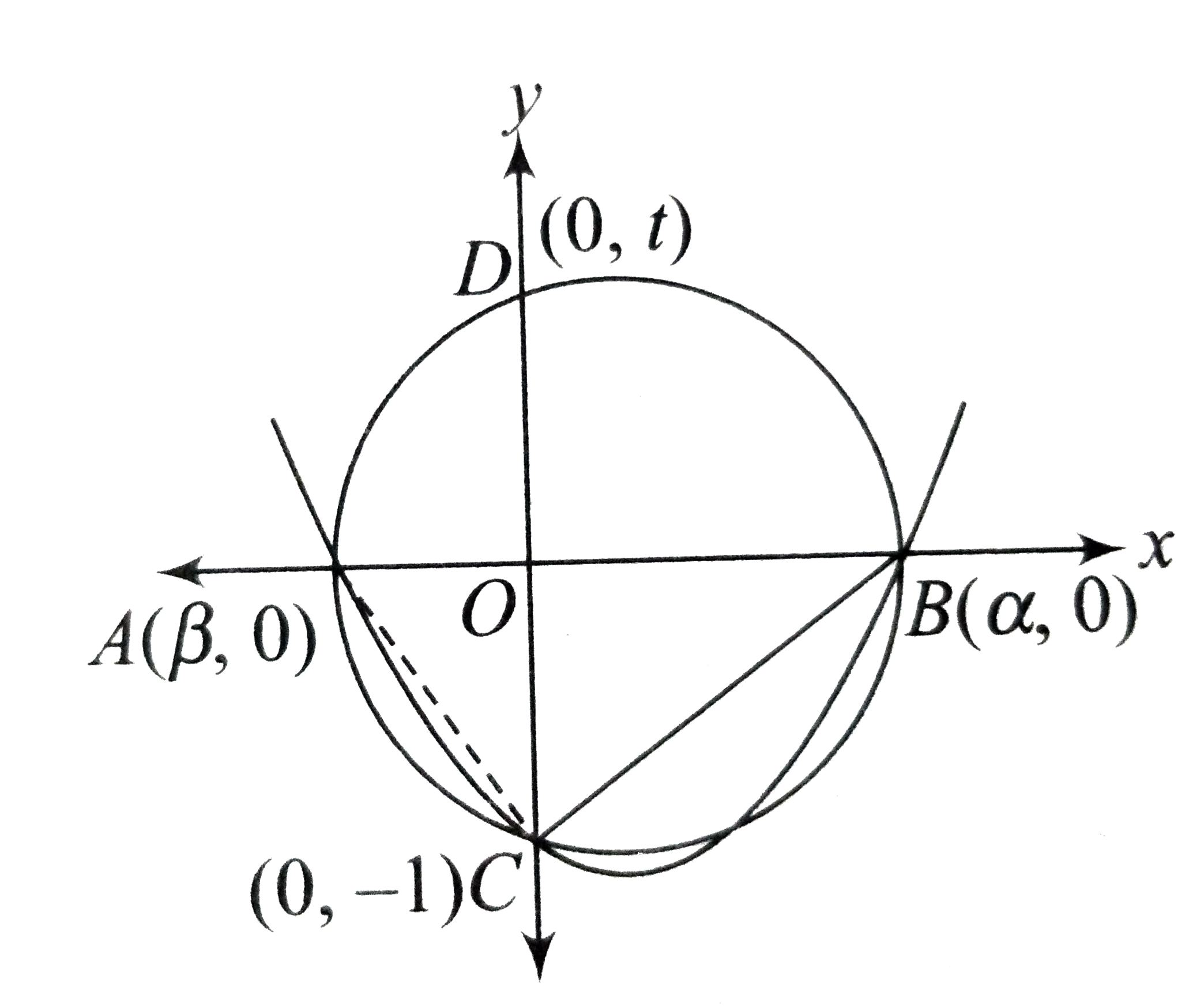
We have x=a±√a2+42
∴α=a+√a2+42,β=a−√a2+42
Equation of family of circles through A and B is
(x−α)(x−β)+y2+λy=0
As it passes through C(0,−1) we have
αβ+1−λ=0 (But αβ=−1)
⇒−1+1−λ=0
⇒λ=0
∴ Equation of circle through A,B and C is
(x−α)(x−β)+y2=0
It cuts the y-axis when x=0, so
αβ+y2=0
y2=1 (Put αβ=−1)
⇒y=1 or −1
Hence t=1
Topper's Solved these Questions
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Multiple Correct Answers Type
10 Videos
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Comprehension Type
1 Videos
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - JEE Advenced Single Answer Type
18 Videos
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Numerical)
5 Videos
- PERMUTATION AND COMBINATIONBOOK - CENGAGECHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Question Bank
4 Videos
Similar Questions
A family of curve S is given by S≡x2+2xy+y2−4x(1−λ)−4y(1+λ)+4, then S=0 represents
View SolutionA parabola is drawn through two given points A(1,0) and B(−1,0) such that its directrix always touches the circle x²+y2=4. Then, The locus of focus of the parabola is=
View Solutionabout to only mathematics
View SolutionThe distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
View SolutionIf the number of ways in which a selection of 100 balls can be made out of 100 identical red balls, 100 identical blue balls and 100 identical white balls is
View SolutionSuppose that three points on the parabola y=x^(2) have the property that their normal lines intersect at a common point (a,b).The sum of their x-coordinates is
View SolutionAn ellipse with axes parallel to coordinate axes cuts the parabola y^(2)=4x at (1,-2) and touches itat (4,4) then the coordinate of other point of intersection is
View SolutionLine x−y=1 intersect the parabola y2=4x at A and B. Normals at A and B intersect at C. If D is the point at which line CD is normal to the parabola, then coordinate of point D is
View SolutionTangent to the parabola y=x2+ax+1 at the point of intersection of the y-axis also touches the circle x2+y2=r2. Also, no point of the parabola is below the x-axis.
The minimum area bounded by the tangent and the coordinate axes is
View SolutionSuppose a parabola y=x2−ax−1 intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of ΔABC intersects the y-axis again at the point D(0,t). Then the value of t is
View SolutionDraw the graphs of 2x + y = 6 and y = 2x + 2 intersecting X and Y axes in the points A, B and C, D respectively. The point of intersection is E. Find the area of (a) ΔAEC (b) ΔEDB
View Solutionमान लीजिये रेखा y = x परवलय y=ax2+c की स्पर्श रेखा है।
यदि (1, 1) प्रतिच्छेद बिंदु हो, तो a का मान होगा
View Solutionमान लीजिये रेखा y = x परवलय y=ax2+c की स्पर्श रेखा है।
यदि c=2 तब प्रतिच्छेद बिंदु होगा
View SolutionThe locus of point of intersection of lines xta−yb+t=0 and xat+yb=1t
View SolutionIf (xa)+(yb)=1 and (xc)+(yd)=1 intersect the axes at four concylic points and a2+c2=b2+d2, then these lines can intersect at, (a,b,c,d>0)
View Solution
