If S is the sum of cubes of possible value of c for which the area of the figure bounded by the curve y=8x2−x5, then straight lines x=1andx=c and the abscissa axis is equal to 163, then the value of [S],where[.] denotest the greatest integer function, is____Solution in Tamil
The correct Answer is:2
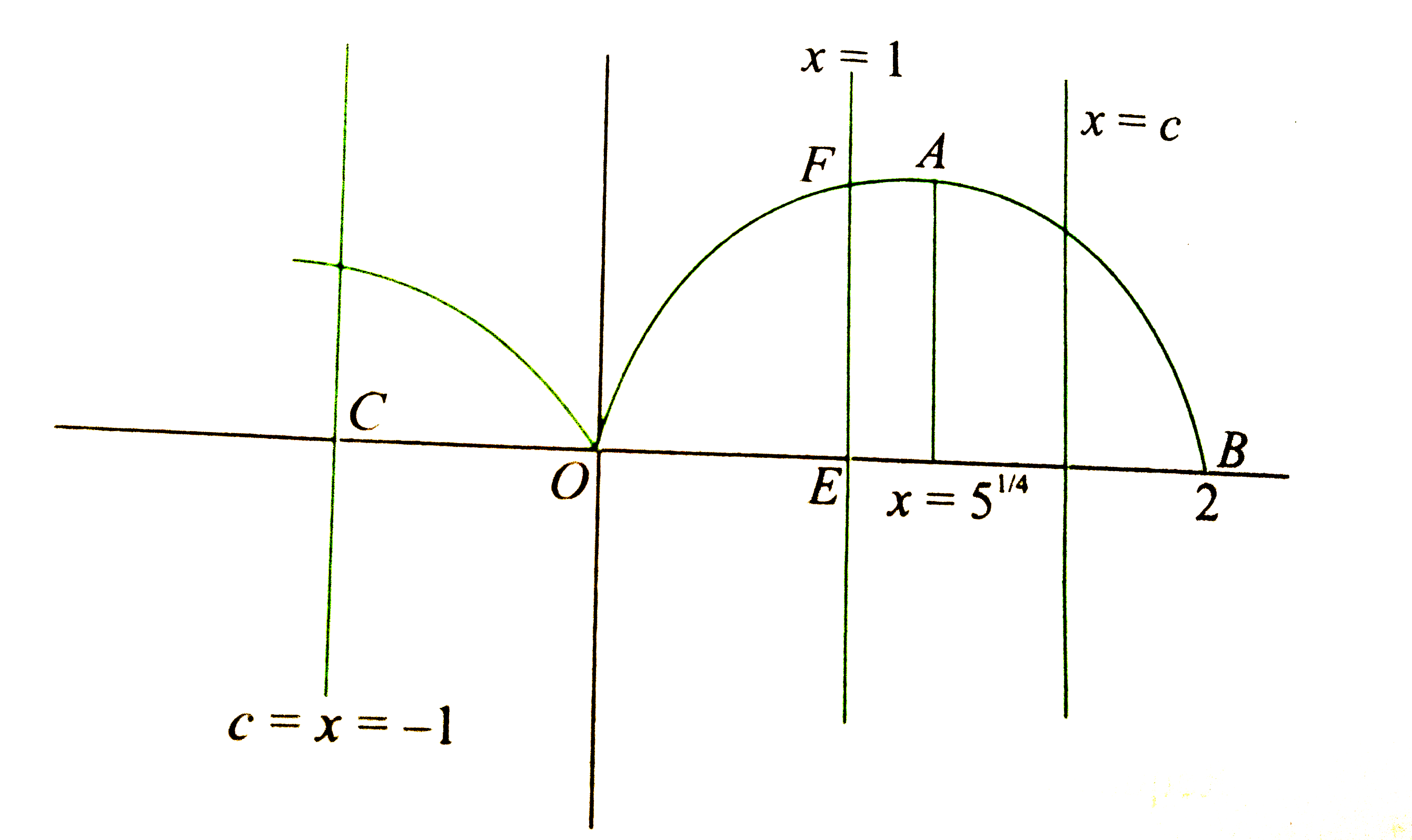
Given that c∫1ydx=163
⇒ c∫1(8x2−x5)dx=163c=(8−√17)1/3 (c>0)
Area OFE=c∫0(8x2−x5)dx=83 (C>0)
so c=−1
Hence, c=−1and(8−√17)1/3
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - JEE Main Previous Year
10 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - JEE Advanced Previous Year
9 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Matrix)
4 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
The area of the figure bounded by the parabola (y−2)2=x−1, the tangent to it at the point with the ordinate y=3, and the x-axis is
View SolutionFind the area bounded by the curves x+2|y|=1 and x=0.
View SolutionMatch the following lists :

View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionIf S is the sum of possible values of c for which the area of the figure bounded by the curves y=s∈2x, the straight lines x=π6,x=c, and the abscissa axis is equal to 12, then the value of π/S is____
View SolutionIf S is the sum of cubes of possible value of c for which the area of the figure bounded by the curve y=8x2−x5, then straight lines x=1andx=c and the abscissa axis is equal to 163, then the value of [S],where[.] denotest the greatest integer function, is____
View SolutionWhich of the following is the possible value/values of c for which the area of the figure bounded by the curves y=sin2x, the straight lines x=π/6,x=c and the abscissa axis is equal to 1/2?
View SolutionFind the value of ‘c’ for which the area of the figure bounded by the curve, y=8x2–x5, the straight lines x = 1 and x = c and the abscissa axis is equal to 16/3.
View SolutionColumn I, Column II Area enclosed , q. 1. sq. units The smaller area included between the curves √x+√|y|=1 and |x|+|y|=1 , r. 4 sq. units Area bounded by the curves y=[x264+2](where[.]de¬es the greatest integer function), y=x−1andx=0 above the x-axis., s. 2/3 sq. units
View SolutionWhich of the following abnormalities is due to X-linked recessive mutation ?
View SolutionIf S is the sum of cubes of possible value of c for which the area of the figure bounded by the curve y=8x2−x5, then straight lines x=1andx=c and the abscissa axis is equal to 163, then the value of [S],where[.] denotest the greatest integer function, is____
View SolutionThe integral ∫1.50[x2]dx,where[.] denotoes the greatest integer function, equals ...........
View SolutionEvaluate: ∫1000(x−[x]dx(where[.] represents the greatest integer function).
View SolutionWhich of the following is the possible value/values of c for which the area of the figure bounded by the curves y=sin2x, the straight lines x=π/6,x=c and the abscissa axis is equal to 1/2?
View Solution
