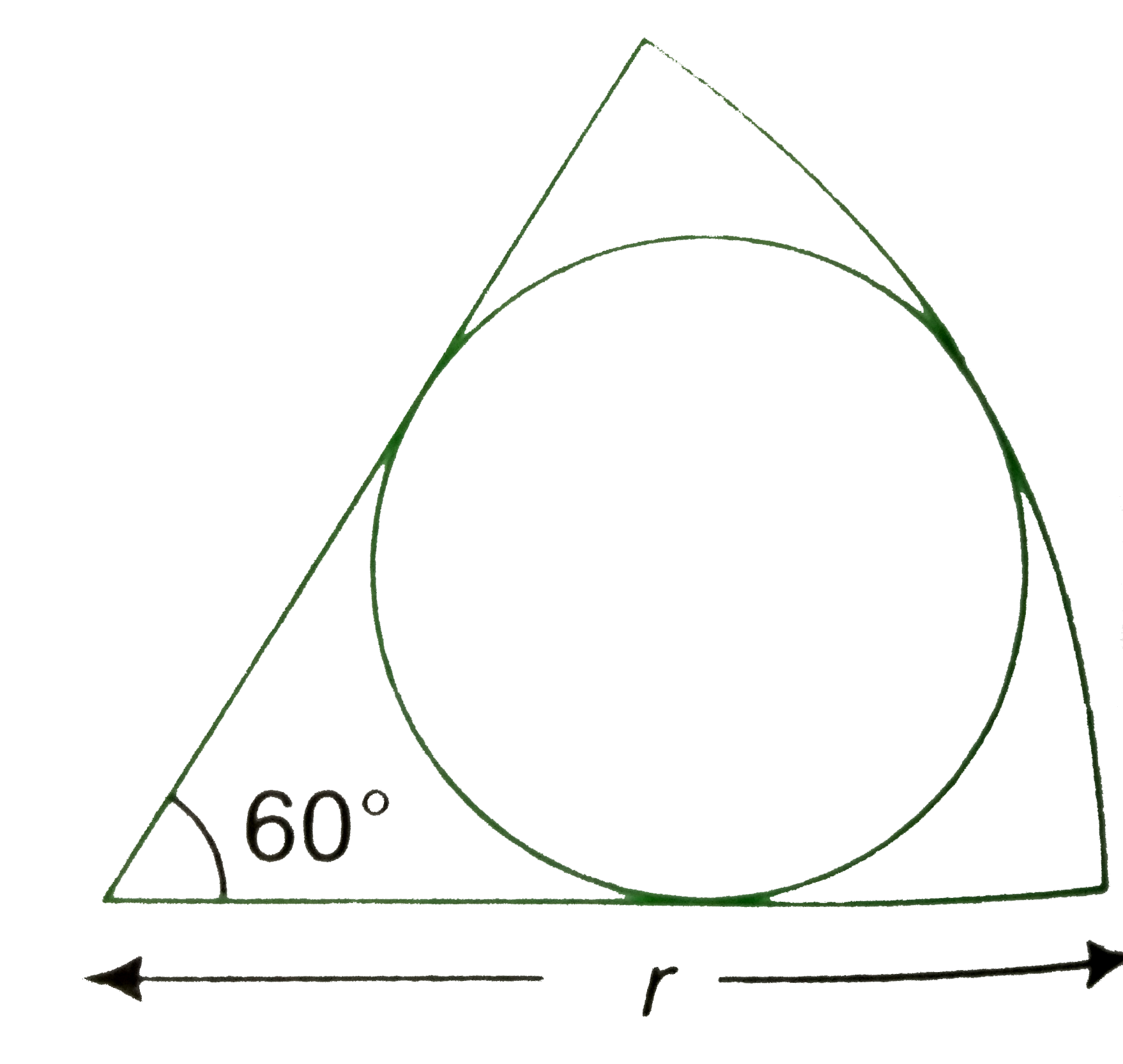
A circle is drawn in a sector of a larger circle of radius r, as shown in figure. The smaller circle is tangent to the two bounding radii and the arc of the sector. The radius of the smaller circle is r2 (b) r3 (c) 2√3r5 (d) r√2Solution in Tamil
r2
r3
2√3r5
r√2
The correct Answer is:A
Let the radius of smaller circle be x. Here,
OP=xcosec30∘
andOQ=r=xcosec30∘
⇒=x=r3

Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Multiple)
17 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Comprehension)
11 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.6
9 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Videos
Similar Questions
If 0≤θ≤πand81sin2θ+81cos2θ=30 is
View SolutionLet us consider the equation cos4xa+sin4xb=1a+b,x∈[0,π2],a,b>0
The value of sin2x in terms of a and b is
View SolutionProve that: tan7200−cos2700−s∈1500cos1200=14
View SolutionFind the derivative of y=tan−12x3
View SolutionRefer to the following diagram :

Column IColumn IIa.Collinear vectorsp.→ab.Coinitial vectorsq.→bc.Equal vectorsr.→cd.Unlike vectors (same initial point)s.→d
View SolutionThree equal circles each of radius r touch one another. The radius of the circle touching all the three given circles internally is (2+√3)r (b) (2+√3)√3r (2−√3)√3r (d) (2−√3)r
View SolutionWrite the area of the sector of a circle whose radius is r and length of the arc is l .
View SolutionA circle is drawn in a sector of a larger circle of radius r, as shown in the adjacent figure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the small circle is-
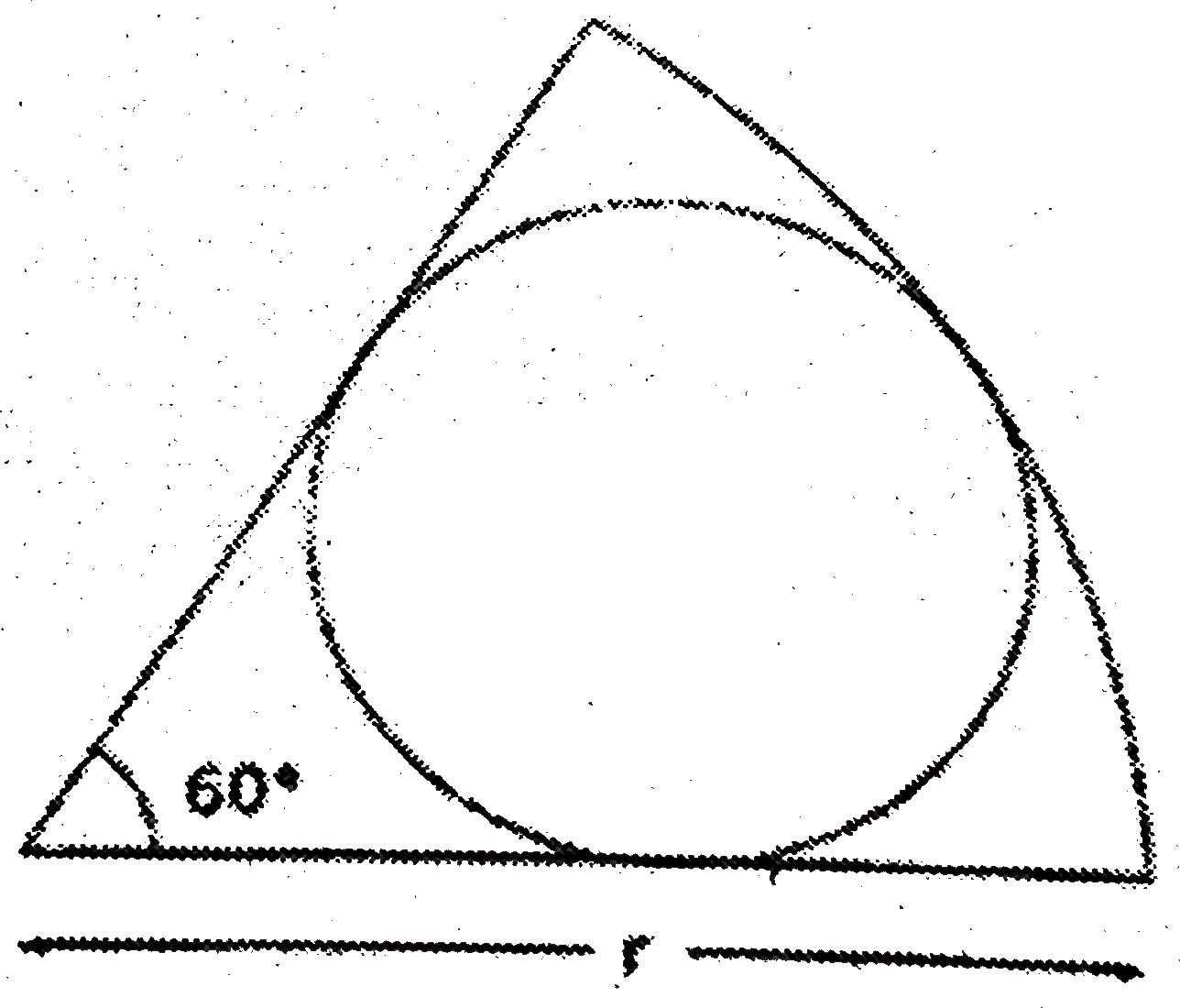
View SolutionA circle is drawn in a sectore of a larger circle of radius r, as shown in fugure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the smaller circle is
View SolutionThe length of an arc of a sector of angle θ∘ of a circle with radius R is
View SolutionLength of an arc of a sector of a circle of radius r and angle θ is
View SolutionProve that (1) the area of a sector of circle with radius r and central angle θ∘ is 12r2θ
(ii) the area of a sector of a circle of radius r and an arc of length s is 12rs
View SolutionA circle is drawn in a sector of a larger circle of radius r, as shown in the adjacent figure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the small circle is-

View SolutionThree equal circles each of radius r touch one another. The radius of the circle touching all the three given circles internally is (2+√3)r (b) (2+√3)√3r (2−√3)√3r (d) (2−√3)r
View SolutionA circle is drawn in a sectore of a larger circle of radius r, as shown in fugure. The smaller circle is tangent to the two bounding radii and the are of the sector. The radius of the smaller circle is

View SolutionWrite the area of the sector of a circle whose radius is r and length of the arc is l .
View Solution
